What is the most essential reason that actually leads to the quantization. I am reading the book on quantum mechanics by Griffiths. The quanta in the infinite potential well for e.g. arise due to the boundary conditions, and the quanta in harmonic oscillator arise due to the commutation relations of the ladder operators, which give energy eigenvalues differing by a multiple of $\hbar$. But what actually is the reason for the discreteness in quantum theory? Which postulate is responsible for that. I tried going backwards, but for me it somehow seems to come magically out of the mathematics.
-
3$\begingroup$ The other postulate is that it arises in the dynamics of little tiny strings, which is perfectly consistent with all observations, but such things are still viewed as speculative. $\endgroup$– FreedomCommented Oct 6, 2012 at 15:38
-
$\begingroup$ In reviewing some of the response and your replies, I am curious as to what you are actually after in the the question "But what actually is the reason for discreteness in quantum theory?" The current high scoring answers are tautological since they rely largely on the enforcement of boundary conditions of some sort. So its not entirely clear what the goal of the question is. I was wondering if you could clarify. $\endgroup$– FreedomCommented Oct 7, 2012 at 15:06
-
$\begingroup$ @HalSwyers: I want an intutive (if possible, in not mathematical) explanation for how discreteness in the theory of quantum mechanics actually arises. So, what feature of QM makes it discrete rather than the continuous solutions in classical mechanics. $\endgroup$– user7757Commented Oct 7, 2012 at 16:04
-
$\begingroup$ @ ramanujan_dirac well the answer there is simply that we define a fundamental unit of action \hbar. This partitions the respective Hilbert/phase space (depending on use). This is, at some level, an arbitrary feature, however, experiment proves that this is how nature operates. As with most things in physics, the proof is usually not mathematical but empirical. At some level, the universe is the ultimate black box. We can ask it well formulated questions, and it will give an answer, but its inner workings are still too complex for use to determine. $\endgroup$– FreedomCommented Oct 7, 2012 at 17:21
6 Answers
If I'm only allowed to use one single word to give an oversimplified intuitive reason for the discreteness in quantum mechanics, I would choose the word 'compactness'. Examples:
The finite number of states in a compact region of phase space. See e.g. this & this Phys.SE posts.
The discrete spectrum for Lie algebra generators of a compact Lie group, e.g. angular momentum operators. See also this Phys.SE post.
On the other hand, the position space $\mathbb{R}^3$ in elementary non-relativistic quantum mechanics is not compact, in agreement that we in principle can find the point particle in any continuous position $\vec{r}\in\mathbb{R}^3$. See also this Phys.SE post.
-
2$\begingroup$ I absolutely agree that the most fundamental reason is the symplectic noncommutative structure which quantizes phase space areas and limits information (in the sense of dimensionality of a complex vectors space) to phase space area. But I'm not sure if this is the whole story if you talk about observed discreteness like in a double slit experiment or atomic spectroscopy. $\endgroup$– A.O.TellCommented Oct 6, 2012 at 14:11
-
$\begingroup$ Awesome answer. I am absolutely delighted! $\endgroup$– user7757Commented Oct 6, 2012 at 17:16
-
$\begingroup$ Excellent answer! You simultaneously explained discreteness from boundary conditions on Schrodinger's Eqn and from the compact Lie Rotation group! Your item (3) would not be an exception if the 3 spatial translation generators were non-commuting generators of a compact group. Perhaps translations become obviously non-commuting at cosmological distances? $\endgroup$ Commented Apr 9, 2020 at 19:55
-
-
$\begingroup$ @user76284 Exactly! The commutator of affine connections (which really are the 4x4 matrix translation generators for 4-vectors) is the Riemann tensor ( $[P_i,P_j]_{kl}=R_{ijkl}=\frac{\Lambda}{3}(g_{il}g_{kj}-g_{jl}g_{ki})$ for de Sitter ). The cosmological constant $\Lambda=\frac{1}{length^2}$ really is a new fundamental constant (ie: not a weird dark energy density) that sets the size of the commutator of translations, just like $\frac{1}{c^2}$ sets the size of the commutator of velocity boosts. $\endgroup$ Commented Sep 25, 2020 at 23:43
There are several forms of discreteness in quantum theory. The simplest one is the discreteness of eigenvalues and the associated countable eigenstates. Those arise similarly to the discrete standing waves on a guitar string. The boundary conditions only allow certain standing waves that nicely fit into the enforced region in space. Even though the string is a continuous object, its spectrum becomes discontinuous and is naturally labeled with natural numbers. Exactly the same thing happens in unbounded (from above) quantum potentials like the infinite well or the harmonic oscillator, where you also get discrete standing quantum waves. (Other potentials can generate both discrete and continuous eigenvalues at the same time)
Another reason for discreteness comes in with multi-particle systems. Quantum theory requires that a system that is realized in space-time contains a unitary representation of the symmetry group of space-time, the lorentz group. In fact, you can define a particle in quantum theory as a subsystem that contains such a group representation. And because you can't have any non integer fraction of a unitary group representation, you need to have an integer number of them in your total system. So the number of particles is also an (expected) discrete feature, and it plays a role when you talk about single photons for example, that are either absorbed completely or not at all.
And finally there is a form of discreteness that comes with quantum measurement. The measurement postulate says that the result of a measurement is an eigenvalue of an hermitian operator called an observable. Now the existence of discrete spectra for these operators is related to my first point (boundary conditions), but this one goes deeper. While the existence of a discrete spectrum of the energies of a system still allows all continuous energy values by superposition, the measurement outcome results in exactly one (often discrete) result. This is responsible for the discreteness of the beams in the Stern-Gerlach experiment for example. Why quantum measurement works this way is essentially an open question even today. There are some approaches to answer it, but there is no generally accepted answer that would explain all aspects convincingly.
-
$\begingroup$ Thanks for the answer. Firstly I would like to know which postulate/property of quantum mechanics entails a unitary representation of the symmetry group (is it because of unitarity - the fact that the sum of the probabilities should be 1?) Secondly, I didnt understand your statement: he measurement outcome results in exactly one (often discrete) result. What do you mean by a discrete result, when we say that QM is discrete we mean that the possible outcomes can take only specific values, but how can you talk about the discreteness of a specific result. $\endgroup$– user7757Commented Oct 6, 2012 at 13:21
-
$\begingroup$ (contd) Do you mean to say that there is no accepted explanation for the discrete spectrum of the eigenvalues? And therefore, the foundations of QM are basically empirical? Help will be much appreciated on this matters. $\endgroup$– user7757Commented Oct 6, 2012 at 13:22
-
$\begingroup$ What I meant is that the outcome is picked from a discrete set. Some measurements allow outcomes from a continuous set, like a position measurement for example. $\endgroup$– A.O.TellCommented Oct 6, 2012 at 13:22
-
$\begingroup$ No, the discrete spectrum of eigenvalues is mathematically well understood. What is not understood is why a measurement forces a system to be in one of the eigenstates associated with the measured eigenvalue after the measurement. This is essentially known as the "quantum measurement problem". You can surely find a lot of information about it if you look for it. $\endgroup$– A.O.TellCommented Oct 6, 2012 at 13:25
-
$\begingroup$ Regarding the unitary representations of symmetry, that issue has mostly been raised by Weyl and Wigner who studied the representation of groups in quantum theory. I'm afraid I cannot explain the details in a comment, but the basic idea is that physically observable symmetries can be described as groups, and the representations of these groups must be contained in the describing mathematics. And it turns out that for quantum theory the only option is a unitary representation. $\endgroup$– A.O.TellCommented Oct 6, 2012 at 13:28
If you want you can go back to Planck's derivation of the black body energy spectrum, otherwise known as Planck's law, as well as Einstein's use of Planck's work in his explanation of the Photo Electric Effect (which garnered him the Nobel prize) in order to first understand some of the experimental motivation. However, to understand the roots of quantum mechanics in atomic physics, one must go back to Bohr and Rutherford model of hydrogen. An Introduction to Quantum Physics by French and Taylor discusses the Bohr-Rutherford model of the hydrogen atom on page 24. This model was introduced around 1913 and Bohr provided two key postulates:
An atom has a number of possible "stationary states." In any one of these states the electrons perform orbital motions according to Newtonian mechanics, but (contrary to the predictions of classical electromagnetism) do not radiate so long as they remain in fixed orbits.
When the atom passes from one stationary state to another, corresponding to a change in orbit (a "quantum jump") by one of the electrons in the atom, radiation is emitted in the form of a photon. The photon energy is just the energy difference between the initial and final states of the atom. The classical frequency $\nu$ is related to this energy through the Planck-Einstein relation:
$$E_{photon} = E_i - E_f = h\nu$$
Which was described in Bohr's paper On the Constitution of Atoms and Molecules. These postulates are slightly dated in modern conceptions of electron motion, since we now understand things better in terms of the Schrodinger equation, which allows for an an extremely accurate model of the hydrogen atom. However, one of the key concepts Bohr introduced is the Correspondence Principle, which according to French and Taylor:
...requires classical and quantum predictions to agree in the limit of large quantum numbers...
This is a key ingredient in modern physics, and is best understood in terms of asymptotic analysis. Most modern theories connect to real observed phenomena at the large N limit of the theory.
Admittedly these are the practical origins of why we have quantum mechanics, as far as the reason nature chose these things, the answer might be very anthropic. We simple wouldn't exist without them. Dirac frequently pondered the question why and here was his answer in 1963:
It seems to be one of the fundamental features of nature that fundamental physical laws are described in terms of a mathematical theory of great beauty and power, needing quite a high standard of mathematics for one to understand it. You may wonder: Why is nature constructed along these lines? One can only answer that our present knowledge seems to show that nature is so constructed. We simply have to accept it. One could perhaps describe the situation by saying that God is a mathematician of a very high order, and He used very advanced mathematics in constructing the universe.
Despite several modern attempts to attack the more meta-physical aspects of this, and give them rigor, there is still no really good answer...as Feynman or Mermin said:
Shut up and calculate!
-
2$\begingroup$ I hope you realize that when Mermin said "Shut up and calculate!" he did not intend this as advice. In his own words, he was "dismissing an interpretive position of others by lampooning it as a 'shut up and calculate interpretation'." $\endgroup$ Commented Oct 7, 2012 at 0:58
-
$\begingroup$ @PeterShor Absolutely agree! The point that I failed to convey but was trying to make is that this question of why nature uses stationary states. We have ample experimental proof, and some proofs of stationary states arising in certain contexts but as far as a reason why this should occur in nature I suspect is unanswerable. I find the other responses to this question to be tautological. String theory is on the right track since at least it keeps this very fundamental. $\endgroup$– FreedomCommented Oct 7, 2012 at 15:01
-
$\begingroup$ You are saying that this question is not answer able by reason. You have to depend on experimental truth. Right? @HalSwyers $\endgroup$ Commented Jun 18, 2014 at 11:24
In a more mathematical sense, the discreteness just arises out of the mathematics. For example: The Schrodinger equation is a classic Sturm-Liouville problem in ODE. https://en.wikipedia.org/wiki/Sturm–Liouville_theory
That means we get eigenfunctions (our eigenstates in QM) and eigenvalues corresponding to those eigenfunctions (our energy levels). The Hamiltonian operator in the Schrodinger equation would be our self adjoint SL operator.
A very interesting question, indeed !
In late 19th century Physics had ordinary crisis - classical physics at that time predicted that black body emitted radiation intensity must increase monotonically with increasing wave frequency. This can be seen from a graph (black curve, 5000K) :
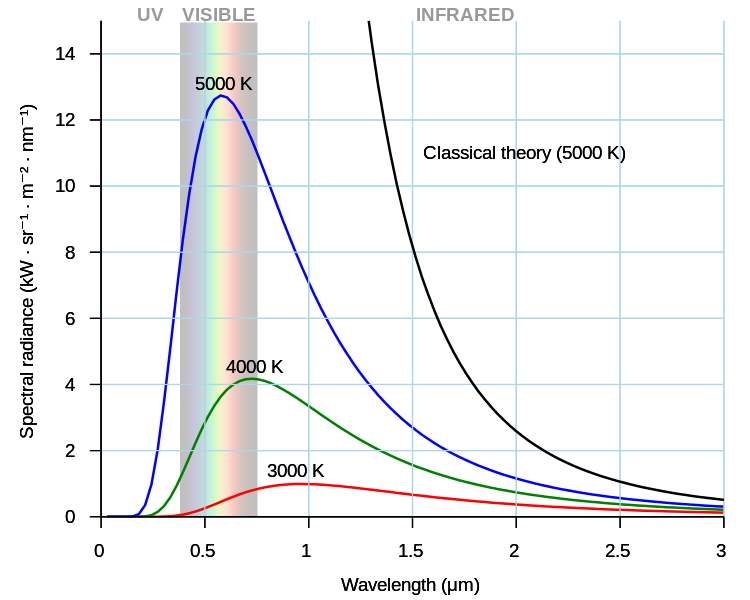
One, by summing all energies which black body radiates away from all frequencies can show that it must approach infinity. Thus black body would almost instantly radiate all it's energy away and cool-down to absolute zero. This is known as "Ultraviolet catastrophe". But in practice, it was not the case. Black body really radiated according to unknown law at that time (blue curve, 5000K).
In 1900 Max Plank using strange assumptions at that time, that energy is absorbed or emitted discreetly - by energy quanta ($E=h\nu$) - was able to derive correct intensity spectral distribution law and resolve Ultraviolet catastrophe :
$$ B_{\lambda }(\lambda ,T)={\frac {2hc^{2}}{\lambda ^{5}}}{\frac {1}{e^{hc/(\lambda k_{\mathrm {B} }T)}-1}} $$
Albert Einstein in 1905 once again patched Physics and showed that Plank's quanta is not just empty theoretical construct, but real physical particles, which now we call photons.
The discreteness of quantum mechanics, is evident from the experimental evidence. Any experiment, take for example the stern gerlach, Will yield probabilistic answers under identical experimental conditions. The Matrix structure of quantum mechanics allows us to calculate only probability amplitudes of processes to happen.
-
1$\begingroup$ I am asking for a theoretical reason. $\endgroup$– user7757Commented Oct 6, 2012 at 12:24
-
$\begingroup$ Operator algebra is responsible. The state is a vector in the Hilbert space. States can be decomposed into the eigenstates of Hermitian operators. And measurement happens with respect to the eigenmodes of suitable hermtian operators. $\endgroup$ Commented Oct 6, 2012 at 12:40
