Finding the units of a variable in the wave function

Question

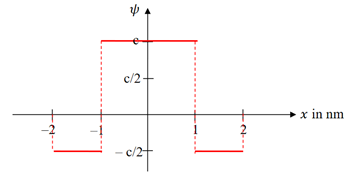
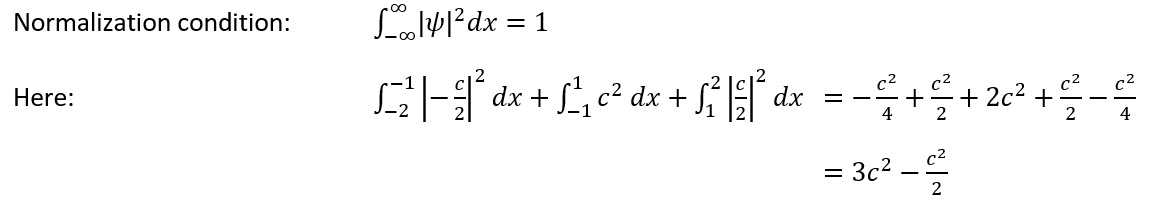I have the following figure which shows the wave function of an electron. The wave function is not realistic due to the discontinuities in slope, but consider its to approximate a possible smooth wave function.

I wondering what the units of $c$ is this question.

My attempt one:

Since:

The physical interpretation of the wavefunction is that $|\psi(\vec r)|^2dV$ gives the probability of finding the electron in a region of volume $dV$ around the position $\vec r$. Probability is a dimensionless quantity. Hence $|\psi(\vec r)|^2$ must have dimension of inverse volume and $\psi$ has dimension $L^{-3/2}$.

So in this case $\psi$ has units $L^{-1/2}$ this means c must have the same units.

However when is use the normalization condition to find the value of c i get the following equation:

which when solved gives me $c=\sqrt{\frac{2}{5}}$.

However, using the second last equation: $3c^2-\frac{c^2}{2}=\frac{5c^2}{2}=1$.

Since 1 is unitless, the LHS should also be unitless; however, since $c$ has the units $L^{-1/2}$ this would give LHS the units $1/L$

Answer 1

You forgot to include the units in your limits integration, $$1=\int_{-\infty}^{+\infty}dx\,\left|\psi(x)\right|^{2}= \int_{-2\,{\rm nm}}^{-1\,{\rm nm}}dx\,\left|\psi(x)\right|^{2}+ \int_{-1\,{\rm nm}}^{1\,{\rm nm}}dx\,\left|\psi(x)\right|^{2}+ \int_{1\,{\rm nm}}^{2\,{\rm nm}}dx\,\left|\psi(x)\right|^{2},$$ which gives, with your wave function, $$1=\frac{5}{2}c^{2}\,({\rm nm}).$$ Solving this for $c$ then naturally gives a $c$ with units of $({\rm nm})^{-1/2}$.