Does fire emit black-body radiation?

Question

Question: Can the radiation emitted by fire be approximated by black body spectrum?

It has been discussed in this community that black-body spectrum mostly serves as an approximation to actual spectra of thermal light sources:

• firstly because perceived radiation are not in thermal equilibrium (although this depends on how one defines BBR - as an equilibrium state of radiation or as a radiation emitted by a black body)
• secondly because the real sources of radiation are usually only approximately black bodies
• finally, the real sources of radiation are usually in a stationary state rather than in thermal equilibrium

Still, a slab of metal taken out of an oven, or even an oven itself could be treated as a black body heated to a constant temperature (which is also a strong assumption). Can we however say the same about a highly dynamic campfire?

Answer 1

Different bits of the fire have different characteristics, the spectrum of a flame would usually consist of discrete line radiation perhaps superposed on a weaker continuum. However, the base of the fire, especially say in the cavities between any burning material would emit radiation that more closely approximates the Planck function. In this respect, a just-lit fire would have light dominated by "flame" and the spectrum would not be Planckian, but a well-established hot fire with a lot of radiation coming from its "heart" would be more blackbody-like.

The flames that you see would usually be "optically thin" - that is they are transparent to their own and other radiation. In such circumstances, the flames emit light that usually correspond to discrete transitions at particular wavelengths - about as dissimilar to the Planck function as it could be. This is how "flame tests" for the presence of different chemical elements work. If the flame was hot enough to ionise atoms you would also get some recombination continuum radiation, but as I said, most flames are optically thin, so the radiation never gets the chance to come into equilibrium with the matter at some particular temperature and you would not get the Planck spectrum. If you can see through the flame, at any wavelength, then it isn't radiating a Planck spectrum.

On the other hand, the space between the coals of a hot fire can provide a reasonable simulacrum to the cavity radiation of an ideal blackbody. Here, we are looking into an enclosed space where the radiation field has had a chance to come into equilibrium with the surrounding material. This is still just an approximation - but a clue that you are looking at something close to the Planck spectrum comes when you start to lose the ability to discern the texture or shape of the material within the cavity. That is because everything is at a similar temperature and the radiation field is starting to approach isotropy.

The above discussion by the way is often why one will notice that it isn't the flames of a fire that give off the most heat, it is the base of the fire. That is because blackbody radiation is the most efficient way that a thermal radiator can lose heat (radiatively).

EDIT:

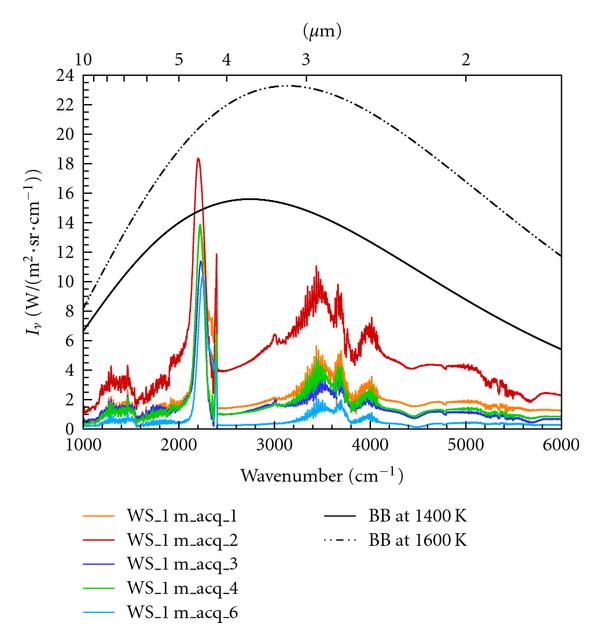
Here are some spectra taken of flames from a simulated wildfire, compared with blackbody spectra - not very Planckian (from Boulet et al. 2011). The main peak at 2300 cm$^{-1}$ is due to radiation from CO$_2$ and CO molecules. The conclusion in this paper is that the temperature is about 1500K and the CO$_2$/CO radiation is optically thick and thus reaches the Planck function, but that the flames are optically thin at all other wavelengths.

Answer 2

A good example of profoundly non-blackbody campfire features: the blue flame bases.

1. they are transparent (not really black in any sense)
2. they are blue. Their "color temperature" (the best-fit blackbody approximation of their spectrum) will be ~10 times the highest temperature one can find in a campfire.

Another (less visible, but pretty much important) non-blackbody part is the gas directly above the flames. It has pretty much linear spectrum (like gases do in general), but its characteristic lines are deep in the infrared.

On the other hand, there are at least two separate things in a campfire that look like a textbook black bodies:

1. The burning chars. They are black in the first place and they burn at 500-700C depending on the available oxygen.

2. The yellow parts of the flames. Here you get 800-1500C (depending on exactly where you look) blackbody radiation. The particular blackbodies in play here are the soot particles. They are pretty much black as well.

Note that by mixing two or more blackbody spectrums you will get a non-blackbody spectrum, unless their temperatures are equal or one of them pretty much dominates the final result.

p.s. obligatory XKCD: https://xkcd.com/1308/

Answer 3

The most accurate and at the same time most pedantic answer is that absolutely nothing emits exact black body radiation. It is always an approximation valid at a given temperature in a given frequency range.

In the case of such loosely defined physical systems as a 'fire' or a 'campfire' any statement about their spectrum is particularly loosely defined. In this handwaving sense a (camp)fire emits black body radiation. It does not exclusively emit black body radiation, but that was not the original question.

See here for a woodfire spectrum

The text states that the size of the CO2 peak is exaggerated for visibility. This is close enough to BBR for me.

Note that you are looking at hot glowing coal and hot gases at the same time.

Answer 4

I think the answer depends on what is meant by 'approximated', and on how well established the fire is. If we take the two key requirements for BBR, namely that the body should be unreflective and in thermal equilibrium, I suspect that parts of a campfire would satisfy both requirements reasonably well.

When wood burns it passes through a phase in which it is charred and black before becoming a white ash residue. During that phase it is probably a good absorber of visible radiation at least.

My guess is that the radiation from a fire can be crudely considered as having two main components. One will be the emission of radiation from the chemical reactions of combustion; the other will be radiation that is not from combustion itself but arises simply from the temperature to which the charred wood has been heated by the flames. I would expect the first component to be nothing like BBR, while the second would be like it.

I have a firebowl at home, and often study the different aspects of the appearance of the fire. When the fire is well-established, the incandescence from the hot logs at its heart seems to be quite different in nature from the light from the flames. It may be an optical illusion, but the impression is given that the glow is coming from some way inside the wood, and not just off its surface. I could readily imagine that the radiation from the gaps between the heated logs would be like that from a heated cavity of graphite.

Answer 5

It depends on the thickness of the fire.

Thin bodies/objects radiate less than the BB of the same $T$ - due to being "optically transparent".