Physical interpretation of increasing Reflectivity with increasing $\epsilon_i$ for small $\epsilon_r$

Question

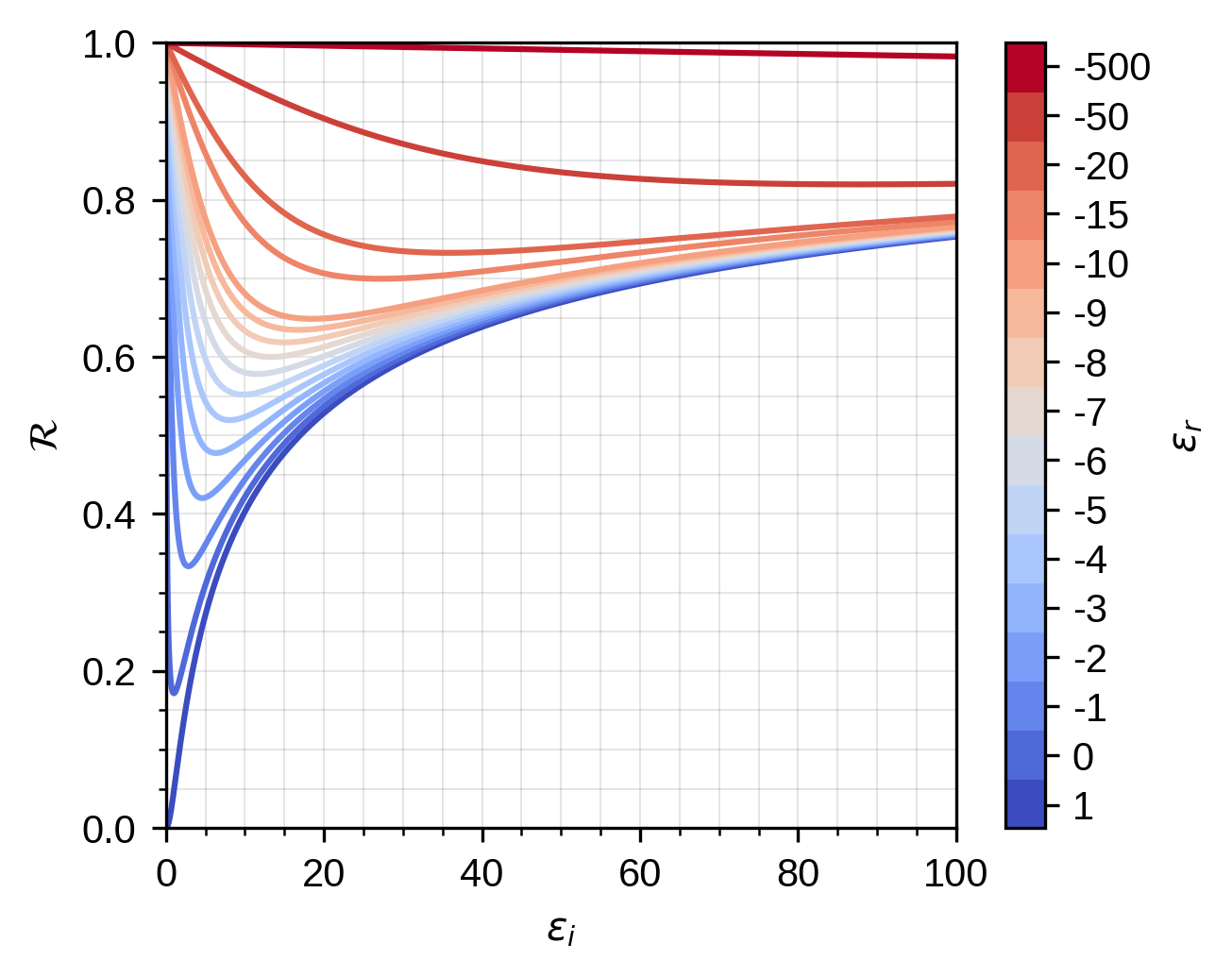
Currently I have plot Reflectivity, $R$ of a generic material (assuming a complex dielectric function, $\epsilon = \epsilon_r + i\epsilon_i$) as a function of $\epsilon_i$ for various $\epsilon_r$ values. These can be related together via: \begin{equation} R = \frac{(1-n)^2 + k^2}{(1+n)^2 + k^2} \end{equation} with $n + ik = \sqrt{\epsilon}$: \begin{equation} n = \frac{1}{\sqrt{2}}\sqrt{\sqrt{\epsilon_r^2 + \epsilon_i^2} + \epsilon_r} \end{equation} \begin{equation} k = \frac{1}{\sqrt{2}}\sqrt{\sqrt{\epsilon_r^2 + \epsilon_i^2} - \epsilon_r} \end{equation}

This plot is shown below:

It seems like for a more metallic material ($\epsilon_r$ << 0), increasing $\epsilon_i$ decreases the reflectivity, which I would understand as an increase in damping of free electron plasmon oscillations upon the surface of the metal in response to an incident EM field (ie light). However, I am struggling to understand the observed dependence for smaller values of $\epsilon_r$ where the system behaves more like a dielectric.

My initial guess is that increasing $\epsilon_i$ provides a dielectric with an increasing finite conduction value, meaning that the system can become polarised in response to an EM field, and thus reflect more light. Are there perhaps any literature references or papers that discuss this more? Is this line of thinking correct?

Thanks in advance.

Answer 1

This comes down to impedance mismatch. Remember, it is the impedance mismatch that ultimately determines the reflection: $$ r=\frac{E_r}{E_i}=\frac{\eta_2-\eta_1}{\eta_2+\eta_1}, $$ where $\eta=\sqrt{\mu/\epsilon}$ is the material impedance. Material 1 is the incident medium and Material 2 is the metal. When you notice that $\epsilon$ is in the denominator within a square root, you'll realize that both its real and imaginary parts will contribute to the real part of $\eta$.

Take your case of $\epsilon_r=1$. For $\epsilon_i=0$, then $\epsilon=\mu$ so $\eta=1$, matching the impedance of free space. So there's no reflection. Then, as $\epsilon_i$ increases, the medium diverges further and further from vacuum, and the impedance mismatch increases.

What actually happens when you introduce a non-zero $\epsilon_i$ to an otherwise lossless metal? Well, this introduces absorption, of course. But the absorption comes due to an admission of time-averaged power propagating into the metal itself. Think about it: If $\epsilon_i=0$, then $n$ is imaginary, and the wave inside the metal is purely evanescent. For $\epsilon_i \ne 0$, there is a net propagating wave inward (you get wiggles, not just exponential decay). This contributes to an impedance mismatch with vacuum.