Confusion on the coefficient of restitution

Question

I am struggling to grasp the usage of the coefficient of restitution $e$, to be more specific the conventions around $e$ taking negative values and its implications on oblique collisions.

As I have learnt, the coefficient of restitution $e$ is given by the following formula:

$$e=\frac{\text{Relative velocity of seperation}}{\text{Relative velocity of approach}}=\frac{\vert V_2-V_1 \vert}{\vert U_1-U_2 \vert}$$

However some confusion arises over the different manner of ways this formula is expressed in different textbooks, some use

$$e=\frac{ V_2-V_1 }{ U_1-U_2 }$$

or

$$e=\frac{ V_1-V_2 }{ U_1-U_2 }$$

.etc

However these formulae tend to give negative values of $e$ in some cases, and I am unsure on the proper convention regarding negative values of $e$ as I have been unable to find any mention of a negative value of $e$ across multiple textbooks.

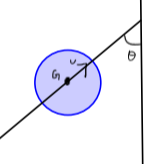
Furthermore another dilemma arises for me regarding using the coefficient of restitution for oblique collisions, say we have the following scenario where;

• The initial velocity of the ball is $u$
• The velocity immediately after collision is $v$
• The coefficient of restitution between the ball and wall is $e$
• At the instance of impact the ball makes an angle $\theta$ with the wall

Taking the upwards and right directions as positive and applying the formula for $e$ along the x direction I get

$$e=\frac{v_x}{u_x} \implies v_x=eu_x$$

However from the diagram it is apparent that the x component of the velocity immediately after collision will be towards the left direction, or in other words negative; The problem arises here, if $e$ can not take negative values, and we know that $u_x$ is positive, then how can $v_x$ be negative?

Answer 1

Use the coefficient of restitution from your first formula to find the magnitude of the velocity of separation (for example), and deal with positive $e$. It won't tell you the direction.

Just use common sense about the real situation to decide the directions.

Here is an example.

A ball A of mass 6kg is moving at 10m/s and collides with a stationary ball B of mass 4kg. The coefficient of restitution for the collision is 0.5

What is the velocity of each ball afterwards?

Taking positive to be the direction that the 6kg ball initially moves in

From your first formula the velocity of separation is 5m/s.

From conservation of momentum

momentum before = momentum after

$60 = 4V_B + 6(V_B-5)$

(we know the 6kg ball doesn't pass through the 4kg ball so we did $V_A = V_B - 5$ instead of $V_A = V_B+5$)

leading to $V_B = 9$ and $V_A = 4$