How is it that a particle's wave function is not a real thing, yet we can still observe it?

Question

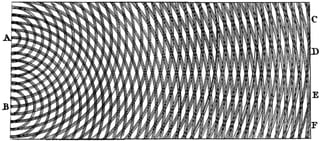
In the double slit experiment, scientists could see an interference pattern on the back panel. However, if the wave function is purely a mathematical object, how can it be the case that some physical manifestation of it can be measured and observed?

Answer 1

Plenty of things in physics are mathematical objects/models that we use to describe the world around us and can also use to guide our measurements and observations. For example, when you are walking around there isn't an actual velocity vector that suddenly forms at your location, yet we can use the mathematics of vectors to describe your motion, how the forces acting on you will change that motion, etc. You can't go and pick up an action integral, and yet much of physics relies on the minimization of such integrals.

The wave function is no different. It is a useful model that we can then use to describe and understand the world around us. It doesn't need to be physical (whatever that may mean) in order to describe the physical world; most (all?) of what we use to describe the physical world is not physical at all

Answer 2

The wave function is merely used to predict the statistical distribution of dots in the pattern you observe; it cannot predict the locations of each individual dot which make up the pattern.

The wave function is a rather complicated thing involving imaginary numbers as well as real ones and is more a model of possibilities than of probabilities. It needs some manipulation (such as squaring) to yield the probability distribution of dots in the pattern. We even say that probability = possibility squared. The "particle" which hits the screen is equally mathematical - we see only the result of its arrival, never the particle itself.

The statistical distribution of the dots is built up from the "collapse" (measurement) of a multitude of individual wave functions, each modelling a single photon. The probable positions of each dot are governed by the wave function, but the point of measurement on the detector, within the parameters of that distribution, is wholly random.

You have to remember that everything in theoretical physics, from Newton's F=ma to the latest entanglement formulae, is a mathematical model used to predict the outcomes of experiments. The more esoteric those equations become, the more the relation between the variables in those equations and the real world around us remains a matter of utter confusion.

Consequently, some people still cling to notions of real waves and real particles at some given location in space and time, perhaps the one guiding the other. But such "local realism" has been shown by entanglement experiments not to be the case at all. We are all arguing about which of locality, realism and/or temporal causality has to be sacrificed in order to reach a deeper understanding.

Answer 3

The wave function is not a purely mathematical object because, as you say, it has physical implications. However, the wave function is not observable directly (e.g. it's a complex function). One can also frame quantum mechanics in the Heisenberg language of matrix mechanics, without the need of wave functions, so the wave function by itself is not necessary to describe physical reality, even if it has physical implications (the Born rule for instance that connects probability of measurement with the norm of the wave function).

Answer 4

In the double slit experiment, scientists could see an interference pattern on the back panel.

You may find it easier if you see it this way:

• Light or broad EM radiation always originates from excited states of subatomic particles and is always emitted quantum by quantum.
• these quanta or photons are indivisible from the time of their emission from excited subatomic particles to their absorption
• Photons ( of low energy) do not interact with each other and are not subject to destructive interference.

The energy distribution (the fringes) are the result of deflections of the photons by their interaction with edges:

• Photons have an oscillating electric and an oscillating magnetic field component
• the surface electrons around the slit (or even a single edge) have static electric and magnetic field components and interact with each other (when externally excited, phonons and other excitons propagate through the material)
• the interaction of the two previous points causes the quantised deflection of the photons, which results in the fringes

However, if the wave function is purely a mathematical object, how can it be the case that some physical manifestation of it can be measured and observed?

Good point. The deflected particles are distributed in such a way (for the reasons described above) that this can be described as an oscillating distribution. To infer the wave properties of the particles from an oscillating distribution pattern is exactly what Newton disputed with Young.

By the way, the averaged wave height of a water wave at any point is always zero. It's just that these waves oscillate so slowly that we see the oscillating progression. The analogy of water waves to the fringe distribution of particles is based on drawing a snapshot of the water wave. On the left Young's sketch, on the right the animation with today's possibilities.

|