A body went up for $4 \;s$ in air and then down for $4 \;s$ with the total journey of $8\;s$. Now, the graph above is a $a-t$ graph for the statement.
My question is that why does $g = 0$ when the body reaches surface. I know that when it reaches the surface, the body doesn’t move. But still, there is an acceleration due to gravity of $9.8m/s^2$ on it. It is just that there is a counter-attacking force but how does that make $a$ on the body $0$?
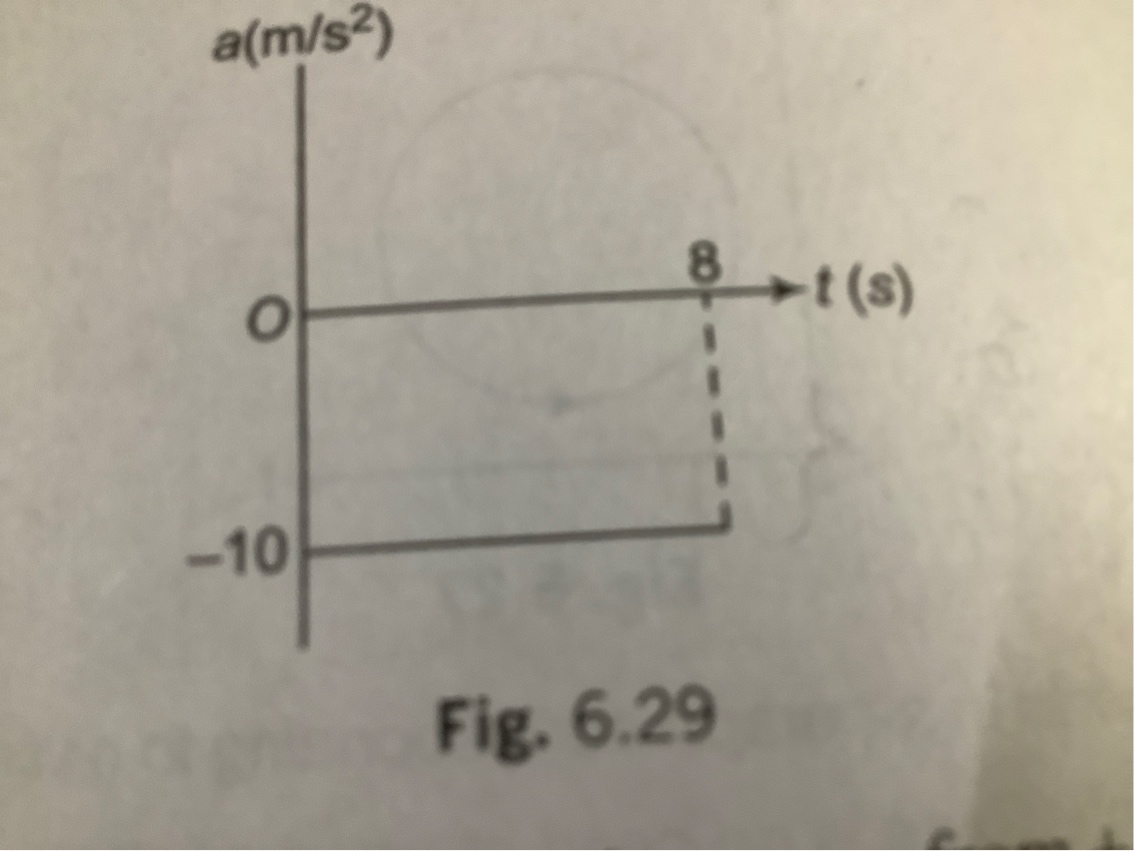
My question is that why does g = 0 when the body reaches surface.
It doesn't! $g$ is the magnitude of the gravitational field of the planet (Earth, in this case). It is $$ |\vec{g}|\simeq\frac{Gm_E}{r^2},$$ where $m_E$ is planet's mass, and $r$ is the distance from the center of the (spherical) planet. At Earth's surface it is approximately 9.8 m/s$^2$, all the time. It just so happens, because of how gravitational forces work, that "the acceleration due to gravity only " ('only' is a key word that gets left out) has a magnitude equal to $g$. (This, IMHO, is one of the big failures in physics pedagogy nomenclature.)
Notice that the graph axis is not showing $g$. It is showing the acceleration, and that results from the net force at the particular time, not merely the gravitational force.
The gravitational field is always present, exerting a force on the mass. But there may be other forces. The acceleration is the result of the net force.
Acceleration is the derivate of speed. If the speed does not change, the acceleration is indeed 0.
As you pointed out, there are 2 forces acting on the body when it lies on the ground. Those 2 forces are equal in magnitude and opposite in direction so the net force (the sum of all the forces) is 0.
Newton's second law is verified: if the net force if zero then the acceleration is also zero.
