WKB application on symmetric potential well

Question

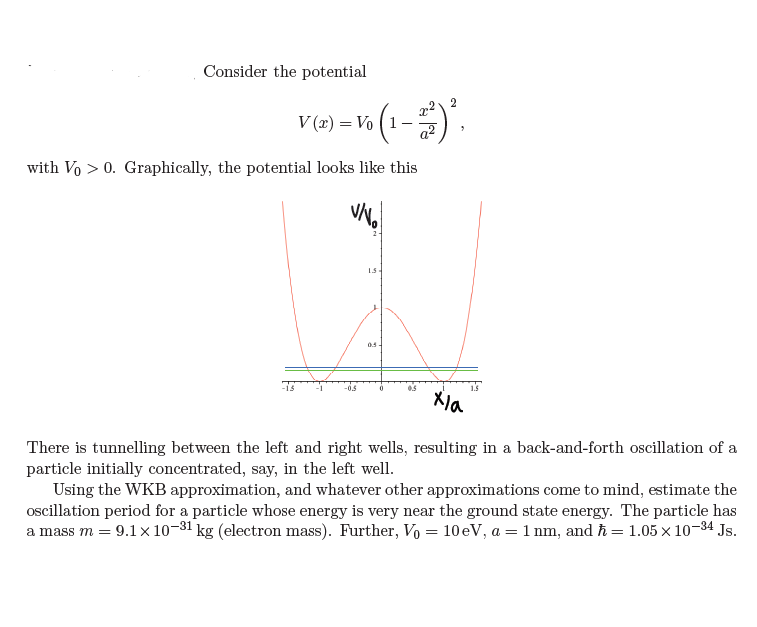
I am a little confused how one can find a wave function by using WKB approximation? I do know the oscillation frequency $$\Omega ~=~ {2E\over h}{\rm Re} \langle L|R \rangle~=~ {E\over \pi\hbar}{\rm Re} \langle L|R \rangle, $$

where $L$, $R$ are the eigenstate of the left and right well.

However, the key of finding this inner product is a pain to me, can someone teach me through it?

Answer 1

Hints:

1. In Ref. 1 it is claimed that $$ \frac{2\pi}{\tau}~=~\Omega~=~\frac{E_n^--E_n^+}{\hbar}~=~\frac{\omega}{\pi}e^{-\phi},\tag{8.63/8.64}$$ where $$ \phi~\equiv~ \int_{-x_1}^{x_1} \!dx |k(x)|, \tag{8.60}$$ so that $$ \phi \sim~ \alpha a^2 \quad \text{for} \quad V(0)\gg E \quad \text{where} \quad \alpha~\equiv~ \frac{m\omega}{\hbar}.$$

2. Now let's for simplicity assume $n=0$. If we define $$\begin{align}\psi_{R/L}(x)~=~&A\exp\left(-\frac{\alpha}{2}(x\mp a)^2\right)~=~\psi_{L/R}(-x), \cr A~\equiv~& \left(\frac{\alpha}{\pi}\right)^{1/4},\end{align}$$ to be the $E_0=\frac{\hbar\omega}{2}$ ground state in the right/left well $$ V_{R/L}(x)~=~\frac{1}{2}m\omega^2(x\mp a)^2, $$ then indeed $$\begin{align}\langle L | R\rangle~=~& \int_{\mathbb{R}}\! dx~\psi_L(x)\psi_R(x)\cr ~=~&\sqrt{\frac{\alpha}{\pi}}\int_{\mathbb{R}}\! dx~\exp\left(-\alpha(x^2+ a^2)\right)\cr ~=~&e^{-\alpha a^2}~\sim~e^{-\phi}. \end{align}$$

References:

1. D. Griffiths, Intro to QM, 1995; problem 8.15.

2. L.D. Landau & E.M. Lifshitz, QM, Vol. 3, 2nd & 3rd ed, 1981; $\S50$ problem 3.