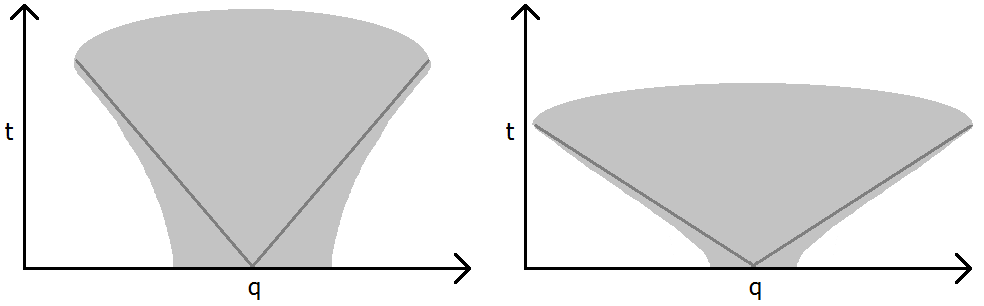
When deriving LSZ formulae, we assume asymptotic particles’ creation/annihilation operators as: $$a_\text{g,in/out}\ \ (\mathbf{p})\equiv \int d^3k \ g(\mathbf{k}) a_\text{in/out}(\mathbf{k}), \ \text{where}\ \ g(\mathbf{k}) =\exp\left(-\frac{(\mathbf{p} - \mathbf{k})^2}{2\sigma^{2}}\right).$$
This is because to get such normalized initial/final states as can define weak convergence of asymptotic creation/annihilation operators, and to ignore the interaction between different particles in initial/final states. However, after computing the LSZ, $g(\mathbf k)$ term is ignored by taking the limit of $\sigma \rightarrow 0$ & integrating about $\mathbf{k}$.
Here are some questions.
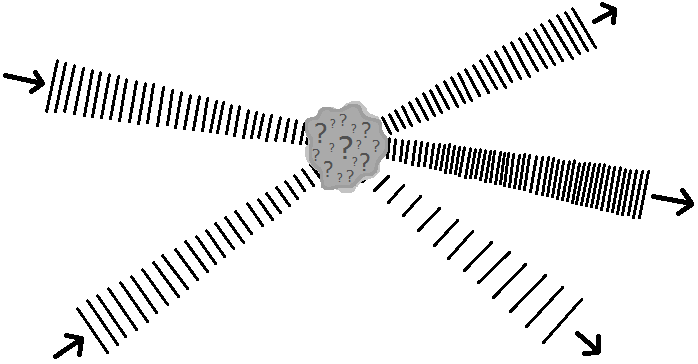
- Why can we ignore $g(\mathbf{k})$ in LSZ? I think such the limit abandons spacial localization of particles at initial and final state and it makes particles interact even in the initial state.
- Even if such the limit is physically correct, what is the difference between assuming the localized operator $a_\text{g,in/out}(\mathbf{k})$ or not. In other word, why should we introduce the $a_\text{g,in/out}(\mathbf{k})$ even though we make wave packets collapse anyway by taking the limit $\sigma \rightarrow 0$ at the end of derivation?
- What is the difference between simply taking plane waves as an asymptotic state and to get plane waves by collapsing wave packets? When we take the limit $\sigma \rightarrow 0$, I think wave packets correspond to delta function, so it seems meaningless to define $a_\text{g,in/out}(\mathbf{k})$ and its spatial locality.
I have already read this post and this post, yet never understood clearly.
References
M. Srednicki, QFT ; chapter 5.
Peskin & Schroeder, QFT; sections 7.1-7.2
Wikipedia, LSZ reduction formula