I've performed a CFD of a flow inside a nozzle and I'm evaluating the differences between various settings. I cannot find a reason why after the shock wave formed in the divergence section, the Mach number rises (as can be seen in the blue dotted line) and the pressure decreases.
I don't understand the physical reason behind that. Shouldn't the shock just be a bit downstream with pressure increasing and M decreasing monotonically (after the shock)?
Much of this answer comes from the following: https://physics.stackexchange.com/a/378783/59023
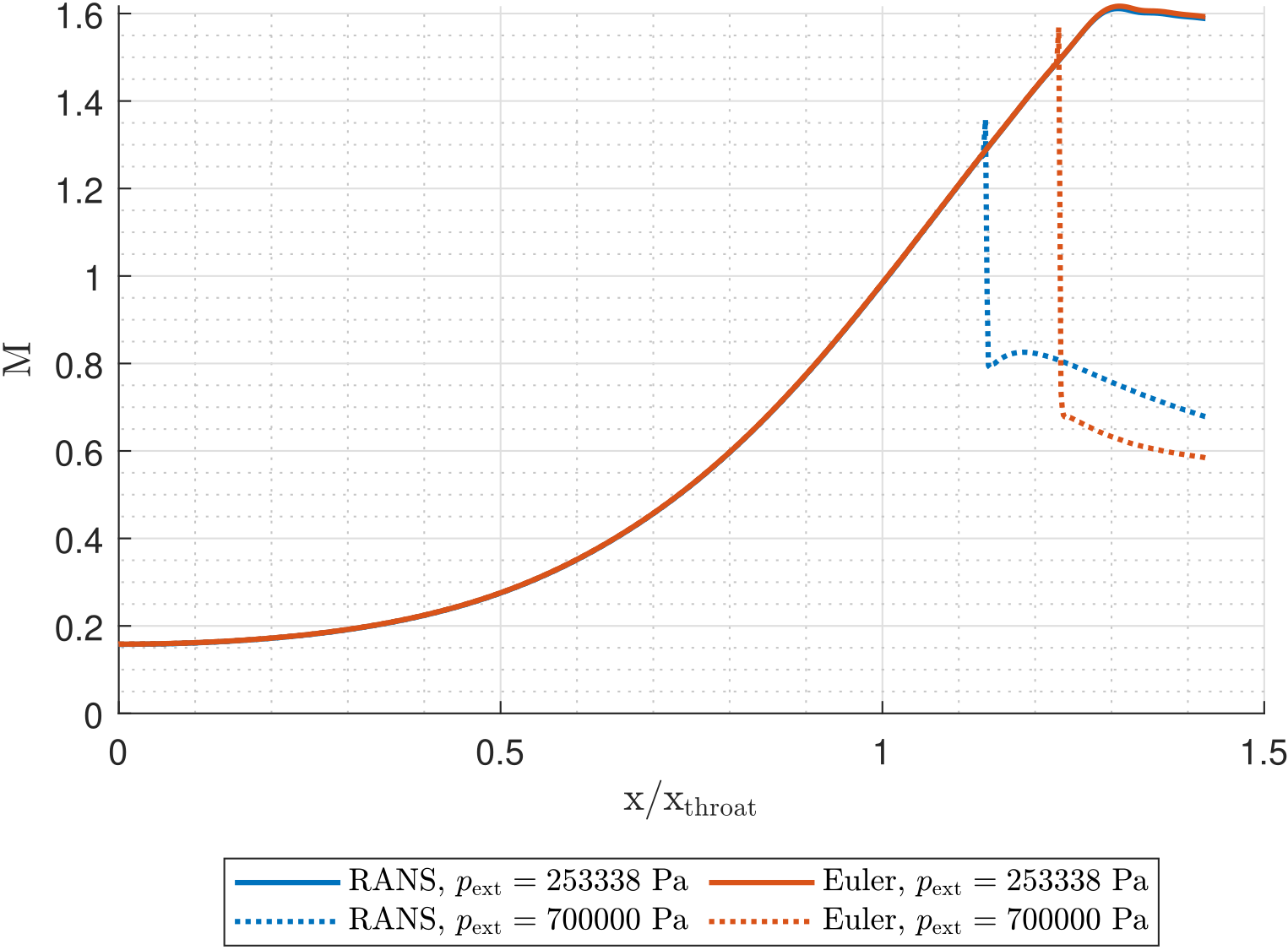
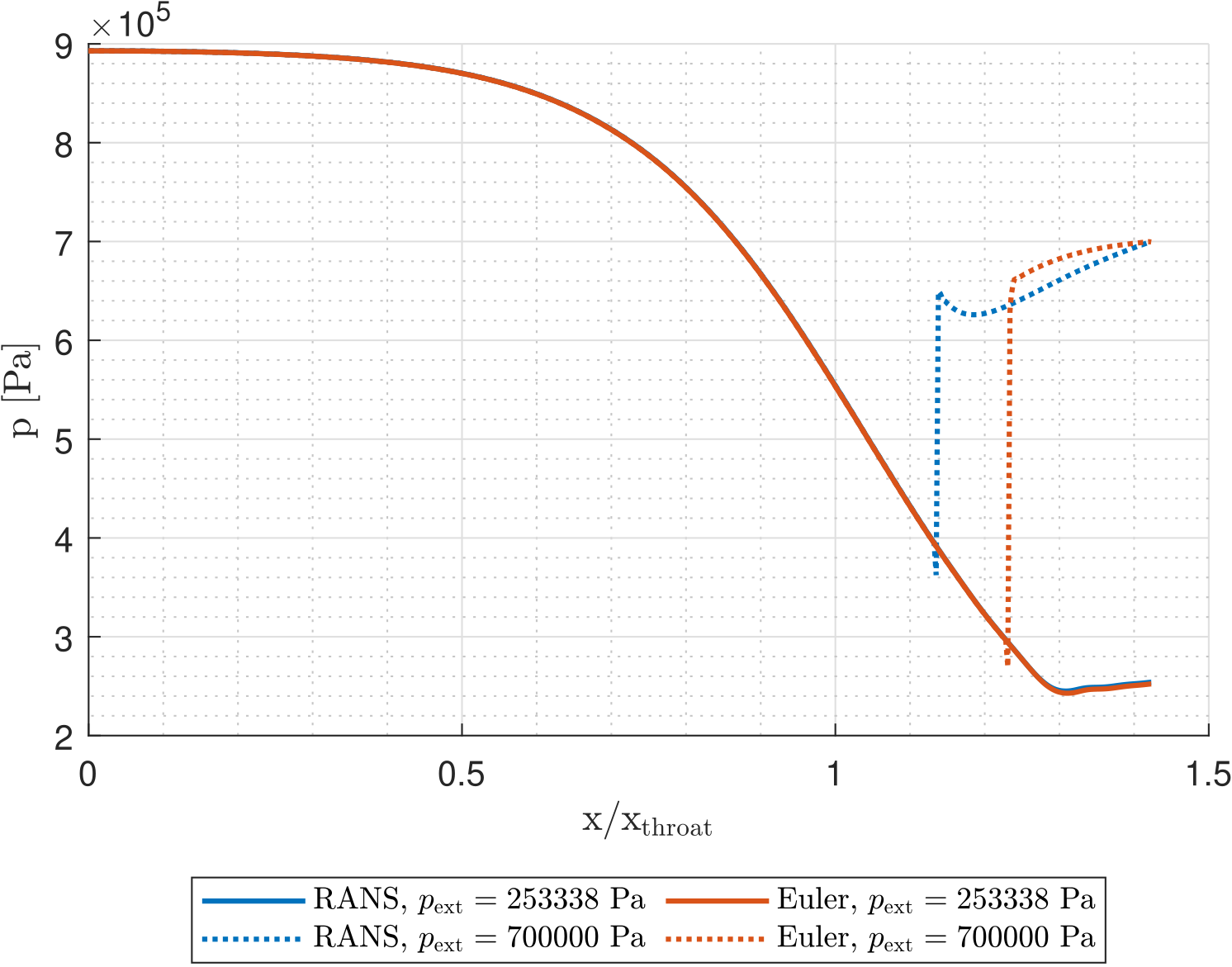
I cannot find a reason why after the shock wave formed in the divergence section, the Mach number rises (as can be seen in the blue dotted line) and the pressure decreases... Shouldn't the shock just be a bit downstream with pressure increasing and M decreasing monotonically (after the shock)?
If we assume isentropic nozzle flow, we can show that: $$ \frac{du}{u} \ \left( M^{2} - 1 \right) = \frac{dA}{A} \tag{0} $$ where $M$ = Mach number, $u$ is the fluid speed, and $A$ is the nozzle area.
From Equation 0, one can see the following list:
The situation in your case is the third bullet. You want the flow to become supersonic just after the constriction point so that both $M$ > 1 and $dA$ > 0 are satisfied, which leads to $du$ > 0 (i.e., acceleration).
As for the pressure, since we assumed an isentropic flow, we can use the ideal gas approximation for an adiabatic fluid given by: $$ P \ \rho^{-\gamma} = \text{constant} \tag{1} $$ where $P$ is the thermal pressure, $\gamma$ is the polytropic index and $\rho$ is the mass density. We can also use the continuity equation to show that: $$ \rho \ A \ u = \text{constant} \tag{2} $$ where $A$ is again cross-sectional area and $u$ is fluid flow speed. Finally the conservation of energy for an isentropic process is given by: $$ \frac{ 1 }{ 2 } u^{2} + \frac{ \gamma }{ \gamma - 1 } \frac{ P }{ \rho } = \text{constant} \tag{3} $$ Differentiating Equation 1 gives us: $$ dP = \frac{ \gamma \ P }{ \rho } d\rho = C_{s}^{2} d\rho \tag{4} $$ where $C_{s}$ is the speed of sound. We can do some arithmetic on Equations 1, 2, and 3 to show that: $$ \frac{ du }{ u } = -\frac{ 1 }{ M^{2} } \frac{ d\rho }{ \rho } \tag{5} $$
From our discussion above, we know that $du$ > 0 therefore to make sure Equation 5 is consistent this would require $d\rho$ < 0. We can then see from Equation 4 if $d\rho$ < 0 then $dP$ must also be less than zero, i.e., pressure decreases.
More details about this can be found in the answer at: https://physics.stackexchange.com/a/524215/59023.
