0
$\begingroup$

Sometimes the Landau levels in a finite 2D sample drawn as in the figure below:

enter image description here

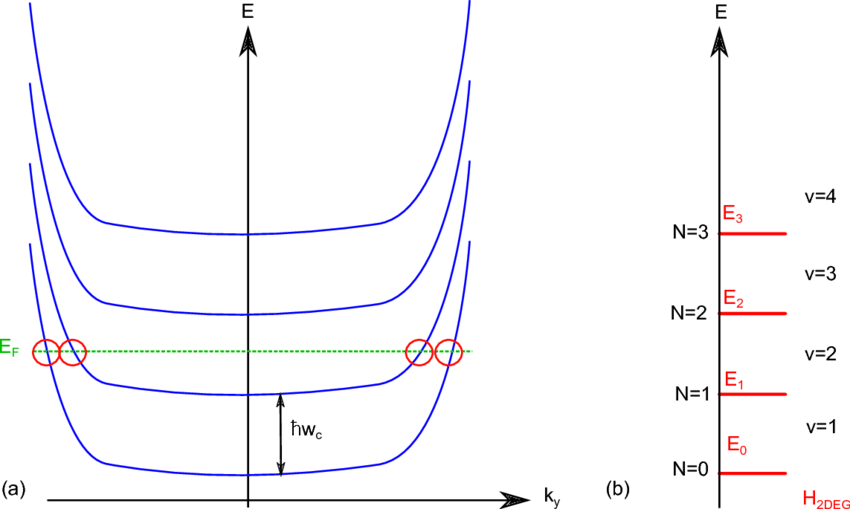
where the energy $E$ is graphed against the width $x$ of the sample in real space where $x=0$ and $x=W$ are the two edges of it. But sometimes it is also graphed in the $E$ vs $k$ plane as in the figure below: enter image description here

Which one of them is the correct representation of the Landau levels?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
3
$\begingroup$

Both. Remember (or note) that in the Landau gauge $\vec A = xB\hat y$, the energy eigenfunctions of a 2D particle in a uniform magnetic field are plane waves in $y$ and exponentially localized in around $x=k \ell_B^2$, with $\ell_B \equiv \sqrt{\frac{\hbar}{eB}}$ and $\hbar k$ the momentum in the $y$-direction. As a result, you could either describe the energy of these states via the $x$-coordinate about which they are localized, or $k = x/\ell_B^2$.

Improve this answer
$\endgroup$
6
  • $\begingroup$ Thanks, for your answer. I have not seen how the first graph is obtained. Ideally, one should add the classical confining potential $V(x)$ to the Hamiltonian for an electron in a magnetic field, and recalculate the dispersion relation. The second graph seems to me to be obtained only heuristically by superposing the classical confining potential $V(x)$ (which is flat in the interior and climbs up at the edges) on top of the quantized Landau levels? Can you say something about how $E-k$ is obtained? Does it require a numerical solution of the Schrodinger equation? $\endgroup$
    – Solidification
    Commented Feb 3, 2021 at 15:52
  • $\begingroup$ Once the $E-k$ graph is justified, then your answer gives a valid argument for $E-x$ graph. $\endgroup$
    – Solidification
    Commented Feb 3, 2021 at 15:55
  • $\begingroup$ @mithusengupta123 Yes - if you add a confining potential $V(x)$ to the Hamiltonian, then you'll need to solve the resulting equation numerically in order to obtain the spectrum. If you stay in the Landau gauge, then you'll still be able to write down $H_k$ by replacing $P_y$ with $\hbar k$ (since the confining potential only involves $x$), but the eigenfunctions will not be simply "harmonic oscillator" eigenstates, and the relationship between the localized $x$ coordinate will have a more complex relationship with $k$. $\endgroup$
    – J. Murray
    Commented Feb 3, 2021 at 16:10
  • $\begingroup$ @mithusengupta123 The general recipe in real materials is to consider the bulk of a sample and the surfaces/edges separately, and then heuristically join the two together as you have seen above. Though numerical solutions are possible (at least in simple cases), they typically don't yield much insight beyond what you'd get by following this simpler prescription (computing small corrections if necessary), and unless the dimensions of your sample are incredibly small (in this case, on the order of $\ell_B$) the improvement in accuracy is pretty minimal. $\endgroup$
    – J. Murray
    Commented Feb 3, 2021 at 16:15
  • $\begingroup$ @mithusengupta123 You may be interested in papers like this one which study this problem numerically (though they use the symmetric gauge and a circular confining potential). The result is a splitting of the landau levels which depends on the parameter $\ell_B / L_x$ - an incredibly small number for strong magnetic fields and macroscopic samples. In such cases, the only real change is in the higher energy states, localized near the "edges" of the well. $\endgroup$
    – J. Murray
    Commented Feb 3, 2021 at 16:25

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.