Semi-major axis and ellipticity of a binary system?

Question

In the image below (source at bottom), it seems to be suggesting that

\begin{equation} a = a_{1} + a_{2}, \hspace{8cm}(1) \end{equation} where $a_{1}$ and $a_{2}$ are the semi-major axis of the two orbits, and $a$ is the semi-major axis of the trajectory for the equivalent reduced-mass star in the one-body problem.

It also says that \begin{equation} e_{1} = e_{2} = e, \hspace{8cm} (2) \end{equation} which is to say that both orbits have the same ellipticity, which is also equal to the ellipticity again of the trajectory for the equivalent reduced-mass star in the one-body problem.

What I'd like to know is: why are (1) and (2) true? Specifically, I have a binary system and I'm working in the centre of mass frame. I'd like go to the reduced-mass particle system and so I want to know how the properties of this new system relate to those of the first system.

Image courtesy of: http://faculty.wwu.edu/vawter/PhysicsNet/Topics/Gravity/BinaryStars.html

Answer 1

Why is Equation 1 true?

Equation 1 stems from the definition of the reduced mass system. The reduced mass system is an accelerating frame of reference for a binary star system in which the heavier star is seen as having no acceleration. However, the physical orbital properties of the reduced mass system must naturally be identical to those of the original binary star system, since both describe the same pair of orbiting bodies. (Another way to think of this is to realize that the reduced mass system is simply a result of viewing the original binary system in an accelerating reference frame, so distances are unchanged.)

Therefore, the distance between the two stars must be identical in either system. In the reduced mass system, the distance was $a$, and in the original system, it was $a_1 + a_2$. Therefore, $a = a_1 + a_2$.

Why is Equation 2 true?

First, we consider that there is no external force acting on the system as a whole. Therefore, the center of mass is not accelerating, and there is an inertial reference frame in which the center of mass of the stars is stationary. This is the reference frame that will be used.

Next, we remind ourselves that the ratio of distances $r_1$ and $r_2$ remains constant throughout the orbit. For $O$ to be the center of mass, $r_1 m_1 = r_2 m_2 = ((r_1 + r_2) - r_1) m_2$. Solving for $r_1$, we find that:

$$ r_1 = (r_1 + r_2) \frac{m_2}{(m_1 + m_2)}$$ and $$ r_2 = (r_1 + r_2) \frac{m_1}{(m_1 + m_2)}$$

Therefore, $$\frac{r_1}{r_2} = \frac{m_2}{m_1}$$

And the ratio of the two distances is always constant.

Now, we use the geometric principle of homothety (Wikipedia) to complete the proof. Homothety states that if every point in an image is flipped around a "center point" in equal proportions, then the resulting image will be similar to the original one. This is demonstrated in the image below:

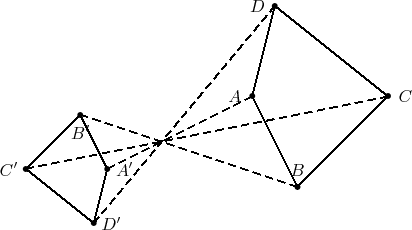
In this case, the center of our transformation is the center of mass of the system. Since the ratio of $r_1$ to $r_2$ is constant, we know each ellipse is similar to the other. Since the ellipses are similar, they clearly have equal eccentricities.

Source: http://farside.ph.utexas.edu/teaching/336k/Newton/node50.html

Answer 2

The equation of motion in polar coordinates of this system, which is the two-body problem, can be written in terms of the relative instantaneous distance $r=r_1+r_2$ between the two bodies, in an inertial reference frame where the center of mass is still. The solution of that equation is an ellipse and represents the relative motion of one particle relative to the other. But the center of mass is located at a constant percentage of the relative distance $r$, i.e. in every instant $r_1 = c_1r$ and $r_2=c_2r$ (with constants $c_1,c_2$), by definition of center of mass. Therefore, both $r_1,r_2$ will draw an ellipse that its just a scaled ellipse of the original, so they have the same shape (equal eccentricity $e$) and proportional linear distances to the origin, making $a=a_1+a_2$.