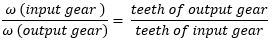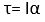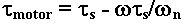1
$\begingroup$

This question came up watching a final sprint at the Vuelta San Juan this year. One of the riders was sitting down in the sprint because he had "spun out" his gears, meaning there was no longer any resistance in the drivetrain to his pedaling motion. The commentator mentioned that in order to spin out a gear ratio of 53:11, you would have to be going fast. How could you determine this speed?

Here was my first approach. At first I used one of the gear equations:

enter image description here

I estimated that at 120 rpm your legs would no longer feel any resistance to pedaling (this may be a little high realistically). I did some calculations and conversions and got a linear speed of the bike of 46 mph. This seems pretty realistic given what I know about bike racing.

However, I was wondering if there is another method to this. Could one develop an equation like this:

enter image description here

Then set the torque_pedal variable to zero (or near zero) and find the velocity?

I attempted several equations but couldn't come up with something that worked. Either the values seemed unrealistic or I just ended up with x = x, or everything cancelled itself out. Here are the starting points I used:

enter image description here

F = ma

enter image description here

I can't seem to really get the intuition of the problem this way. I guess you would be looking for the rotational speed of the rear wheel such that the 53:11 gear ratio could no longer accelerate the rear wheel?

Anyone have any ideas on this?

Improve this question
$\endgroup$
6
  • $\begingroup$ Aren't you merely asking how fast human legs can turn the cranks? If so, it's not really a physics question. It's more about human physiology. $\endgroup$
    – Solomon Slow
    Commented Dec 3, 2020 at 17:38
  • $\begingroup$ @SolomonSlow Maybe. However, intuitively it seems to me that there would be a point for a given gear ratio, rider+bike mass, and rolling friction (ignoring air resistance) at which the rider would "exhaust" the capabilities of that gear ratio to continue to accelerate the bike. This would be the case whether it was a human pedaling or a robot. $\endgroup$
    – Ryan C
    Commented Dec 3, 2020 at 20:43
  • 1
    $\begingroup$ @SolomonSlow Actually this may have just clicked for me. I think you are right. The sensation of "spinning out" a gear it merely due to the inability of the rider's legs to produce the required RPMs in that gear ratio to continue to accelerate the bike. Obviously a motor could push RPMs way higher than any human would go. In that case I think my first approach is the best way. Start with the human limit of pedaling RPMs and work backwards. I guess I couldn't figure out the second method because there was no answer. $\endgroup$
    – Ryan C
    Commented Dec 3, 2020 at 20:49
  • $\begingroup$ I have misunderstood this problem. This really has to do with human limitations, not a physics problem. My first method is correct, the second, which I have asked a question about, does not have an answer. $\endgroup$
    – Ryan C
    Commented Dec 4, 2020 at 12:12
  • $\begingroup$ don't ignore air resistance $\endgroup$
    – Robert DiGiovanni
    Commented Dec 4, 2020 at 14:50

1 Answer 1

Reset to default
0
$\begingroup$

Ryan, give yourself more credit. The speed limit for a bicycle is not the ability to pedal fast. It is the ability to produce enough thrust force over a given distance per unit time to match drag x distance/time.

A cyclist can easily "spin out" in first gear, which is why we move up to higher gears. At top speed, the amount of geared force is equal to drag (the higher the speed, the stronger your leg muscles need to be to use the higher gear and not spin out).

If the cyclist is a well conditioned athlete, the bicycle designer would be wise to add more gears! (12 speed?). As with a car or truck, the gears should match the "engine".

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.