-4
$\begingroup$

I know many people (including me) are bombarding this site with questions about the recent Veritasium video about the one-way speed of light, but I think I found a way.

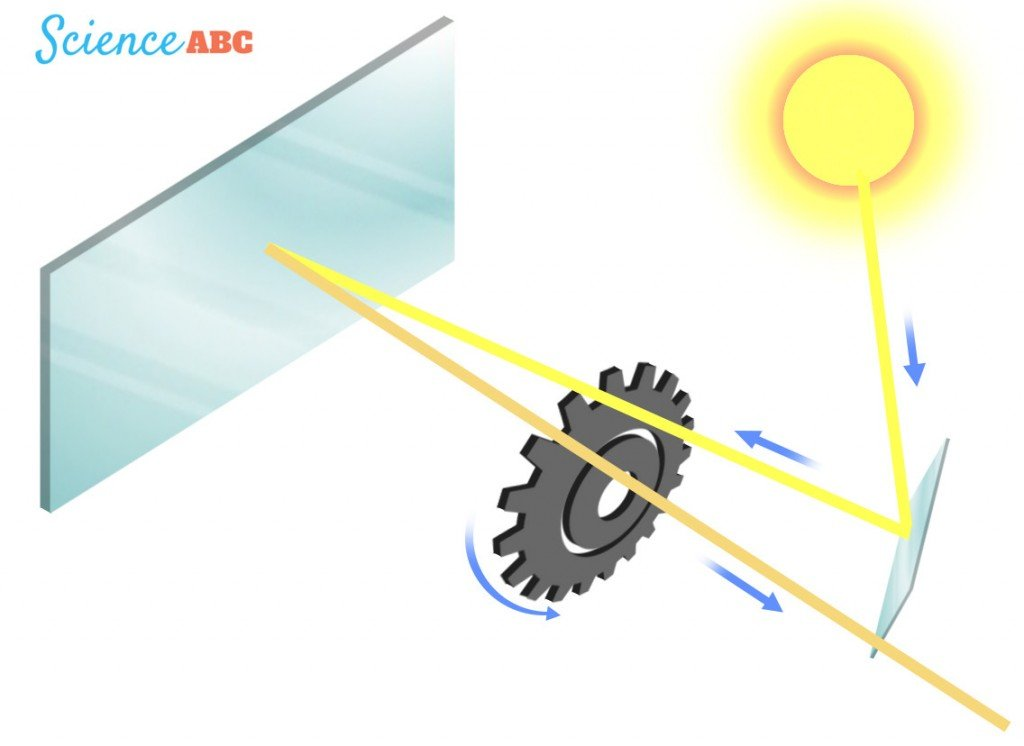
Here it is: (You can see sort of what I am talking about in the picture I attached. Just pretend the mirrors aren't there.)

You could set up a light source and direct it toward a spinning object/wheel with teeth (like the diagram). Then, by adjusting the rotation rate of the wheel, you could see when the light reaches the other side of the wheel/gear (no need to measure how long it takes to get there or back—this eliminates the need for clocks at all [other than any ones need for calibrating the rotation of the wheel]). By knowing the distance the light has to travel to get to the gear, you would be able to calculate its speed if you knew that it passed through the gear at a certain time (based on the rotation rate of the wheel). I know this is very similar to how the speed of light was calculated by Fizeau, but by eliminating the reflection of the light, I believe this setup would work. Am I missing something, or is this possible?

Also, please let me know if my procedure was too incoherent.

Improve this question
$\endgroup$
6
  • $\begingroup$ "other than any ones need for calibrating the rotation of the wheel" <- there's the rub. How do you make sure the two wheels are in sync? With, uh, clocks. Or by transmitting a signal which moves at light-speed or less. Those ideas are all covered in the video. $\endgroup$
    – Criticizing Israel not allowed
    Commented Nov 3, 2020 at 13:45
  • $\begingroup$ And to be clear, because it's not obvious from your diagram: your solution is to use two wheels instead of using a mirror that reflects back through the same wheel, right? $\endgroup$
    – Criticizing Israel not allowed
    Commented Nov 3, 2020 at 16:24
  • $\begingroup$ @user253751 No, I was thinking just a light source, wheel, and detector. $\endgroup$
    – user276997
    Commented Nov 3, 2020 at 17:12
  • $\begingroup$ oh well then I don't understand how it measures the speed. Unless it compares the time the light hits the detector with the time it came through the wheel? Then it's the same as if you had a flashing light and a detector. Which is the first thing you would think of to measure the speed of light. And they debunked it in the video. $\endgroup$
    – Criticizing Israel not allowed
    Commented Nov 3, 2020 at 17:58
  • $\begingroup$ @user253751 I was thinking that the fact that the light passes through the gear is the information needed. The time that it takes the light to reach the gear could be calculated using the rotation rate of the gear. However, as others have answered, this wouldn't work because you woulnd't be able to calibrate the wheel's rotation without using a clock, which wouldn't be synchronized with the light source. $\endgroup$
    – user276997
    Commented Nov 3, 2020 at 18:18

4 Answers 4

Reset to default
2
$\begingroup$

This experiment will not measure the speed of light at all, let alone the one way speed (which is impossible to measure without making a simultaneity assumption).

For this specific experiment, no speed is measured at all. We have a spinning gear which alternately blocks and passes light. On the detector side we get a trace that shows that light is alternately blocked and passed. There is no information in that trace that allows us to determine the speed of light. Regardless of the speed of the signal or the length of the path, the trace will be the same.

To turn this into a measurement of speed would require a pair of synchronized clocks so that you can find when the gear tooth blocks the light and when the detector trace shows the light being blocked. Without that additional time information the trace gives no speed information at all. Unfortunately, the usual synchronization process already assumes the one way speed of light is isotropic, which is precisely what we were trying to measure.

In general, any measurement of the one way speed of light will explicitly or implicitly assume some synchronization convention. That assumption determines the measured speed. So there is no way to measure the one way speed of light. Since the two way speed of light is isotropic, it makes sense to use the convention that the one way speed of light is also isotropic, but it is a convention.

Some people think that it is only a matter of adding one more complication to their experiment, but it is not. The definition of the one way speed of light depends on your time coordinates and therefore your choice of synchronization convention. It is not a matter of being a clever experimenter, it is simply a matter of definitions.

Improve this answer
$\endgroup$
6
  • $\begingroup$ Ok, thank you! It just really bugs me that there is no answer; I don't like that. $\endgroup$
    – user276997
    Commented Nov 3, 2020 at 15:51
  • 1
    $\begingroup$ Well, it is not that there is no answer. The point of a convention is that there is more than one answer, none of which have any physical consequence. Since there is no physical consequence you just pick one, in this case the one to pick is based on convenience. $\endgroup$
    – Dale
    Commented Nov 3, 2020 at 15:56
  • $\begingroup$ Ok, but (maybe I’m misinterpreting what you mean when you say “more than one answer”) how does light both travel at the same speed and all directions and not? I understand that there may be no consequences of either one, but you said that there is “more than one answer,” so how are they both correct? Wouldn’t one be correct, but we just wouldn’t know it? $\endgroup$
    – user276997
    Commented Nov 3, 2020 at 17:14
  • 1
    $\begingroup$ @NotSoPedantic No, when something is a "convention" it doesn't mean that there is one correct answer and we just don't know, it means that nature simply doesn't care and we can choose. There is no right or wrong at all. The convention doesn't describe nature at all, it only describes our theory. An example is the negative sign of the charge of an electron. There is no sense in which the negative sign is right or wrong, it isn't part of nature. We just agreed to call it negative. There simply is no truth to the matter in nature. Perhaps surprisingly, the one-way speed of light is the same $\endgroup$
    – Dale
    Commented Nov 3, 2020 at 19:44
  • $\begingroup$ Didn't Romer measure the one way speed of light? $\endgroup$
    – jim
    Commented Jul 7, 2021 at 14:17
1
$\begingroup$

I wanted to add this as a comment, but can't because of low reputation, so I will write a bit more.

The experiment is build like this:

light source------------rotating wheel--------------detector

As soon as the rotating wheel is out of the way, the light hits the detector and you measure the time and by knowing the distance get the speed. Do I understand correctly?

I don't think this works. This has the same problem as measuring the speed of light with two clocks (like in the video of Veritasium), but now one clock is exchanged with the wheel. The rotation of the wheel is fixed with a clock and then you move the clock over to the detector. Because the time that is measured in the end is the time difference between the wheel and the clock at the detector. And because of special relativity, this does not work.

Improve this answer
$\endgroup$
1
  • $\begingroup$ I see. I forgot about the second clock. Thanks! $\endgroup$
    – user276997
    Commented Nov 3, 2020 at 15:50
-1
$\begingroup$

I think we can measure one way speed of light. we need to redefine simultaneity. My proposition is as follows: if a rigid body AB of length l is moving without any acceleration parallel to X axis and at time t0 its point A is at location x, then simultaneously its point B is at location x+l

Let's now design the experiment to synchronize distant clocks and measure one way speed of light:

Imagine four spaceships flying as perfect square EFGH towards (or away from) not moving (at least relative to each other) points ABCD, where AD is parallel to EF and distance EF equals AD. Points EG should be collinear with points AB and points FH collinear with CD. Making sure that ABCD (and EFGH) is a square is relatively easy, since 2-way speed of light is constant: we can measure (and correct, if necessary) distances BD and CA by sending light signals from B to D (and from C to A) and back Now we can measure distance from A to G (L) and from D to H (L’) using light (laser) signal send from A to G (and reflected back to A) as well as distance from D to H. If the distance AG (L) equals DH (L’) signals from A and D had been sent simultaneously. Of course the measurements could be done in continuous mode and for example if AG=1000km has been measured at A at 12:00 and DH=1000km at D at 12:15, we would need to adjust the clocks accordingly. Sorry, I could not paste my drawing, but it is simple enough to visualize.

Improve this answer
$\endgroup$
1
-3
$\begingroup$

While most approaches to one-way light speed measurement are ambiguous at best there are instances where the assumption that light speed is isotropic is essential to proper operation of the system. The international pulsar timing network is an example and very long baseline radio astronomy interferometry (VLBI) is another. In both of these applications anisotropy of light speed would introduce significant errors. These errors have never been observed. While it is not absolutely conclusive it is reasonable to assume one-way speed as derived from two-way measurements is valid.

Improve this answer
$\endgroup$
2
  • $\begingroup$ VLBI uses Einstein synchronisation, see here. As Dale explains, in that convention the one-way speed of light equals the two-way speed. $\endgroup$
    – PM 2Ring
    Commented Sep 16, 2022 at 21:09
  • $\begingroup$ As it’s currently written, your answer is unclear. Please edit to add additional details that will help others understand how this addresses the question asked. You can find more information on how to write good answers in the help center. $\endgroup$
    – Community Bot
    Commented Sep 17, 2022 at 8:14