Field in the case of two charged plates [duplicate]

Question

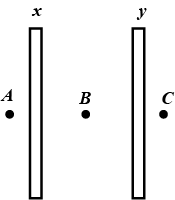
A questions goes like this:

X and Y are large,parallel conducting plates close to each other. Each face has an area A. X is given a charge Q. Y is without any charge. Points A, B and C are as shown in the figure.

To find the field at A, the solution goes; $E_A = E_1 + E_4 = \frac{Q}{4A\epsilon_0}$X$2 = \frac{Q}{2A\epsilon_0}$. The same is held true for the field at $E_C$.

How's this possible? If we label the surfaces from the left to the right as 1, 2, 3, and 4, wouldn't it be surface 3 that affects the field at A? And similarly, wouldn't surface 2 affect the field at C?

Answer 1

I think your question assumes that the plates are infinitely thin and are infinitely large, with plate $X$ having a certain surface charge density, $\sigma$. In other words, they don't have any thickness. Plate $X$ induces a negative charge density $-\sigma$ on plate $Y$ because of their close proximity.

If you assume the positive $x$-axis to be from left to right, the total field at point $A$ due to plates $X$ and $Y$ respectively is $$E_A = -\frac{\sigma}{\epsilon_0} + \frac{\sigma}{\epsilon_0} = 0.$$ By symmetry, the field at $C$ is zero as well (you can work this out if you want). At $B$, the fields add up constructively, resulting in $$E_B = +\frac{\sigma}{\epsilon_0} + \frac{\sigma}{\epsilon_0} = +2\frac{\sigma}{\epsilon_0}$$ toward the positive $x$-axis.