I hope everyone is doing well and staying safe. So instead of simply memorizing the SUVAT equations, I wanted to find out how the equations are derived to broaden my knowledge. I'm currently a high school student who is completing university-level mathematics and somehow ended up doing questions on SUVAT and constant accelerations (haha). Are there any mnemonics or easy way to memorize/understand the equations. Regardless, I am very keen to know how the SUVAT equations are derived.
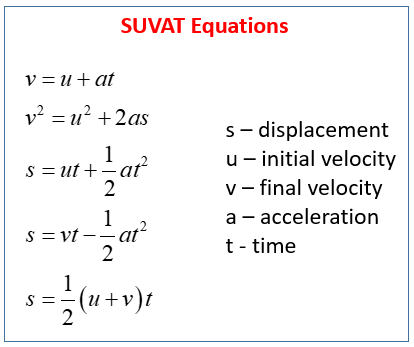
If the acceleration $a$ is constant, then from the calculus definition of acceleration as the time derivative of velocity $$\frac{dv}{dt} = a$$ we see that integrating the left-hand side with respect to $t$ gives $$\int_0^t \frac{dv}{dt} dt = \int_{v(0)}^{v(t)} dv = v(t) - v(0) = v - u,$$ where we set the initial time as $t = 0$ and initial velocity as $v(0) = u$, while on the right-hand side we have $$\int_0^t a dt = a \int_0^t dt = at$$ so $$v = u + at.$$ This is the first equation. Using the definition of velocity as the time derivative of position $$ \frac{dx}{dt} = v$$ we see that integrating the left-hand side gives $$ \int_0^t \frac{dx}{dt} dt = \int_{x(0)}^{x(t)} dx = x(t) - x(0) = s(t)$$ where we set $s(t) = x(t) - x(0)$ as the displacement, and integrating the right-hand side gives $$ \int_0^t v(t) dt = \int_0^t [u + at] dt = u t + \frac{1}{2} at^2$$ so that $$s(t) = u t + \frac{1}{2} at^2.$$ This is the third equation. This is expressed in terms of $u$, we can express it in terms of the final velocity $v$ by taking $v = u + at$ and setting $u = v - at$ to find $$s(t) = ut + \frac{1}{2} a t^2 = (v - at)t + \frac{1}{2} a t^2 = vt - at^2 + \frac{1}{2} a t^2 = vt - \frac{1}{2} a t^2 .$$ This is the fourth equation. We can eliminate $t$ from the third equation $s = ut + \frac{1}{2} at^2$ by using the first equation $v = u + at$ to solve for $t = \frac{v-u}{a}$ and then use this in the third equation $$s = ut + \frac{1}{2} at^2 = u \frac{v-u}{a} + \frac{1}{2} a(\frac{v-u}{a})^2 = u \frac{v-u}{a} + \frac{(v-u)^2}{2a} $$ reduces to $$2a s = 2u(v-u)+ (v-u)^2 = 2uv-2u^2+ v^2 - 2vu+u^2 = -u^2+ v^2 $$ or $$v^2 = u^2 + 2a s.$$ This is the second equation. We can eliminate $a$ from the third equation $s = ut + \frac{1}{2} at^2$ by using the first equation $v = u + at$ to solve for $a = \frac{v-u}{t}$ so that $$s = ut + \frac{1}{2} at^2 = ut + \frac{1}{2} \frac{v-u}{t} t^2 = ut + \frac{1}{2} (v-u) t = \frac{1}{2} (u+v) t .$$ This is the fifth equation. Summarizing:
Thus, using calculus to get the first and the third from the assumption of constant acceleration is the key.
Things naturally change for non-constant acceleration, we can no longer do the step $\int_0^t a dt = a \int_0^t dt$ we have $\int_0^t a(t) dt \neq a(t) \int_0^t dt$ i.e. $\int_0^t a(t) dt$ might not even be something we can compute or we might not even know $a(t)$ as a function of $t$ (for example in the Harmonic oscillator problem $F = - kx$, what is $a(t)$ based on this information? We are forced from $F = ma$ i.e. $-kx = m \ddot{x}$ with $a = \ddot{x}$ to solve the 'second order linear differential equation' $\ddot{x} + k x = 0$ rather than working out a simpler integral, thus you see why constant acceleration problems are important).
You can think of SUVAT as saying
