Motion of ball (air viscosity concerned)

Question

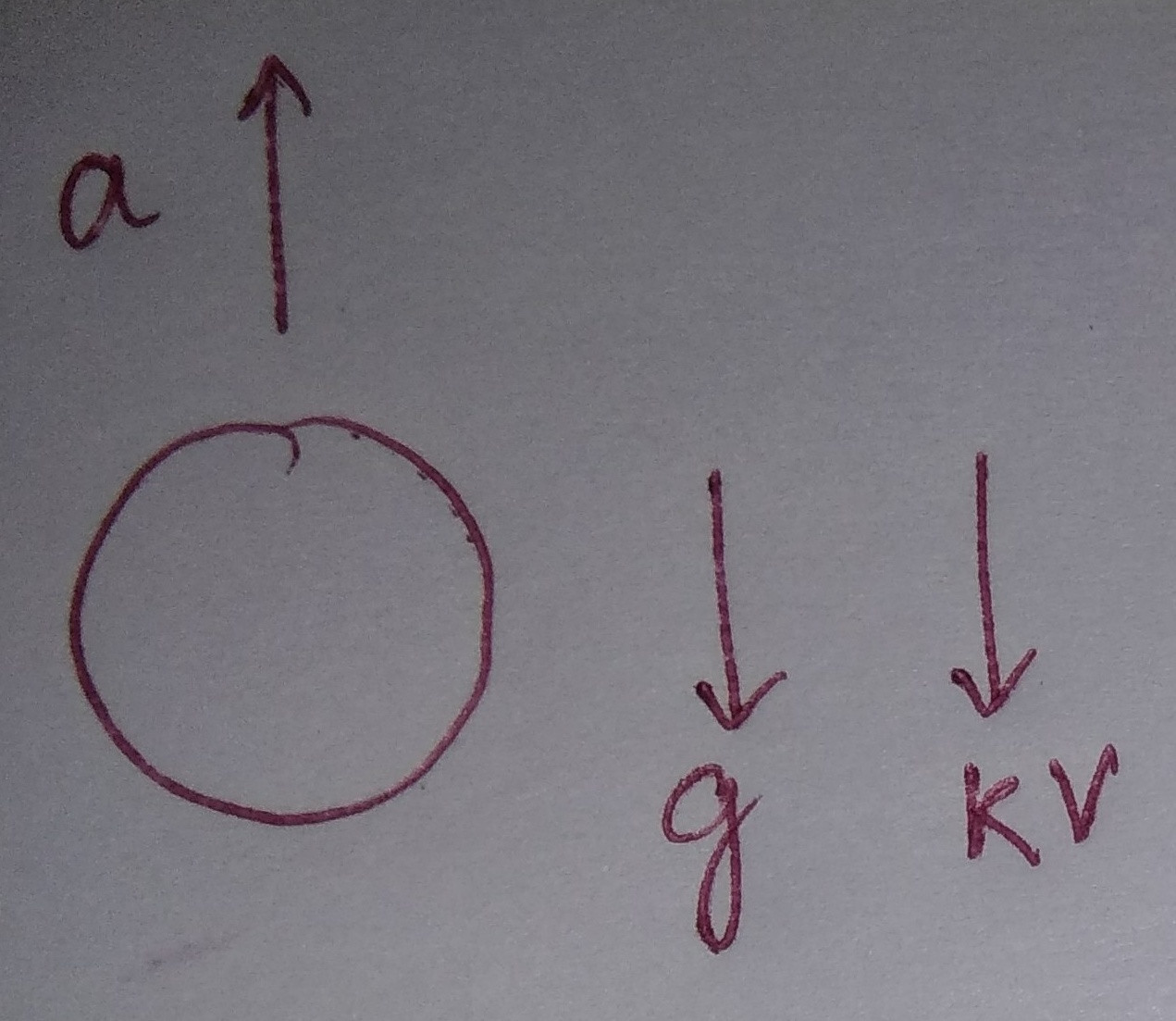
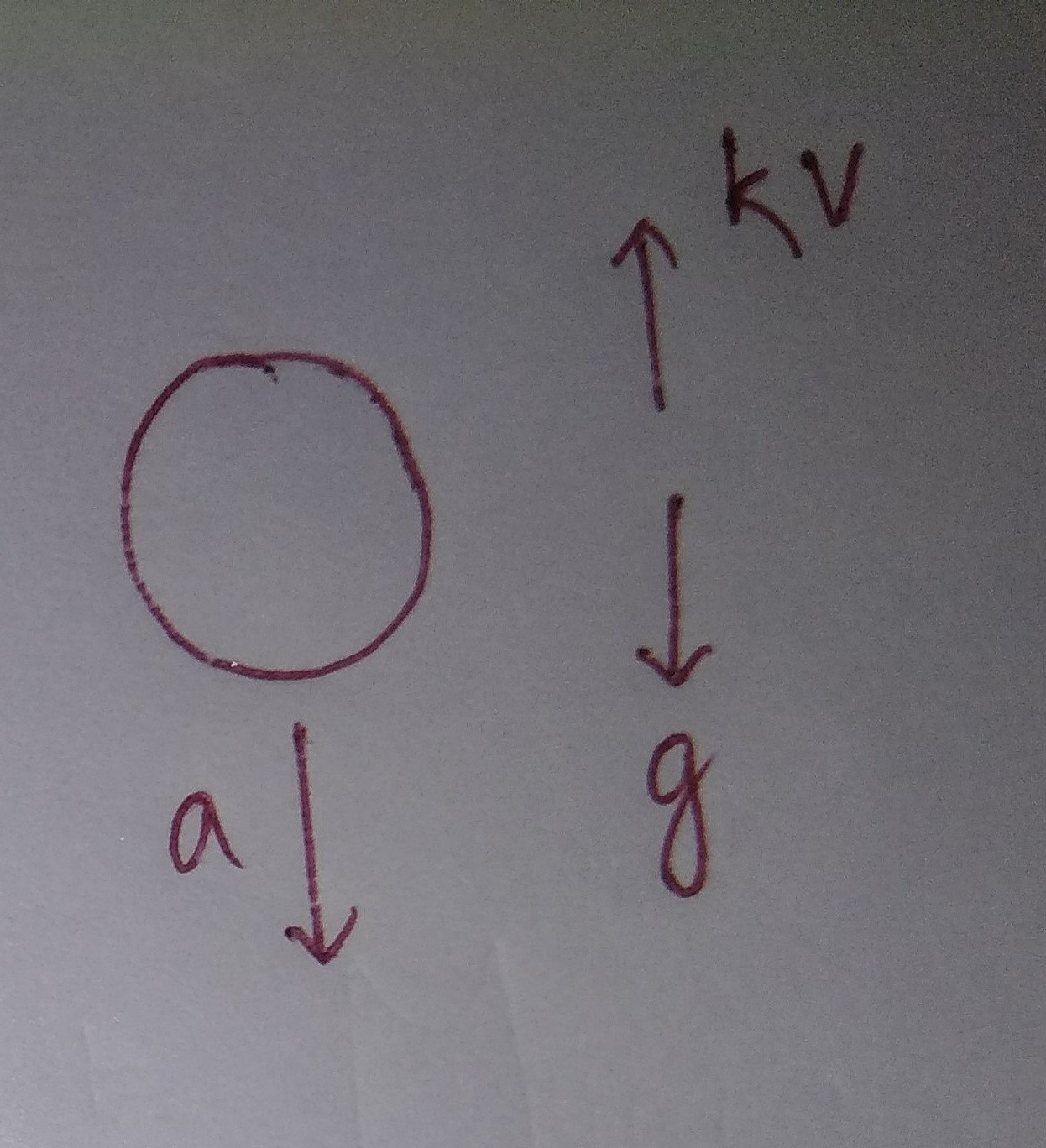
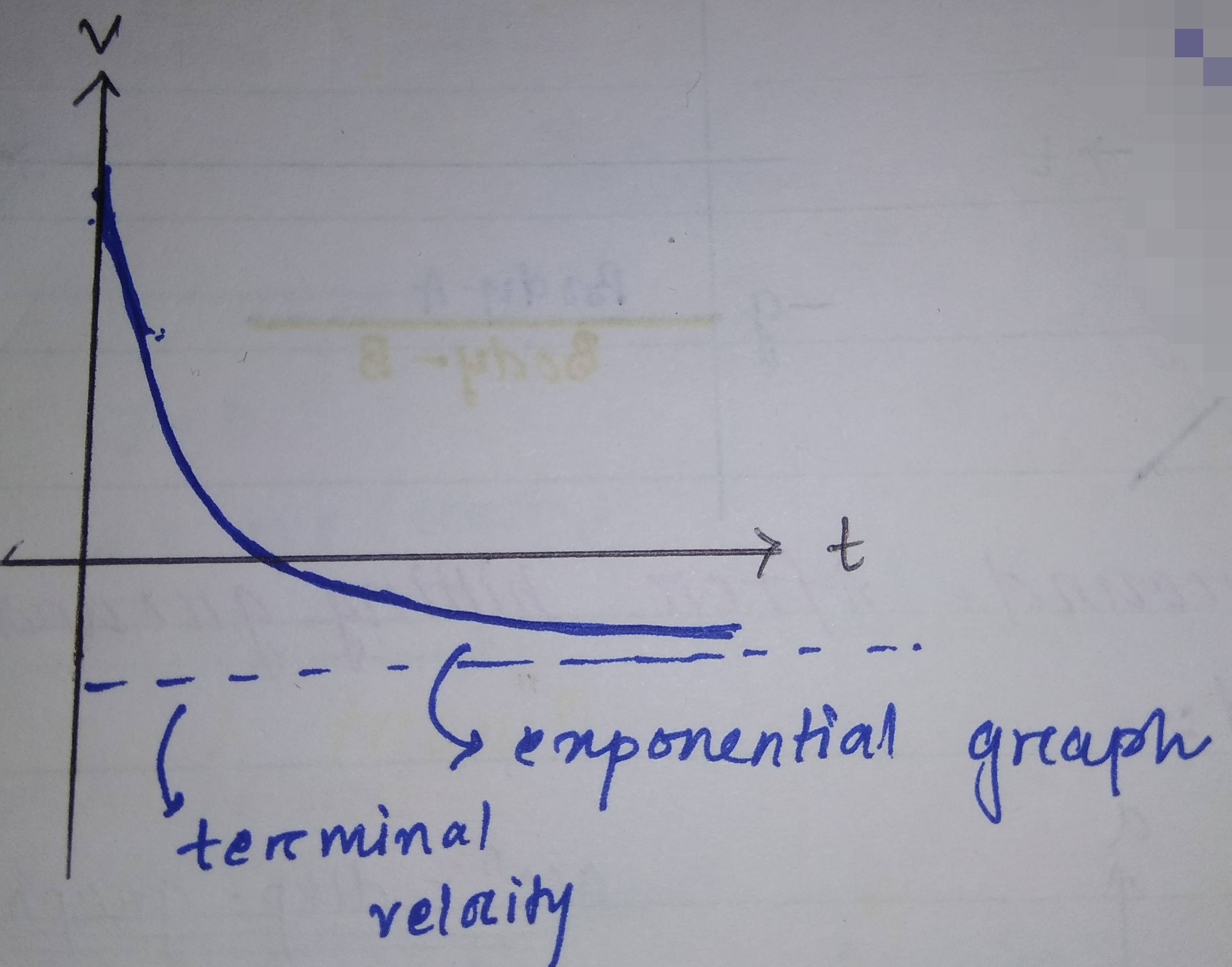
Suppose a ball of mass $m$ is thrown vertically upwards from the ground. I understand that the speed-time graph would be somewhat like a distorted parabola. But what about the velocity- time graph (considering air drag or viscosity)?

According to me it would attain a kind of terminal velocity while falling down. But I am unable to interpret it mathematically.

And sometimes you really need mathematical intuition to see what is happening. So can anyone make a brief mathematical interpretation of this? Thank you.

Answer 1

Let, the viscous force drag, $${F}={k}{v}$$ where ${k}$ is a constant and ${v}$ is the velocity at any instant. While moving up (upward acceleration is negative),

$${ma} = {mg} + {kv}$$ $${a}={g} + \frac{kv}{m}$$

While moving down (downward acceleration is positive),

$${ma}={mg}-{kv}$$ $${a}={g} - \frac{kv}{m}$$

From any of the two equations, it is clear that $$-\frac {dv}{dt} \propto {v}$$ $$\frac {dv}{v} \propto {-dt}$$ $$\int \frac {dv}{v} = {n}\int {-dt}$$ Where $n$ is a constant $${\ln v} = {-nt + c}$$ $$e^{\ln v}= e^{-nt+c}$$ $${v} = e^{-nt+c}$$

Thus the graph will have an asymptote which represents the terminal velocity.