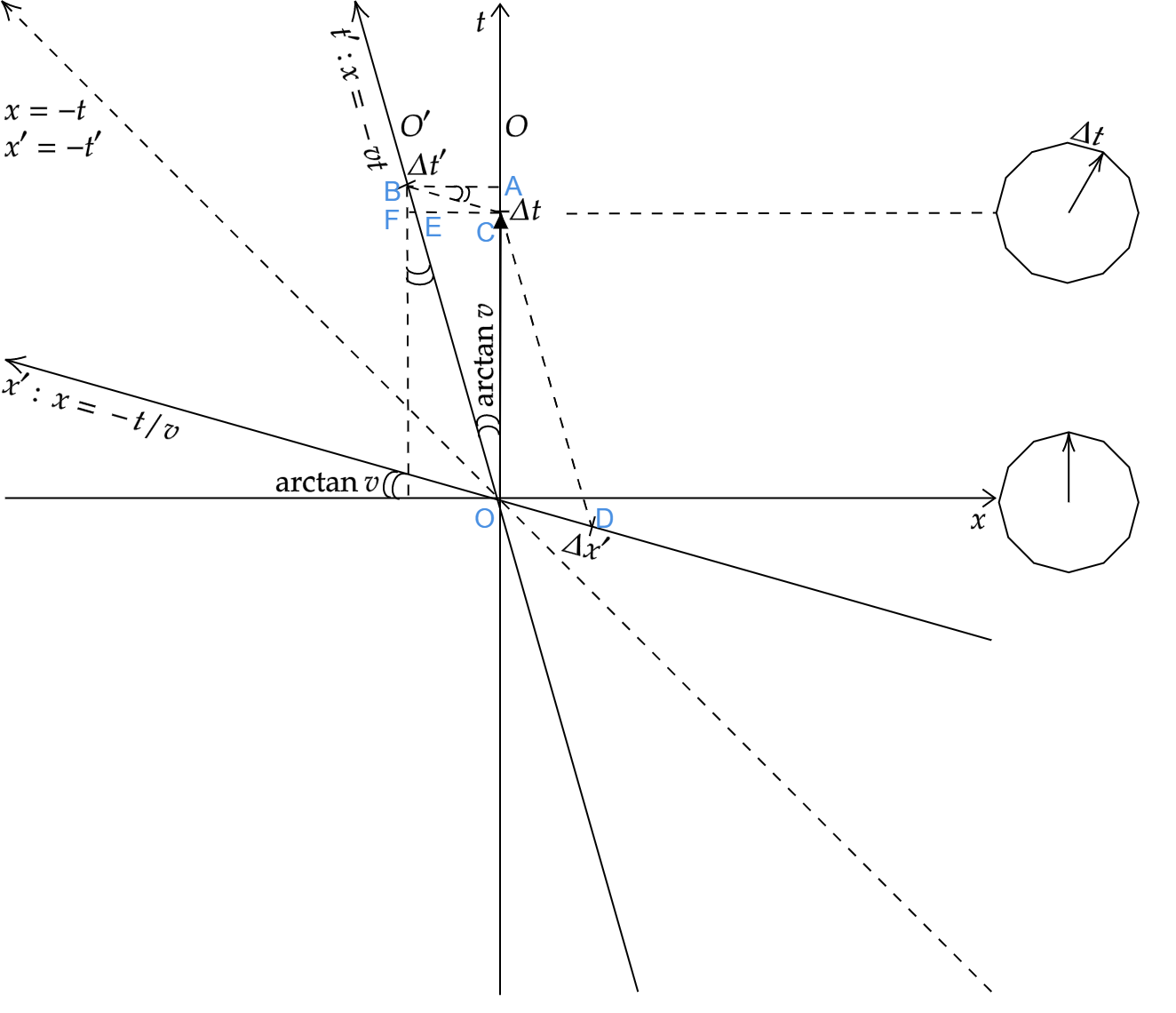
I wanted to visualize time dilation through Minkowski diagrams but I ended up in a fiasco. Let me show how. Consider two observers $O$ and $O^\prime$ at rest and moving with velocity $-v$ with respect to a clock, respectively. Suppose a one-dimensional boost that transforms the coordinate frame from $x^\alpha$ (in which $O$ is at rest) to the coordinate frame $x^{\prime\alpha}$ (in which $O^\prime$ is at rest) as shown in the figure. Note that I have taken the speed of light $c=1$.
 Let me prove time dilation first.
Let me prove time dilation first.
Proving $\Delta x^\prime=v\Delta t^\prime$ (can also be proved by boost transformation):
In $\unicode{0x22BF} AOB,\ OB=\Delta t^\prime\Rightarrow AB=\Delta t^\prime \sin(\arctan v)$.
In $\unicode{0x22BF} ABC,\ AB=\Delta t^\prime \sin(\arctan v),\ BC\parallel x^\prime \text{axis} \Rightarrow BC=\Delta t^\prime \displaystyle\frac{\sin(\arctan v)}{\cos(\arctan v)}=\Delta t^\prime \tan(\arctan v)=v\Delta t^\prime$.
In parallelogram $ABOD,\ OD\equiv \Delta x^\prime = BC$, so that $$\Delta x^\prime = v\Delta t^\prime \tag{1} \label{time_dilation_length}$$
Invariance of proper time $d\tau$ for both observers gives the common result of time dilation as follows: \begin{align*} d\tau^2&=d\tau^{\prime 2}\\ \Delta t^2 &= \Delta t^{\prime 2} - \Delta x^{\prime 2}\\ \Delta t^2 &= \Delta t^{\prime 2} - v^2\Delta t^{\prime 2}&(\because \Delta x^\prime = vdt^\prime)\\ \Delta t^2 &= (1 - v^2)\Delta t^{\prime 2}\\ \Delta t^\prime &= \frac{\Delta t}{\sqrt{1 - v^2}}\\ \Delta t^\prime &= \gamma\Delta t\tag{2} \label{time_dilation} \end{align*} Now comes the ambiguity I ended up in:
\begin{align*} \Delta t^\prime &= OE+EB\\ \text{where }OE &= \Delta t/\cos(\arctan v) = \Delta t\sqrt{1+v^2}&(\text{In }\unicode{0x22BF} EOC)\\ EB &= FB/\cos(\arctan v)&(\text{In }\unicode{0x22BF} FEB)\\ FB &= BC\sin(\arctan v)&(\text{In }\unicode{0x22BF} FBC)\\ \Rightarrow EB &= BC\tan(\arctan v)= vBC= v\Delta x^\prime=v^2\Delta t^\prime &(\because\eqref{time_dilation_length})\\ \text{so that}\quad \Delta t^\prime &= \Delta t\sqrt{1+v^2} + v^2\Delta t^\prime \\ (1-v^2)\Delta t^\prime &= \Delta t\sqrt{1+v^2}\\ \text{If I apply equation \eqref{time_dilation}, I get}\ \sqrt{1-v^2} &= \sqrt{1+v^2} \end{align*} What is going wrong?
