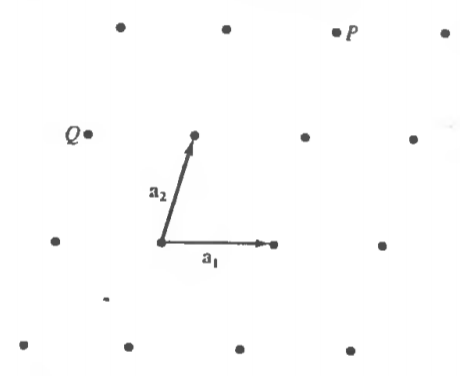
But, in general, what does it means by "vectors"?
It is the standard definition of vectors, in this case two dimensional vectors (very naively pairs of real numbers, or less naively, objects that suitably transform under rotations).
Where are these vectors placed and which points do they connect?
Well, conventionally the origin is placed on a random lattice point (you chose which one) and the lattice vectors connect that site to all the others.
Is the previous definition true for vectors applied in any point of
space and with any length?
Maybe this question is not so clear to me. I think you are asking: "can I put the origin of my system anywhere, must I place it on a lattice point?" and "can lattice vectors have arbitrary lengths once I fix the length of a1, a2?".
Well, in principle the origin can be anywhere, but it would be really confusing to place it anywhere but in a lattice point. So since the position of the origin is completely arbitrary, it is worth choosing a convention, which is to place it on a site. Finally, the lengths of lattice vectors are not arbitrary at all, once you fix a1 and a2. I can give you a simple expression for the lengths: let $a_1^2=\bf{a}_1 \cdot \bf{a}_1$, $a_2^2 = \bf{a}_2 \cdot \bf{a}_2$, $b = \bf{a}_1 \cdot \bf{a}_2 $. A generic lattice vector is $\bf{R} = m \bf{a}_1 + n \bf{a}_2$, where $m,n$ are integers, so its length is $R = \sqrt{\bf{R}\cdot\bf{R}}$:
$$
R = \sqrt{a_1^2 m^2 + a_2^2 n^2 + 2b m n},
$$
so R cannot assume all the possible real values.
PS: my analysis is for 2-dimensional lattices, but generalizing is straightforward.