Classical Particle Disintegration (Landau Lifschitz)

Question

So I'm reading Landau's mechanics books and there was a question for the angular distribution of the resulting particles in the lab system (p.44 Q2 Chpt 4). It says when $v_0>V$, it is given by

$$\frac{1}{2}\sin\theta\,d\theta\left(2\frac{V}{v_0}\cos\theta+\frac{1+\frac{V^2}{v_0^2}\cos2\theta}{\sqrt{1-\frac{V^2}{v_0^2}\sin^2\theta}}\right)$$ I managed to find this but what I don't understand is why for the case that $v_0<V$ the difference instead is taken. In the book it's explained by saying that as $\theta$ increases one value of $\theta_0$ increases and the other decreases, referring to this equation $$\cos\theta_0=-\frac{V}{v_0}\sin^2\theta\pm\cos\theta\sqrt{1-\frac{V^2}{v_0^2}\sin^2\theta}$$ Where $v_0$ is the velocity of the particle in the Centre of Mass Frame, $V$ is the velocity of the Centre of Mass Frame, $\theta_0$ is the angle between the direction the particle travels velocity of the centre of mass frame when measured in the centre of mass frame, and $\theta$ is the angle between the direction the particle travels and the velocity of the centre of mass frame when measured in the Lab frame.

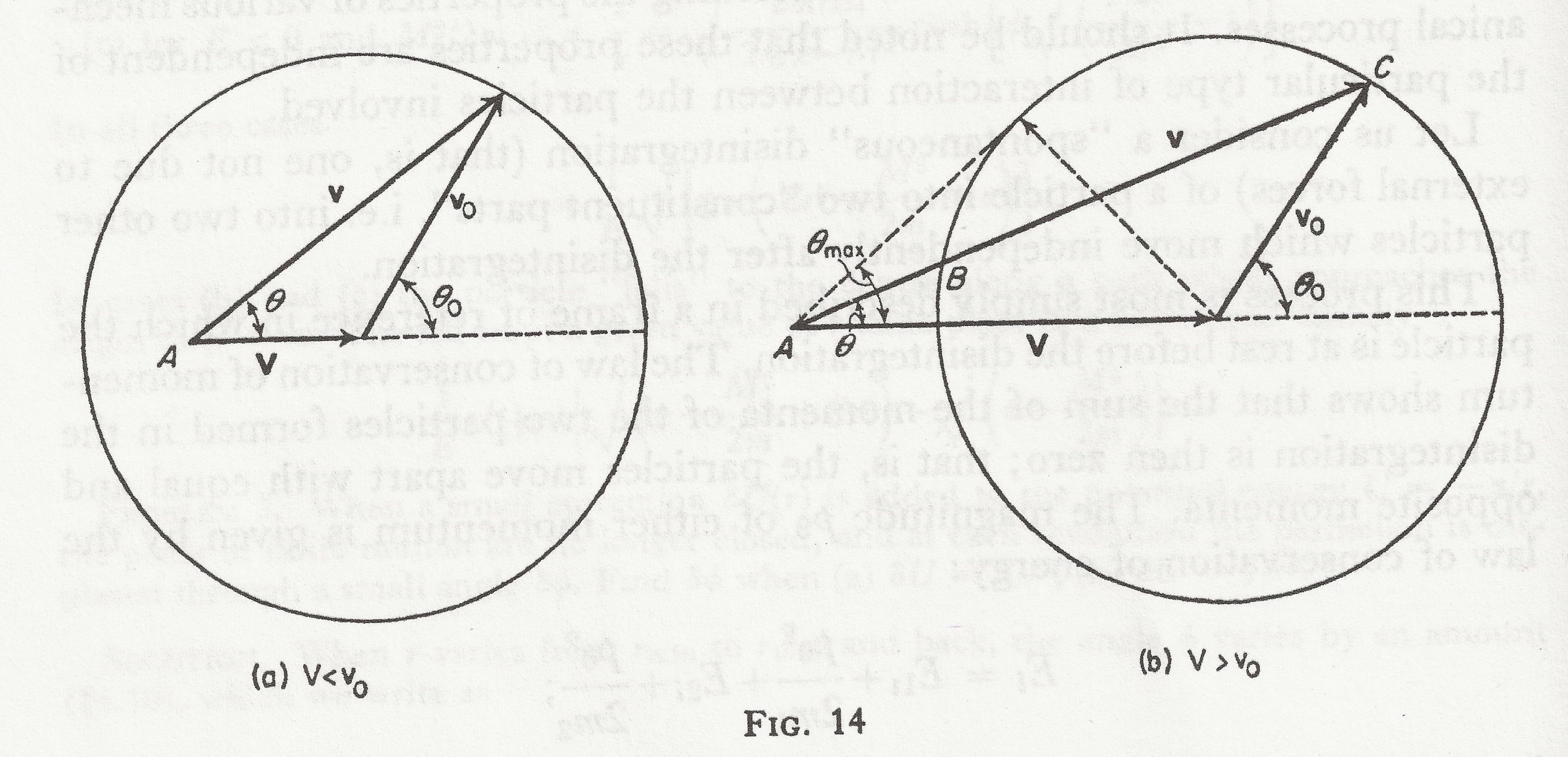
However, isn't the behaviour that Landau describes still present even when $V<v_0$? I can't figure out what the difference between the two cases is other than when $V>v_0$ there exists some discontinuities in the graph of the function, but that doesn't explain why the difference is taken instead. Could someone please explain to my why when $V>v_0$ the difference is taken instead? Thanks!

Answer 1

The angular distribution in the L frame is the sum of contributions from the two possible $\theta_o$ angles. As $\theta$ increases, one of the $\theta_o$ angles decrease meaning $d(cos\theta_o)$ is negative. So minus the minus to get a positive.
A bit like integration gives an area below the x-axis as negative. If $A_1$ is the integral above the x-axis and $A_2$ is the integral below the x-axis, the total area is $A_1$ - $A_2$