3
$\begingroup$

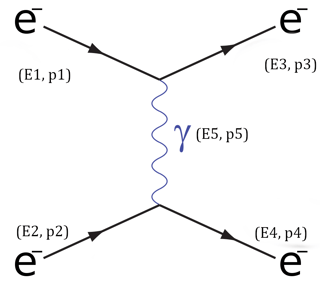
I am trying to understand the argument for why internal lines in a Feynman diagram are virtual particles that do not obey the energy-momentum relation.

For the specific case of the following Feynman diagram, I was given the argument that the energy and momentum of the photon are over-constrained, hence the photon violates one constraint ie. the energy-momentum relation.

Electron Scattering Feynman diagram

However I fail to see why this is the case. This is my understanding: $E_1$, $E_2$, $p_1$, and $p_2$ are known as they are experimental parameters, so this leaves 6 unknowns (energy and momentum of the final states and of the photon). If I assume all of them follow the energy-momentum relation then this is reduced to 3 unknowns. Energy is conserved in each vertex which gives the relation between the remaining unknowns leaving 1 degree of freedom.

Is there any constraint I am missing? The only other reason I can think of is that the energies of the two final electrons are experimentally found not to depend on each other, meaning that this requires 2 degrees of freedom instead of one. Is this maybe the case?

Improve this question
$\endgroup$
1

1 Answer 1

Reset to default
2
$\begingroup$

Energy and momentum are conserved on internal lines, which means that the mass shell condition cannot hold (as you can establish with a simple calculation). $$ m^2\ne p^2 = E^2 - \mathbf p^2$$ The question of whether internal lines represent "virtual" particles is primarily philosophical. Feynman for one regarded them as real particles which are not directly observed.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Maybe you mean are not conserved ? Else, your answer is impossible ot understand. $\endgroup$
    – Mathieu Krisztian
    Commented Mar 20, 2022 at 13:50

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.