Given a Mach number, how would I go about determining the geometry of the associated Mach cone? Apologies, I'm not too well versed in physics.
$\begingroup$
$\endgroup$
1
-
$\begingroup$ See [NASA website][1], it's a definition. Notice that the sound speed needed to be fixed. grc.nasa.gov/WWW/k-12/airplane/machang.html $\endgroup$– ShoutOutAndCalculateCommented Apr 24, 2020 at 16:56
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
2
While @R.W.Bird's answer is absolutely correct, I will complement it with a more graphical explanation.
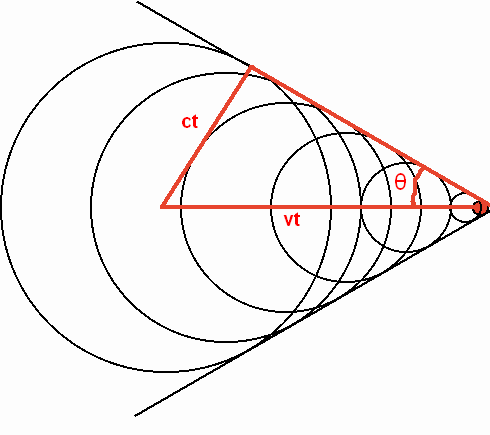
Consider an airplane flying with speed $v$, and the spherical sound waves spreading from the airplane with the speed of sound $c$.
You see, when $v>c$ (i.e. when the airplane is faster than sound), then the sound waves form to a cone with the airplane at the tip of the cone.
During a time interval $t$ the airplane flies a distance $vt$. In the same time a spherical sound wave grows to radius $ct$. To calculate the cone angle $\theta$ consider the red right-angled triangle. From the geometric definition of the sine function you get $$\sin\theta=\frac{ct}{vt} =\frac{c}{v}. \tag{1}$$
On the other hand the Mach number is defined as $$M=\frac{v}{c}. \tag{2}$$
By comparing (1) and (2) you can conclude $$\sin\theta=\frac{1}{M}$$
answered Apr 24, 2020 at 19:45
-
$\begingroup$ How to prove that there is rectangular triangle (the red one)? Regards RJ $\endgroup$– rafjakCommented May 1, 2022 at 12:51
-
$\begingroup$ The right angle occurs because, for sound waves, the direction of propagation is perpendicular to the wavefronts. $\endgroup$ Commented May 1, 2022 at 13:17
$\begingroup$
$\endgroup$
A wave in the shock front moves away from its point of origin at the speed of sound. The plane moves away from that point at the Mach number times the speed of sound. In a given time the distance traveled by the plane forms the hypotenuse of a triangle with the distance traveled by the sound forming the side opposite the apex of the cone. One over the Mach number gives the sine of the angle at the apex (measured from the path of the plane).
