$q$ sets up an E-field, expanding outward at $c$, 'carried' by photons. The quantity of photons is finite - then, the longer the distance $r$ from $q$, the more 'diluted' the photons are about the spherical surface they span.
Eventually, $r$ is large enough that mutually closest photons are light years apart. Then, if E-field is, in fact, not mostly zero at that radius - what is transmitting the E-field to matter light-years between the distant photons? Whatever it is, it must be able to influence an arbitrary amount of matter in the 100 light years spanned between the two source carriers (see below) - simultaneously, even if the matter itself is separated by light-years. This implies superluminal speed of information transmission through space, violating relativity.
"It's a wave, not a particle" - the problem persists; can one wave simultaneously exert influence on quadrillions of particles (and separated by light years)? A wave's energy is finite, and finitely-reducible, and its speed is $c$, so this is impossible.
While the linked answer and others suggest photons are the 'carriers", I'm unsure whether there's a concensus - but whatever the carrier, as long as finite, above holds. All said; how is the electric field set up by a source charge not mostly zero very far away?
Diagram:
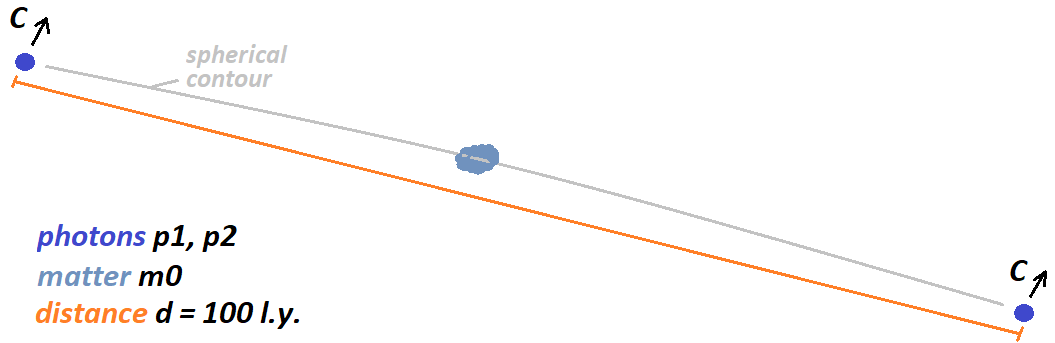
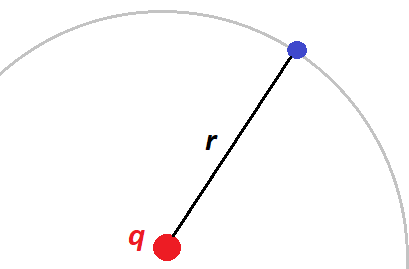
Update: I'll refine the inquiry to minimize any further ambiguity.
The root premise of the inquiry is localization. Whatever the 'thing' - a field, a particle, a wave - it isn't "omnipresent", and its interaction with matter is bound within a finite region of spacetime. This includes space itself; while possibly infinite, it isn't 'same' everywhere - its curvature isn't fixed, and matter behaves differently within its different subregions. Anything that isn't uniformly distributed over all of spacetime obeys this logic; e.g., in some models, electrons have an infinitely extending position probability distribution - but probability is unit-normed, and thus isn't uniform over all of spacetime (else $P = \infty$).
Whether as waves or as particles, photons (or whatever the carrier) must likewise obey the above. The "balls" in the diagrams represent localization, not particles.
Re: G. Smith's answer.
My inquiry concerns the E-field due to a charge. I particularly fail to make any sense of:
Finally, each charge does not have its own field. There is only only one quantum EM field pervading the universe, and all photons in the universe are quanta of this one field.
Whether true or not, somehow it's acceptable to casually introduce such a "non-mainstream" idea to a followup of a followup question. I digress; it seems more a matter of relevance than truth - again, I ask about the E-field due to a charge. If we agree that E-fields vary through space, and that one definite source of this variation is a net-charge, and that the transmission of this variation involves finitely many carriers, then all below "Update" applies.
The only scenario I see in which my arguments break down is if interpreting the quoted excerpt a certain way: that the EM-field is like the gravitational field - permeating all of spacetime, and affected by charges just like space is affected by matter. Then I've been heavily misled by both this and this answer to ever fail to bring this up (and to explicitly suggest photons are said carriers), likewise by my 'respected' university physics & engineering textbooks or professors. If this is the truth, I'll accept it, but there's sure a point to be made on not keeping such facts as "side ideas".
Even so, this puts in question the relevance of photons in context of E-fields (as it's been explained by the "answers"), but that'll be a separate question.
(Lastly, before there's again confusion, I clarify that the localization argument pertains to a field, set up by a localized entity, that's transmitted by finite carriers, which differs in context space & mass, or gravity)
