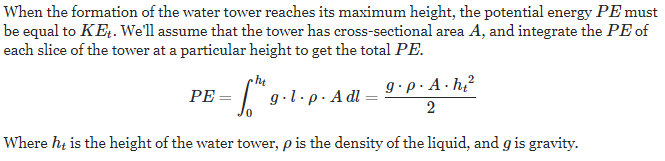Hello I was reading another question asked by zach466920, and when he was trying to calculate the total GPE of a water 'tower', he used this explanation:
He basically used integration to calculate the total GPE of a cylinder by dividing it into disks of infinitesimal thickness, and summing those GPEs. However, another comment said:
Well the integration part is not necessary you know ... You only have to consider the center of gravity of the water pillar when calculating the GPE as the volume is uniform
I do not get this explanation, rather I think the integration method makes sense. Which one is correct?
The post is from How does a ball cause a splash? (With the relevant math)