1
$\begingroup$

l just encountered a problem that is about a string in harmonic motion. The question states that the cord is elastic and gives a table like this

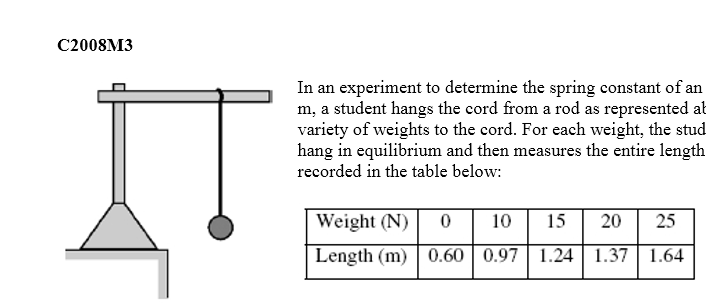
enter image description here

The question didn't states that the cord changes, only the mass and the length are the variables, however, l found out that for each Weight, the value of string constant is slightly different from each other, why? Isn't k only depends on the material of the spring, if the spring did not change, k should be constant all the time.

If spring constant is not a constant value, why it is called spring constant

What caused k varies?

Improve this question
$\endgroup$
4
  • $\begingroup$ How about experimental error. $\endgroup$
    – Bill Watts
    Commented Mar 8, 2020 at 7:39
  • $\begingroup$ but what kind of errors can that be? l thought our technology is powerful enough to have high accuracy? $\endgroup$
    – Sherri
    Commented Mar 8, 2020 at 7:53
  • $\begingroup$ They do not specify the measurement device, so we can't know what it's accuracy is. But even the mighty Kibble Balance, a true testament of engineering, has errors. $\endgroup$
    – Cort Ammon
    Commented Mar 8, 2020 at 7:58
  • $\begingroup$ Some materials have strain hardening or strain softening, where k changes with length, but in those cases you have a trend. The clue here is the k varies up and down with the measurement, so the variation is most probably experimental error. The problem gives no clue as how the student is doing his measurements. $\endgroup$
    – Bill Watts
    Commented Mar 8, 2020 at 8:06

3 Answers 3

Reset to default
1
$\begingroup$

Yes, the force a spring (or generally any material obeying Hooke's law) exerts varies linearly with the elongation, as long as it is sufficiently small . Looking at a typical stress/strain diagram, for materials it is accepted that Hooke's law becomes invalid around a strain of 1%. The reason your constant varies is because of supposed measurement errors though.

Improve this answer
$\endgroup$
2
  • $\begingroup$ so, you mean that k is not an absolute constant value, instead, it is an average constant value based on many experimental results ? $\endgroup$
    – Sherri
    Commented Mar 8, 2020 at 7:55
  • $\begingroup$ The spring itself has an unchanging constant, it's just that you can only measure the elongation and the mass of the body attached so well. To get a very precise idea about the spring constant you would have to take a lot of measurements and average them. $\endgroup$
    – Johnny
    Commented Mar 8, 2020 at 8:00
1
$\begingroup$

This is a nice example of the difference between Physics and Mathematics.

The mathematical model says that, for a limited range of elongations, modulus of the force and displacements are proportional. However, real measurements deviate from the exact Hooke's law for two reasons.

The first is due to the unavoidable perturbations connected to each measurement. The exact sources of such perturbations may be difficult to identify uniquely and depend on the details of the protocol, on the hardware, on the external conditions, just to mention a few causes. Usually they introduce a kind of random variation of the individual measurements which can be analyzed and controlled with statistical methods and this is what people call the statistical error. In principle, it can be systematically reduced by increasing the number of measurements.

A second class of deviations originates from non-random sources. Collectively, and on the base of the effect on results, this class of deviation is called systematic error. Once again there are many different possible causes of systematic error. In the case of a spring constant measurement I would list:

  • bad calibration of the instruments;
  • action of a physical mechanism not present in the model like, for example, the presence of a non-linear regime or a dependence of the force constant on temperature with experiments performed in conditions of systematic increase or decrease of temperature;
  • experimental points coming from completely different experiments and measurement methods.

Therefore, you see that the world, out of the textbooks is quite complex. The fascinating thing is that, notwithstanding such difficulties, it is still possible to reduce all the sources of uncertainty and to build predictive and accurate theories out of noisy experimental data.

The typical problem of the experimental data is how to be able to assign a probability that a given set of noisy data is compatible with a physical hypothesis. I assume that the problem, with its quite large deviation from linearity, was proposed as an exercise related to error analysis.

Improve this answer
$\endgroup$
2
  • $\begingroup$ well, it is possible for us to get accurate data in the future? Or those small errors is always unavoidable and the only thing we could do I s just to minimize it? $\endgroup$
    – Sherri
    Commented Mar 8, 2020 at 17:49
  • $\begingroup$ @Sherri A measurement with zero uncertainty has to be considered an asymptotic aim. Probably one of the most accurate quantities ever measured is the intrisic electronic magnetic moment, which is known with a relative precision of about $1$ part in $10^{12}$. Very small, but not zero. $\endgroup$
    – GiorgioP-DoomsdayClockIsAt-90
    Commented Mar 9, 2020 at 7:14
0
$\begingroup$

Under the elastic limit, an object under tension experiences an increment/decrement of length $\Delta x$ (with initial length being $x_0$)and this causes a restoring force,$F$, to act, which is given by:

$$F = \left ( \frac {YA}{x_0} \right)\Delta x = k\Delta x$$

$$\Rightarrow k=\frac {YA}{x_0}$$

Here, $Y$ is the Young's modulus of elasticity (a constant for a given material), and $A$ is the cross sectional area of the material.

Clearly, you can see that $k$ varies from one material to another. Also it varies from one object to other (for the same material).

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.