What does it mean that an expression satisfies Poisson's equation?

Question

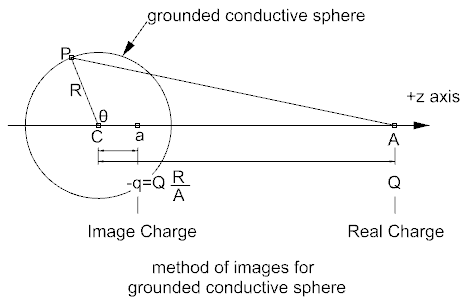
The criteria for an expression to determine the field and charge distribution when using the method of images is that:

1. the expression must satisfy Poisson's equation, which is, $\nabla^2\phi=-\frac{\rho}{\mathcal{E}_0}$.
2. the potential must approach 0 as r approaches infinity and
3. the potential from the image(s) and real charge must sum to 0 on the grounded sphere or a constant value on the Non-grounded sphere

I am using the method of images to solve for the field outside of and charge distribution on a sphere. I understand #2 and #3, but I don't quite understand #1. When I solve this for the field and the charge distribution I get:

$\phi=\frac{1}{4\pi\mathcal{E}_0}\left[\frac{Q}{\sqrt{R^2+A^2-2RAcos{\theta}}}-\frac{q_i}{\sqrt{R^2+a^2-2Racos{\theta}}}\right]=0$ on the surface

$\ \sigma=-\mathcal{E}_0\frac{\partial\phi}{\partial r}=\fbox{$\frac{-Q\left(A^2-R^2\right)}{4\pi R}\left[\frac{1}{\left(R^2+A^2-2RAcos{\theta}\right)^{3/2}}\right]$} $

I have verified by integrating to get the total charge on the outside of the sphere which should be $-Q\frac{R}{A}$ as in the Figure and it is.

I haven't put down more of the steps because that’s not the main point I am asking about.

I of course realize that the expression that I use has the form of Poisson's equation. I also realize that the problems are electrostatic and therefore have no extra energy from displacement currents and that they relate only to potential fields. What explicitly does #1 mean? Just that the form must match, and that it is a potential, and that there is no extra charge or energy from somewhere?

Answer 1

First note that the potential of the real charges - the external charge and the surface charge on the sphere - will obey Poisson's equation, because real charges obey Maxwell's equations! When using the method of images you don't change the distribution of charge or the potential outside the sphere, so Poisson's equation is still obeyed in that region.

It is fairly obvious that you don't change the charge distribution outside the sphere -- you leave the single external charge alone -- but less obvious that you leave the potential unchanged. This relies on a uniqueness theorem, which is proven straightforwardly in Griffiths' Electrodynamics text book and probably others.

The uniqueness theorem says that if you specify the charge distribution in the region of interest, and you also specify the potential on the boundaries, then this fixes the potential everywhere in the region. (Slightly subtle point: Your region of interest is the space between the surface of the sphere and infinity. It does not contain its boundaries, but the boundary conditions still apply. Your boundary conditions are that $V=V_0$ on the surface of the sphere and $V=0$ at infinity.)

Once you have the potential outside the sphere, you can combine this with what you already know about the potential inside ($V_0$, because there is no electric field in a conductor) to determine the surface charge distribution.