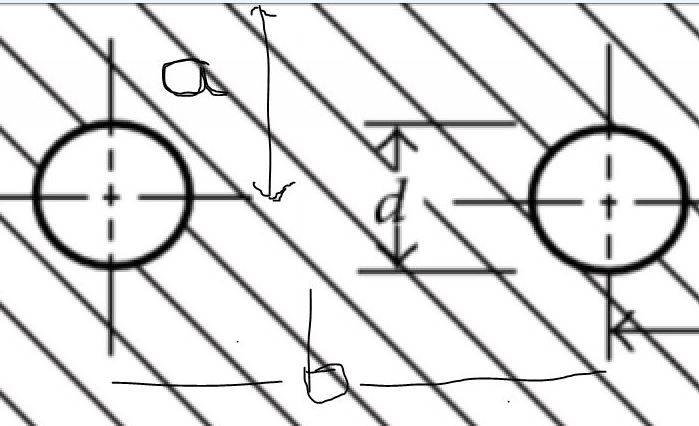
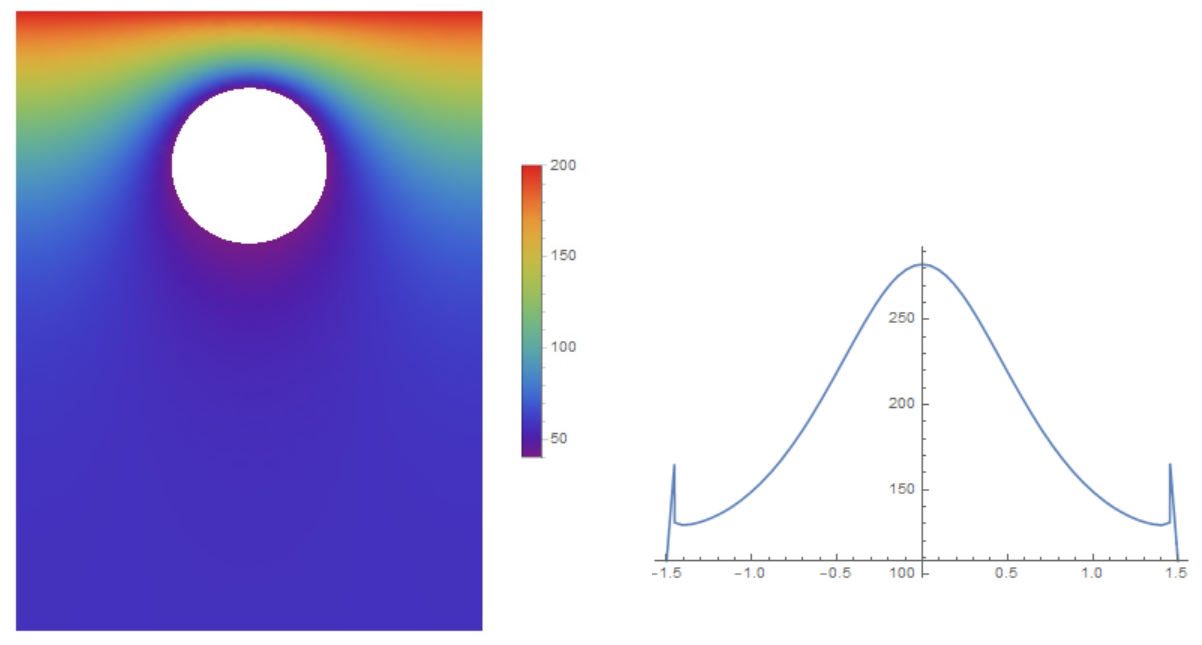
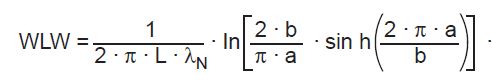2
$\begingroup$

This is a section of cooling channels in injection moulding tools.

enter image description here enter image description here

I have tried to calculate the thermal resistance in this section with 2 methods, one analytical and one numerical.

  1. analytical solution:

I have calculated the thermal resistance by this equation

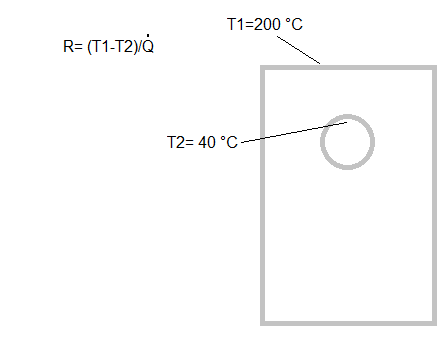
enter image description here

  1. numerical there I have the difficulties, I vary the T1 and T2 and I read out the heat flows from ANSYS. What I can't understand is, which heat flow should I take (on the wall? or on the channel? in the middle?), because the heat flow is not constant in the part.

enter image description here

Many thanks

Improve this question
$\endgroup$
10
  • 1
    $\begingroup$ What is painted in color? $\endgroup$
    – Alex Trounev
    Commented Jan 14, 2020 at 0:17
  • $\begingroup$ This is a transient problem, right? $\endgroup$
    – Chet Miller
    Commented Jan 14, 2020 at 0:49
  • $\begingroup$ @ChetMiller Yes, it's a transit analysis. $\endgroup$
    – Zouhir Bensebban
    Commented Jan 14, 2020 at 10:28
  • $\begingroup$ @AlexTrounev do you mean the last painting? $\endgroup$
    – Zouhir Bensebban
    Commented Jan 14, 2020 at 10:29
  • $\begingroup$ @ZouhirBensebban There is one color drawing - something with many holes. Is this a tool section? $\endgroup$
    – Alex Trounev
    Commented Jan 14, 2020 at 10:50

1 Answer 1

Reset to default
0
$\begingroup$

A numerical solution to the problem $\nabla ^2 T=0$ with boundary conditions $T=T1=200C$ at the upper boundary and $T=T2=40C$ at the boundary of the hole is shown in Figure 1 on the left, and the heat flux at the upper boundary $\dot {q}=-\vec {n}.\lambda \nabla T$ at $\lambda = 1$ on the right. To find the thermal resistance, we calculate the integral $$\dot {Q}=\int_0^b \dot {q}dx$$ Figure 1

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thank you very much for your helpful answer. how did you get this curve? through Matlab? i first have to simplify dot {q} to calculate this integral $\endgroup$
    – Zouhir Bensebban
    Commented Jan 15, 2020 at 10:03
  • $\begingroup$ I used FEM and Mathematica 12. $\endgroup$
    – Alex Trounev
    Commented Jan 15, 2020 at 11:39
  • $\begingroup$ Hello, Alex, is the integral with the separation of the variable solvable? I have followed the steps in the book "Fundamentals of Heat and Mass Transfer by Theodore L. Bergman" on pages 211-214, but I am confused when it comes to inserting constants. $\endgroup$
    – Zouhir Bensebban
    Commented Jun 11, 2020 at 8:33

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.