Boyle's law question

Question

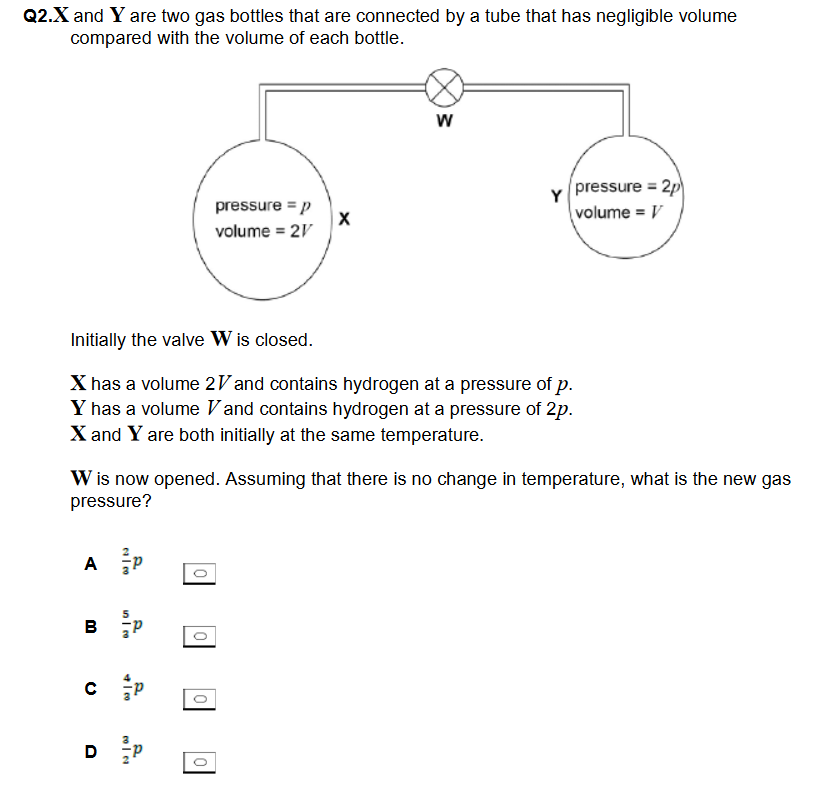
X and Y are two gas bottles that are connected by a tube that has negligible volume compared with the volume of each bottle. Initially the valve W is closed. X has a volume 2V and contains hydrogen at a pressure of p. Y has a volume V and contains hydrogen at a pressure of 2p. X and Y are both initially at the same temperature. W is now opened. Assuming that there is no change in temperature, what is the new gas pressure?

I used Boyle's law by saying both container have the constant 2Vp=constant as they are both at the same temperature . When the valve is opened I said the new volume is 3V and I then equated to find the new pressure by saying: where P is new pressure , 3VP=2Vp ..so P=2p/3 . However the answer was 4p/3?

Answer 1

I want to answer this in case someone uses this in the future, even though this is two years late. Once the valve opens, the gas will be equally spread between both bottles. This means that each bottle will have $1.5 \ V$. We know that $PV = kT$. Assuming we take $X$ as the reference (even though $Y$ works as well), $P$ has decreased by $\frac{1}{4}$ which means volume must increase by $\frac{1}{4}$ considering $T$ is constant and $PV$ are inversely proportional which means $P$ is $\frac{4}{3}$. I hope that helps.