3
$\begingroup$

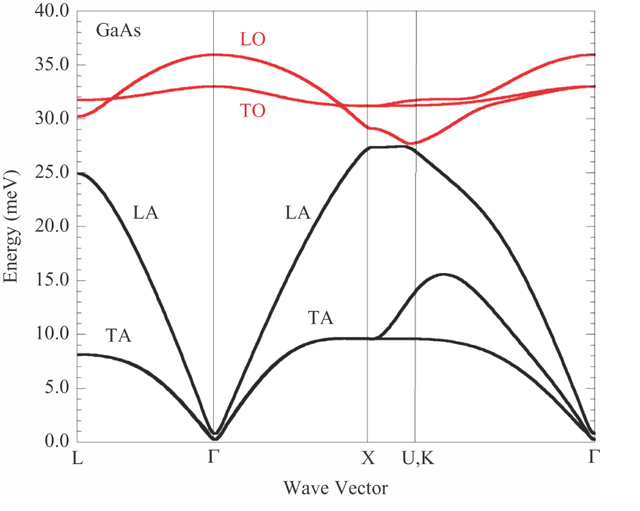
I have a beginner question on solid state physics:

GaAs has phonon dispersion spectrum that looks so:

enter image description here

the horizontal axis parametrizes the wave numer values $k$, therefore it seems plasible that the direction axis of $ \vec{k} $ is fixed in the whole diagram. In other words $k=\vert \vec{k} \vert$.

On the other hand $\Gamma, X, U, K, L$ represent different directions in the $k$-space: see here

Therefore I'm a bit confused what is parametrized by horizontal axis.

Or does every connected path in the diagram correspond to a special direction $\frac{\vec{k}} {\vert \vec{k} \vert}$ for example $X, K$ or $L$? If it is so, then which path belongs to which direction $\frac{\vec{k}} {\vert \vec{k} \vert}$?

Could somebody explain how to read/understand what is going on at the parametrization of the $k$-axis? What do the big letters on the bottom say? $L - \Gamma - X - U,K - \Gamma$.

Does it mean that firstly we start at point $L$ of the $\vec{k}$-space and go along the straight line to $\Gamma =(0,0,0)$ (thus along the direction $\vec{k}_{\Gamma}- \vec{k}_L$), then - after arriving at $\Gamma$, we change the direction to $\vec{k}_X -\vec{k}_{\Gamma}$ an go along it until we arrive point $X$ and so on?

What happens between $X$ and $U,K$?

Improve this question
$\endgroup$
8
  • $\begingroup$ Between, say, the [100] and [111] directions, you have wave vectors of [1xx] with x increasing from 0 to 1 along the segment joining those two particular directions. $\endgroup$
    – Jon Custer
    Commented Oct 27, 2019 at 3:30
  • $\begingroup$ ok, so on every partial intervall between two big letter say A and B on the $k$-axis we choose as direction $\frac{\vec{k}} {\vert \vec{k} \vert}$ the difference vector of direction vectors of $A$ and $B$,right? $\endgroup$
    – user267839
    Commented Oct 27, 2019 at 22:46
  • $\begingroup$ The graph shows dispersion along high-symmetry directions in the Brillouin zone. There are many other points in k-space, with other directions. Densities of states are derived from sampling all of the Brillouin zone (sampling an irreducible wedge is enough). $\endgroup$
    – user137289
    Commented Oct 27, 2019 at 23:17
  • $\begingroup$ yes yes, that's clear. The point is for example if well consider the partial intervall between $X$ and $U$ in the middle, in which direction in the $k$-space we are going there? As you said $X$ and $U$ represent certain high-symmetry directions in $k$-cpace, let call them $\vec{k}_X$ and $\vec{k}_U$. My intuitive suspicion is that as long as we are going along path X - U on the $k$-axis, we go in direction $\vec{k}_U- \vec{k}_X$. Is this the correct interpretation or did I misunderstood the point? $\endgroup$
    – user267839
    Commented Oct 27, 2019 at 23:29
  • 1
    $\begingroup$ The cuts are along a specific direction. E.g. $\Gamma$ - $X$ is along the direction from $\Gamma$ towards $X$. etc. $\endgroup$
    – Nathan Holman
    Commented Nov 10, 2019 at 5:49

1 Answer 1

Reset to default
1
+50
$\begingroup$

The purpose of drawing the dispersion relation like this is to facilitate the visualization of a 3-dimensional function in a 2-dimensional plot by traveling in lines along high symmetry points. Traveling along 2 points, say from $\Gamma$ to $X$ means to travel from k value [0,0,0] to k value [1,0,0] with points of the form [x,0,0] in between.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.