Misunderstanding the calculation of the potential for a system of 2 concentric shells

Question

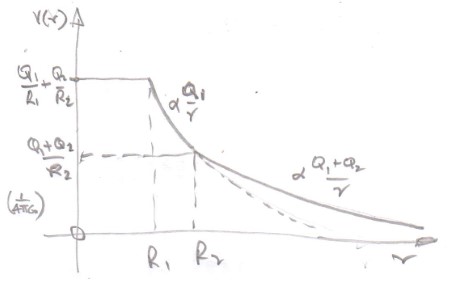
Let's say we have 2 concentric shells. The little one has a charge $Q_1$ and a radius $R_1$ and the greater one has a charge $Q_2$ and a radius $R_2$ with $R_2$ > $R_1$.

We want to calculate the potentials so :

1) $r > R_2 :$

$V(r) = \frac{(Q_1 + Q_2)}{4\pi \epsilon_0}\frac{1}{r}$

$V(R_2) = \frac{(Q_1 + Q_2)}{4\pi \epsilon_0}\frac{1}{R_2}$

2) $R_1 < r < R_2 :$

This is where I don't understand. I would say that it's the difference of potential $V(R_2) - V(R_1)$

I would calculate : $V(r) = \frac{(Q_1 + Q_2)}{4\pi \epsilon_0}\frac{1}{R_2} - \frac{(Q_1)}{4\pi \epsilon_0}\frac{1}{R_1}$

However we have : $\frac{(Q_1)}{4\pi \epsilon_0}\frac{1}{r} + \frac{(Q_2)}{4\pi \epsilon_0}\frac{1}{R_2}$

3) $r < R_1 :$

$V(r) = 0$ (no charges inside)

I suppose that the following potential comes from the fact that to bring a charge from $+\infty$ we have to "fight against" the contributions of the charge $Q_2$ uniformly distributed on the sphere of radius $R_2$ and of the charge $Q_1$ uniformly distributed on the sphere of radius $R_1$ ?

$V(R_1) = \frac{(Q_1)}{4\pi \epsilon_0}\frac{1}{R_1} + \frac{(Q_2)}{4\pi \epsilon_0}\frac{1}{R_2}$

But why don't we have (following the logic of the case $r > R_2$) : $V(R_1) = \frac{(Q_1)}{4\pi \epsilon_0}\frac{1}{R_1} + \frac{(Q_1 + Q_2)}{4\pi \epsilon_0}\frac{1}{R_2}$ ?

Answer 1

3) $r < R_1 :$ $V(r) = 0$ (no charges inside)

Is an incorrect statement.
Inside the inner shell the electric field is zero and the potential is constant.
It can be zero if you define it to be so, however, from what you have written for case 1 you have taken the zero of potential to be at infinity.

The electric field outside the outer shell is due to charge $Q_1+Q_2$, that between the two shells is due to charge $Q_1$.

The potential outside of the outer shell is $V(r) = \dfrac{(Q_1 + Q_2)}{4\pi \epsilon_0}\dfrac{1}{r}$ and so at the outer shell it is $V(R_2) = \dfrac{(Q_1 + Q_2)}{4\pi \epsilon_0}\dfrac{1}{R_2}$

The potential of the inner shell relative to the outer shell is is $ \dfrac{Q_1}{4\pi \epsilon_0}\left (\dfrac{1}{R_1}-\dfrac{1}{R_2}\right )$

So the potential of the inner shell is $V(R_1) = \dfrac{(Q_1 + Q_2)}{4\pi \epsilon_0}\dfrac{1}{R_2}+\dfrac{Q_1}{4\pi \epsilon_0}\left (\dfrac{1}{R_1}-\dfrac{1}{R_2}\right ) = \dfrac{1}{4\pi \epsilon_0}\left (\dfrac{Q_1}{R_1}+\dfrac{Q_2}{R_2}\right )$

If the charges are positive the potentials look something like this.