1)
Conservation of momentum in the absence of external forces is not valid in non-inertial frames. This is because Newton's second law, in which $\vec{F}$ stands for the net external physical (not including pseudo forces) force, is valid only in inertial frames.
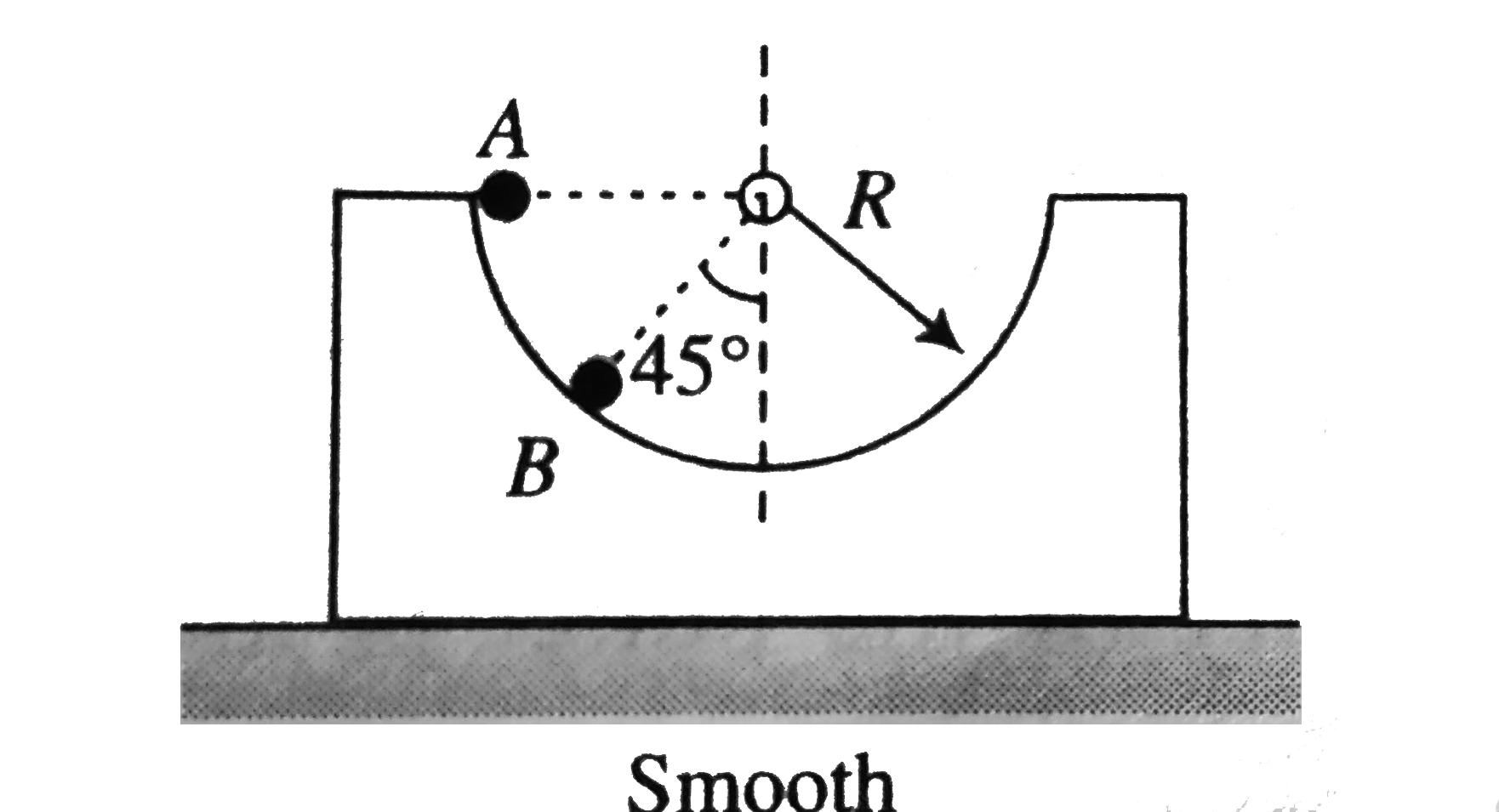
Next, you can solve this by considering the (block + wedge) as a combined system in the ground (inertial) frame. In the solution provided $v_1$ is the speed of the block with respect to the wedge and $v$ is the speed of the wedge with respect to the ground.
$\vec{V} = (v_1 cos(45)-v) \hat{i} + v_1sin(45) \hat{j}$ is the velocity of the block with respect to the ground (Can you see why?). All the calculations provided in your solution are made using $\vec{V}$.
The reason they introduced $v_1$ (speed of the block wrt wedge) is because we don't know what angle $\vec{V}$ (velocity of the block with respect to the ground) makes with the horizontal axis : we don't know the direction of $\vec{V}$. On the other hand, because the block slides tangentially on the wedge, we do know the direction of the relative velocity of the block wrt the wedge ($\vec{v_1}$ makes an angle of 45 degrees with the horizontal axis). So, we can use that to solve the problem. This has nothing to do with non-inertial frames as you suggested.
2)
$$KE_{\text{ of the block with respect to the ground}} = {1 \over 2}m |\vec{V}|^2 = {1 \over 2}m \vec{V}\cdot\vec{V} = {1 \over 2} m ({V_x}^2 + {V_y}^2) = {1 \over 2} m ((v_1 cos(45)-v)^2 + (v_1sin(45))^2)$$
Let me know in the comment if you have questions.
EDIT :
When you face questions like this, you should go back to the derivations : see where these conservation laws come from and check what the assumptions made were.
I'm going to start at the Work-Energy theorem (can be derived from Newton's second law). It states that the work done in an inertial frame by all the external physical forces on a particle of mass $m$ is equal to the change in its kinetic energy.
$$ W^{(ext)} = {1 \over 2}m (v_f^2 - v_i^2) = T_f - T_i \tag{1}$$
Let's say that there's only one force (call it $\vec{F}$) that's doing work (can be generalized to many forces). If the force is conservative, we can write it as $\vec{F}=-\nabla V$. This in turn gives,
$$W = \int_i^f \vec{F}\cdot d\vec{s} = - \int_i^f \nabla V \cdot d\vec{s}= V_i-V_f \tag{2}$$
Combining $(1)$ and $(2)$ gives,
$$ V_i + T_i = V_f + T_f $$
Now, let's consider your system. It's a non-inertial frame since the wedge is under an accelerated motion with respect to the ground (inertial) frame. But no worries, the Work-Energy theorem can be extended to non-inertial frames provided we include the work done by pseudo forces. Pseudo forces are the "correction" terms added to make Newton's law work in non-inertial frames. These forces have no physical origin.
$$\text{In a non-inertial frame : } \vec{F}_{(net,physical)} \neq m\vec{a} \Rightarrow \vec{F}_{(net,physical)} + \vec{F}_{(correction)} = m\vec{a}$$
For your block system in the wedge frame, there are three forces acting on the block [gravitational force (which is conservative), normal force (which does no work since $\vec{v_1}$ is perpendicular to the force) and pseudo force ($\vec{F}_{(correction)} = -m\vec{a}_{\text{ of the wedge with respect to the ground}}$)]. This pseudo force does work on the block however.
Therefore,
$$ W_{\text{ (by the pseudo force)}}+ mgrcos(45) = {1\over 2} m v_1^2$$
There's also a simple intuitive example to see why the law of conservation of energy wouldn't work in a non-inertial frame. Consider a train that has a box inside it (the train's surface is frictionless). When the train begins to accelerate in the ground frame and start moving, we know that the box is not going to move and simply stay at the same place since there are no forces acting on it (wrt ground frame). But, when you are in the train's frame, you observe that the box was initially at rest and then it started moving at greater and greater speed in the opposite direction. Where does its kinetic energy come from?