0
$\begingroup$

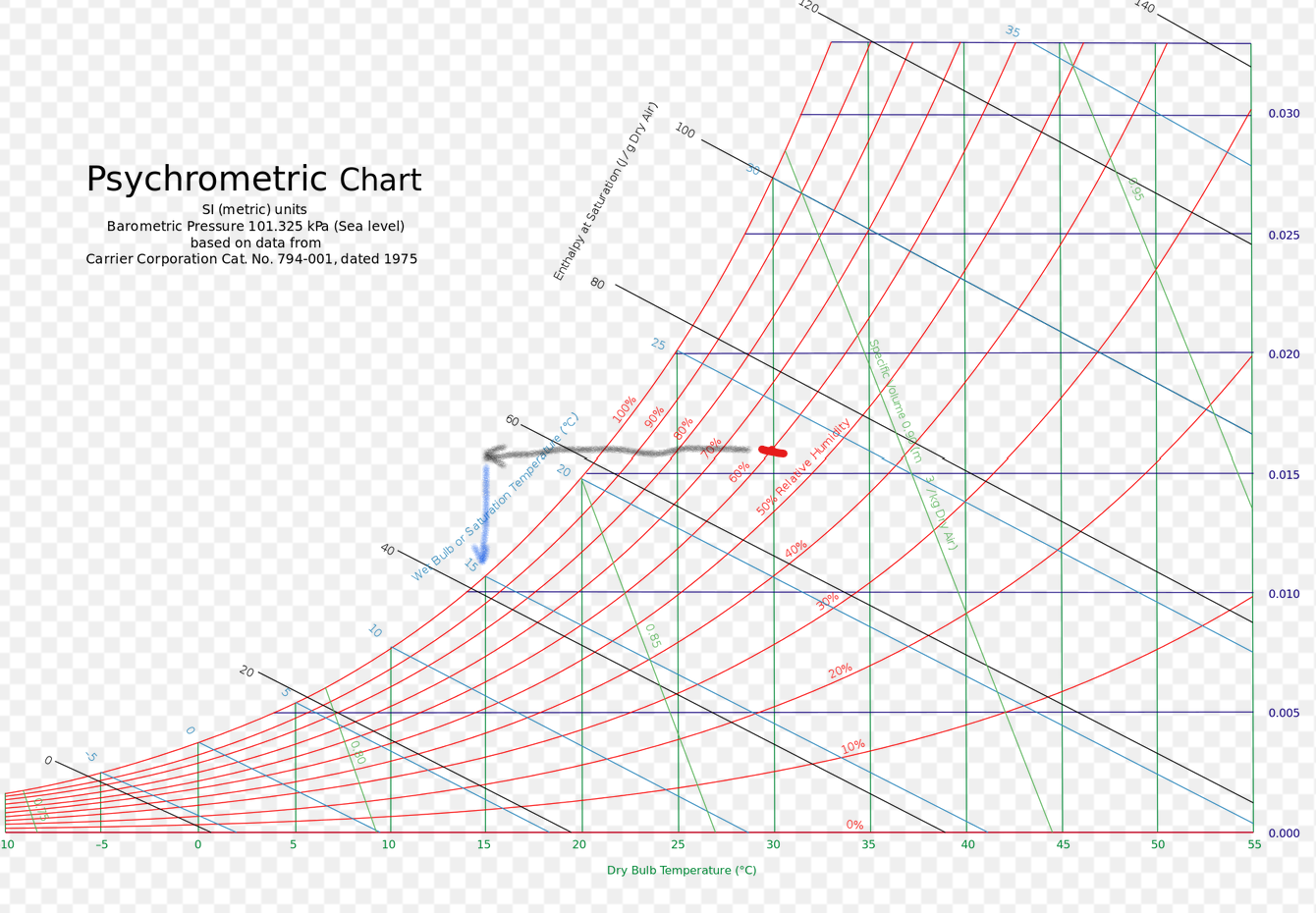
Assume that we have a box of $1\ \mathrm{m^3}$ with a constant wall temperature of $15\ \mathrm{^\circ C}$. The box was filled with air. The temperature of the air was $30\ \mathrm{^\circ C}$ and the relative humidity was $60\ \%$. After cooling the temperature of the air is equal to the wall temperature.

How can I figure out how much a change in temperature affects the relative humidity? Sadly, we don't know the amount of condensed water.

Improve this question
$\endgroup$

2 Answers 2

Reset to default
1
$\begingroup$

At 30 deg C, calculate the vapor pressure of water using the Antoine equation (https://en.wikipedia.org/wiki/Antoine_equation). Multiply this value by 0.6 to arrive at the partial pressure of water vapor at the start of cooling.

Now, use the Antoine equation to calculate the vapor pressure of water at 15 deg C. Divide this vapor pressure by the partial pressure from above. If your value is less than 1, there is no condensation, and you have the new relative humidity in the box. If the number is greater than 1, there will be condensation, and the air in the box will be at 100% humidity.

If there is condensation, use the ideal gas law to calculate the amount of water in the box at 30 deg C and the amount of water in the box at 15 deg C. The difference is the amount condensed. Note that water vapor is not truly an ideal gas, but that assumption will be reasonably close.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thank you for your answer. I have a few questions about the Antoine equation. " If your value is less than 1, there is no condensation, and you have the new relative humidity in the box. If the number is greater than 1, there will be condensation, and the air in the box will be at 100% humidity. " - is that assumption correct? $\endgroup$
    – solaryasha
    Commented Aug 30, 2019 at 12:57
  • $\begingroup$ I thought that the partial pressure of the vapor $after$ cooling should be lower than $before$ cooling $\endgroup$
    – solaryasha
    Commented Aug 30, 2019 at 13:02
  • $\begingroup$ The partial pressure is set by the initial conditions. You can calculate a partial pressure at 100% humidity from the Antoine equation at the final conditions. Both conditions allow you to calculate the number of moles present, from which you can calculate the amount condensed (if that happens). $\endgroup$
    – David White
    Commented Aug 30, 2019 at 16:04
0
$\begingroup$

This sort of questions can be answered using a psychrometric chart, such as the one below, adapted from Wikipedia. The initial conditions are marked in red (30C, 60%RH). The decrease in air temperature follows the black arrow. As you can see, this arrow brings you beyond 100%RH, therefore relative humidity will be 100%. The blue arrow denotes the amount of water that has to condensate so that humidity does not exceed 100% (units are g water per g of air).

Psychrometric chart from Wikipedia

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thank you for the information, it is really helpful! $\endgroup$
    – solaryasha
    Commented Aug 30, 2019 at 15:30

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.