Parity operator expression in relativistic quantum mechanics

Question

I was reading Schwabl's Advanced quantum mechanics. In that book it is written in the Spatial reflection part that the parity operator is $P=e^{i\phi}\gamma^0$.But after some lines it is written as $P=e^{i\phi}\gamma^0P^{(0)}$ where $P^{(0)}$ causes the transformation $\mathbf{x}=-\mathbf{x}$. But I do not get this.If I proceed with the first one I get $$\psi^\prime(x^\prime)=P\psi(x)=e^{i\phi}\gamma^0 \psi(\mathbf{x},t)=e^{i\phi}\gamma^0\psi(-\mathbf{x^\prime},t)$$ If I proceed with the second one I get $$\psi^\prime(x^\prime)=P\psi(x)=e^{i\phi}\gamma^0P^{(0)} \psi(\mathbf{x},t)=e^{i\phi}\gamma^0\psi(-\mathbf{x},t)=e^{i\phi}\gamma^0\psi(\mathbf{x^\prime},t)$$ The two statements do not seem to be equivalent. Can anyone elaborate how these two are equivalent?I am also giving the screenshot of the page.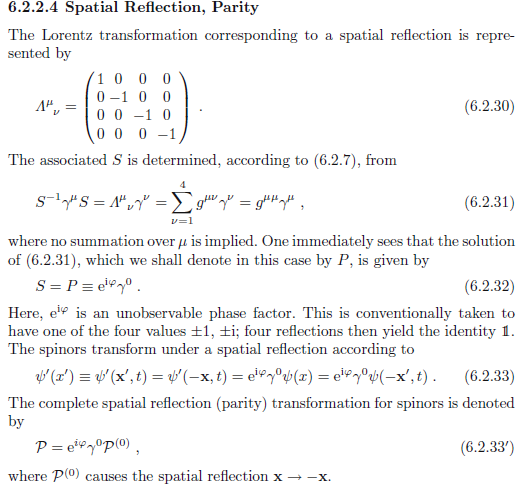

P.S. This is my first question in stackexchange. So, any suggestions regarding question is heartily welcomed.

Answer 1

$\let\g=\gamma \let\pd=\partial$

In that book it is written in the Spatial reflection part that the parity operator is $P=e^{i\phi}\,\g^0$.

This I can't believe. Please re-read carefully. I don't know that book but I'd bet he's saying that in order to correctly transform Dirac's equation then you must change sign to the $\g^i\pd_i$ part of the equation. This is rightly accomplished by $\g^0$, which anticommutes with $\g^i$. Then $P^{(0)}$ takes care of inverting coordinates in $\psi$.

Answer 2

To fully understand this, you should really read into the Poincare group, i.e. the Lorentzgroup+Translations. There you can obtain that every field transforms under Lorentz trafos like a scalar one plus an additional generator, which is caused by its spin. For spin-1/2, this additional transformation is the famous $S= e^{i w_{\mu\nu} S^{\mu \nu}}$, where w are the parameters and the generator is proportional to the commutator of the Dirac matrices. But, which is often forgotten, you also have the scalar transformation. As scalars are invariant, you would have for parity $\phi_P ( x_P) = \phi (x)$. As the psi also transforms like this, you should also end up with $\psi_P (x_P)= S \psi (x)$. I hope this helps a bit.