Recently I've begun to study magnetism and I'm familiar with the fact that all wires (however ideal they may be) will have a magnetic field around them when they have current passing through them and hence all wires have inductance.
Now let's suppose we have a very long wire carrying some steady DC current.
We want to calculate the net magnetic field around a length $x$ along the wire(just a bit of imagination).
If at a point on the wire(starting point), we add all the magnetic fields (on the imaginary disk perpendicular to the wire at that point) from radius $0$ to $R$ where $R$ is the maximum radius after which the field is too weak to be noticed. Now this field will be constant for any nearby point if the wire is sufficiently long enough. It will be $$\frac{\mu_0I}{2\pi}\int_0^R\frac1 r dr$$ Let's now find the net field for the whole length $x$. (I'm not sure how to do that)
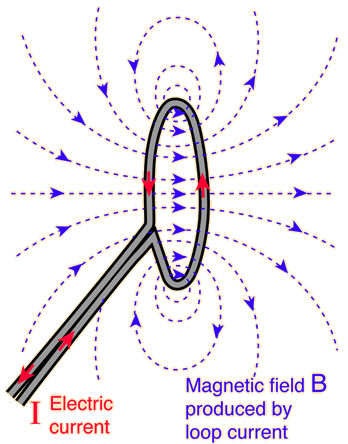
Now if we make a bit of that wire into a circle of perimeter $x$ the magnetic field around that loop will be like this.
What I want to know is whether the net magnetic field around the (previously straight) $x$ m of wire is the same as the net field around the loop of $x$ m, and similarly,
if that same $x$ m of wire is made into an inductor whether the net magnetic field will also be the same ( I mean sum of the fields both inside and outside the inductor).
Also, a certain amount of energy is used up in creating the fields so if the magnitude of fields around in the three cases are the same, the energy required to create them should also be same though energy densities will vary in the three cases.
But it is generally told that the magnetic field around a wire is negligibly small hence it doesn't take up noticeable energy to create it but how does that energy suddenly become big enough to be able to notice when the same length of wire is made into a loop and an inductor?
P.S. I might have misconceptions...Please clear them as I'll be blessed. I know a healthy bit of calculus so feel free to use it though I'm not well acquainted with vector calculus.
It will be very helpful for me to have an answer...Thanks!