If you ask around about magnetic fields, you will read seemingly-authoritative articles which say magnetism is a consequence of length contraction.
They should say that magnetic fields and magnetic forces are a unique and required element to add to electric fields and forces in order to make it relativistically invariant. Which is a slightly different thing to say.
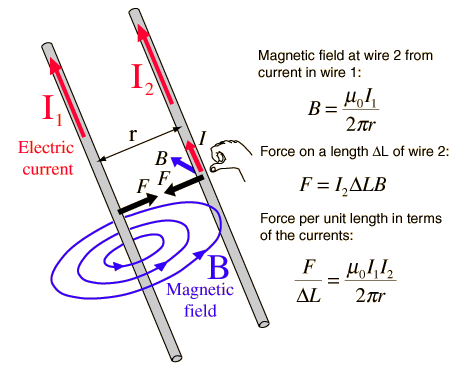
This is widely taught and is repeated in answers such as this one which talks about the magnetic force between current-carrying wires.
I think they answer cited lines up with what I said, not with what you said.
I'm sure we're all happy with this force, which you can see described in the picture from Rod Nave's hyperphysics
We aren't happy enough to not make comments and pass judgment, especially if you don't want to be wrong. So skip to the next quote if being totally right about the case of the wires and what's going on and why is not important.
Let's step back a tiny bit further and notice that Maxwell and Lorentz do not together predict that two wires feel an acceleration in any particular direction just because they have current flowing between them. And that stems from the fact that Maxwell is a Partial Differential Equation (PDE) and so is affected by boundary conditions. So namely we need first that the boundary conditions give that magnetic field you listed first. Except of course that doesn't happen either.
So let's go back to the beginning. Linearity. You can find an electric field due to some charge, current, change in charge and change in current. Jefimenko's equations are fine. As is any other solution to Maxwell's equations with just that source. Then by linearity you can add those solutions together and get a solution to Maxwell with both those sources. Then you can add any vacuum solution to Maxwell and get another solution to Maxwell. And then you could worry about meeting any boundary conditions such as zero field in a conductor, which is a boundary condition for the whole field (due to this source plus that due to that source plus the vacuum solution).
Now that means the true electromagnetic field is going to be due to all the charge, and all the current (both wires) and also from the vacuum solution. And that's what the force is going to be based on (with a possible exception of a charge feeling a force due to its own field). And the circular field you drew is just the field from just one of the wires. Not the whole field that causes forces.
So if you are going to switch frames you have to know how all the fields change, the field due to one wire, the field due to the other wire, and the vacuum solution. And the first problem is that Maxwell doesn't tell you any of them, it only tells you the sum of all three and only when you provide boundary conditions. And Lorentz isn't going to give forces until you get the whole field.
So if you want the force (per unit length) on the wire you should find the force on each part and average it together. The force on each part is just the force on each charged part. And in the frame of that part, the only electromagnetic force is the electric force. So pick a part, and pick the global inertial frame that is instantaneously comoving with the charge being analyzed right now that special relativity postulates to exist. A realistic frame could move in any direction because there is thermal motion to the parts, this would produce radiation from the finite temperature of the charged parts so would give a more complicated answer than we are aiming for. But we can easily consider the other extreme the positive charges at rest and in their frame seeing zero charge density anywhere.
Now the usual argument would be that the electric field due to zero charge density everywhere is zero. But there are vacuum solutions with electric fields, so this isn't given. Plus we are supposed to be considering the actual fields. Plus even from Jefimenko's solutions we see that electric fields can be nonzero for many reasons, but not in a static situation.
So we can choose an electric and magnetic field for each wire to be the one you drew at the beginning (zero for the electric field). And have a vacuum solution that is zero. And have a zero charge density and a nonzero current density that goes straight up and down in each wire and is zero elsewhere.
In that case the current could be realized by a negative line charge density with a motion in one direction and a positive line charge density at rest. This is the opposite extreme as including the thermal motion.
Now the positive line charge feels no force due to the zero electric field from the other wire. Or from the zero vacuum field. Now we can include the field of the wire itself or we can hope that that any force the wire parts exert on each other come in action reaction pairs and hence cancel out when we sum the forces on all the parts of the wire.
Since the third law doesn't hold for charges alone, the latter isn't obvious (or even true for the case of thermally moving charges that radiate). The third law is, in electromagnetism, a balance between the field momentum and the particle momentum. And we get our first serious complication. It is the mobile electrons that are going to feel the magnetic force, and the positive charges in the same wire moves because of a Hall voltage. So there is going to be an electric field. Which means there is both, so there is a field momentum (and to be honest even if there weren't field momentum there can be a flux of field momentum just like there can be a current density even when there is no net charge density).
One approach is to railroad everything. We could use other non electromagnetic forces to hold the wires still overall and to also make the charges that make the current flow a straight up and down. Then there is no hall voltage, no electric field and no momentum. But there is still a nonzero flux of momentum, so no obvious third law allowing you to ignore the fields one wire. But you could also consider two wires with current already flowing and then ask about how the force changes as you bring them closer while keeping the current steady.
This allows a wire to contract or expand or exert stress or tension on itself and refocuses the attention on additional forces due to the other wire. This also addresses any concerns about transient effects from turning current on or off. Leave the current on and steady, and bring the wires closer together as slowly as you want.
So we've now gotten to the point where we need to consider the forces due to the field you drew. And in the frame of the stationary charges, no force. So now let's move to the frame of the mobile charges making the current. We are still focusing on the force due to the other wire, but now length contraction changes the linear charge density of the formerly stationary line charge compared to the formerly moving line charge in the other wire. This charge imbalance can be imagined to produce an electric field in the new frame.
However this idea of breaking a field into three parts: a field due to this wire, a field due to that wire, and a vacuum field; isn't obviously unique. So just because one field seems natural in one frame doesn't mean the natural seeming field in another frame is the transformation of that first field.
Normally we could find out how fields change in different frames by appealing to the definition in terms of forces and put in test particles with different velocities in enough directions and magnitudes that we know the electric and magnetic field. But if our goal is to find out the magnetic force we can't use it to find how the electromagnetic field transforms.
This is bad bad bad. It means we have something in one frame and have zero idea what it looks like in another frame. And that's because we didn't really have a thing.
So if we want to derive a magnetostatic force on steady wires from length contraction we have to define something somewhere. And one option is to define an electrostatic force for static charge distributions.
If you do that, then we get a force from the charge density and then we can compute how hard the wire is held to keep it steady. Then use regular transformation of forces (from regular transformation of energy, momentum, and time) to find out the force in the original frame.
So we do get that electrostatic force laws plus relativity (not just length contraction, we need to transform the force back to the original frame) plus a focus on forces due to a different wire give the result we wanted. Now since it is all in statics we can't actually move the wire, we have to consider a bunch of universes with different wires at different positions. On paper we can do this. And report how the difference in forces felt depended on how far away the wires were.
If that's what we started with, and all we have, then we still haven't defined a magnetic field. So we can't even say there is a circulating magnetic field. At some point you have to define an electromagnetic field. And then it will all depend on your definitions like any theoretical construct does.
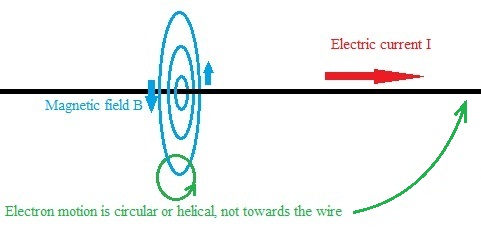
However note the concentric magnetic field lines around the wire.
The ones due just to the left wire, the thing Maxwell alone (without boundary conditions) does not predict?
We know that a charged particle such as an electron will circle around these field lines, as per this depiction courtesy of Chegg homework help
It will feel a force orthogonal to the magnetic field depicted and also orthogonal to the velocity, but the actual motion will be affected by all forces on it, not just those forces.
In addition we know that a positron will circle the magnetic field lines the other way.
Sure. In the global inertial frame that is instantaneously comoving with the electron/positron there is an electric field and if that field were the same for the electron and the positron they would feel equal and opposite forces (because of their equal and opposite charge). The total field is not the same however, since the two different charges produce different fields themselves and since the total field needs to meet the boundary conditions such as inside the conducting wires, the wires have to respond to the electron/positron thus because of the wires reaction the field from the wire will be different (think image charge due to the electron/positron). And even if the external fields were the same, the usual electromagnetic self force (so called radiation reaction) requires the self field anyway. Which is different for the two charges. Again, you can't just talk about magnetic fields or forces you have to say field due to this and force due to this, and because of the image charge problem even saying force due to the wire isn't good enough.
When you place bounties for being least wrong, the answers you get are going to be really really long to avoid being wrong.
If the electron path is a left handed helix, the positron path is a right-hand helix. Or a circle, as per these pictures of an electron beam in a uniform magnetic field.
The length-contraction explanation for two wires moving together sounds fairly plausible. However there doesn't seem to be any way that length contraction in that linear wire can result in the opposite circular motion of electrons and positrons.
They feel opposite forces because in their comoving frame they see the same external electric field yet feel opposite forces (because of their opposite charge) from the same external electric field. The fact that it is circular happens because you use a different frame at every point hence are getting different electric fields and hence different forces at every point.
You could even see this whole story from the relativistic version. Keep in mind that the Dirac matrices $\gamma^0,$ $\gamma^1,$ $\gamma^2,$ and $\gamma^3,$ can be added and scaled by real numbers so are a perfectly fine vector basis for spacetime. They also naturally anticommute and so their antisymmetric products $\gamma^0\gamma^1,$ $\gamma^0\gamma^2,$ $\gamma^0\gamma^3,$ $\gamma^2\gamma^3,$ $\gamma^3\gamma^1,$ and $\gamma^1\gamma^2$ span a six dimensional space that and naturally work for rank two antisymmetric tensors in spacetime. Then you can write the electromagnetic field as $$F=\alpha_1 \gamma^0\gamma^1+
\alpha_2 \gamma^0\gamma^2+
\alpha_3 \gamma^0\gamma^3+
\beta_1 \gamma^2\gamma^3+
\beta_2 \gamma^3\gamma^1+
\beta_3 \gamma^1\gamma^2,$$
where the three alphas are related to the electric field in a frame moving in the $\gamma^0$ spacetime direction, and the three betas are related to the magnetic field in the same frame. We can recover the electric field by looking at $\gamma^0F$ and $F\gamma^0$ and noting that only the $\alpha_1 \gamma^0\gamma^1+
\alpha_2 \gamma^0\gamma^2+
\alpha_3 \gamma^0\gamma^3$ part survives $\gamma^0\frac{1}{2}(\gamma^0F-F\gamma^0)$ and so we can start with a unified electromagnetic field and then using a vector comoving with the frame and some algebra we can get the electric field in that frame.
And now realize that any linear combination of gamma matrices is just as good a vector in spacetime. So we can choose any orthonormal set of linear combinations of the gamma matrices and it is just as fine a basis. So you can take the unit tangent $u=\nu_0\gamma^0+\nu_1\gamma^1+\nu_2\gamma^2+\nu_3\gamma^3$ and get the electric field the comving frame sees. Namely $u\frac{1}{2}(uF-Fu).$ So there is one electromagnetic field, $F$ and every frame has a a unit tangent $u$ in spacetime for particles at rest in that frame, and it works just like $\gamma^0$ and any three mutually orthogonal unit spatial directions in spacetime orthogonal to $u$ work just like $\gamma^1,$ $\gamma^2,$ and $\gamma^3.$ So there is a single object and breaking it into parts is no different than choosing an orthonormal basis.
But the force due to an external electromagnetic force when a particle is at rest is just the electric force. Each particle feels the electric force in the comoving frame where it is at rest.
So you can have your $\vec B$ in some frame, then get $F=\beta_1 \gamma^2\gamma^3+
\beta_2 \gamma^3\gamma^1+
\beta_3 \gamma^1\gamma^2$ in that frame, which is perfectly find in any frame, its the invariant electromagnetic field. Then for any particle at any moment you find the unit tangent $u$ to its worldline and then $u\frac{1}{2}(uF-Fu)$ is the electric field is sees in its frame and so is the external electromagnetic force it feels in its frame.
Basically, when you have a unified electromagnetic field and no how to get it a components in any frame then the force a particle feels from an external field is just the electric force it feels due to the electric field in its own frame ($u\frac{1}{2}(uF-Fu)$).
How can length contraction result in electron circular motion in a magnetic field, and the opposite circular motion for a positron?
Length contraction by itself doesn't give the result. You have to figure out exactly which field you are asking about (e.g. an external field, a field due to a wire, due to a current in a wire, due to charge on the wire, etcetera). Then when you find the electric field in the comoving frame of the charge you can get the force the charge feels in that frame. And then you get the force it feels in any frame. And then you'll get it pointing in the right direction and magnitude every time.
Let's see. When a particle has a worldline with tangent $\gamma^0+v_1\gamma^1+v_2\gamma^2+v_3\gamma^3$ in magnetic field $F=\beta_1 \gamma^2\gamma^3+
\beta_2 \gamma^3\gamma^1+
\beta_3 \gamma^1\gamma^2$ then it sees an electric field in its own frame of $u\frac{1}{2}(uF-Fu)$ or $$\frac{\gamma^0+ v_1\gamma^1+ v_2\gamma^2+ v_3\gamma^3}{2}\left((\gamma^0+v_1\gamma^1+v_2\gamma^2+v_3\gamma^3)(\beta_1 \gamma^2\gamma^3+
\beta_2 \gamma^3\gamma^1+
\beta_3 \gamma^1\gamma^2)-
(\beta_1 \gamma^2\gamma^3+
\beta_2 \gamma^3\gamma^1+
\beta_3 \gamma^1\gamma^2)(\gamma^0+v_1\gamma^1+v_2\gamma^2+v_3\gamma^3)\right)=$$$$\frac{\gamma^0+v_1\gamma^1+v_2\gamma^2+v_3\gamma^3}{2}2\left(v_1\gamma^1(
\beta_2 \gamma^3\gamma^1+
\beta_3 \gamma^1\gamma^2) +v_2\gamma^2(\beta_1 \gamma^2\gamma^3+
\beta_3 \gamma^1\gamma^2)+v_3\gamma^3(\beta_1 \gamma^2\gamma^3+
\beta_2 \gamma^3\gamma^1)\right)=$$$$\frac{\gamma^0+v_1\gamma^1+v_2\gamma^2+v_3\gamma^3}{2}2\left(v_1(\beta_2 \gamma^3-\beta_3\gamma^2) +v_2(\beta_3 \gamma^1-\beta_1\gamma^3)+ v_3(\beta_1 \gamma^2-
\beta_2 \gamma^1)\right)=$$$$\frac{\gamma^0+v_1\gamma^1+v_2\gamma^2+v_3\gamma^3}{2}2\left(
(v_2\beta_3 -v_3\beta_2)\gamma^1+
(v_3\beta_1-v_1\beta_3)\gamma^2+
(v_1\beta_2-v_2\beta_1)\gamma^3
\right)$$
So in the frame of the charge it sees three components of an electric field and the magnitude is proportional to the 3d cross product of $\vec v$ and $\vec B.$ And that determines the force.
The point is we don't need a separate force law since the frame of the charge only ever feels a force due to the electric field in its comoving frame. But if you then try to write that force law in a frame other than the comoving frame of the particle you get the Lorentz force law and it depends on parts of the electromagnetic field that are electric fields in a different frame.
Let's summarize.
So if there is no force on a particle at rest, then the electromagnetic field is $F=\beta_1 \gamma^2\gamma^3+\beta_2 \gamma^3\gamma^1+\beta_3 \gamma^1\gamma^2$ and then a different particle, not at rest, moving with worldline with momentary tangent $\gamma^0+v_1\gamma^1+v_2\gamma^2+v_3\gamma^3$ will see an electric field in its instantaneously comoving frame with components proportional to $\left(
(v_2\beta_3 -v_3\beta_2)\gamma^1+
(v_3\beta_1-v_1\beta_3)\gamma^2+
(v_1\beta_2-v_2\beta_1)\gamma^3
\right)$ which is related to $\vec v \times \vec B$ so the force is in some sense always the electric force in the frame of the particle.
And the fact that the two charges go in different directions is just because they have opposite charges. And the fact that the force doesn't change the speed is just because the force is orthogonal to the velocity, just like you asked for.
So the only thing left to explain is why in the first frame their was no electric field, which is straightforward if you use Jefimenko's equations and assume $\rho=0,$ $\dot\rho=0,$ and $\dot{\vec J}=\vec 0.$ Otherwise you have to open up a can of very hairy worms and deal with saying which fields come from where, which fields you are worried about computing and how to handle boundary conditions, and the fact that the wire reacts to the finite charged electron/positron and that the electron/positron can react to its own field and so on.
Where does length contraction come in? If you computed the electric field directly in the different frame then you'd need to compute the nonzero charge density $\rho$ in the wire in the comoving frame of the charge. And then you'd need to compute the rate of change of charge density $\dot\rho$ in the wire in the comoving frame of the charge. And then you'd need to compute the rate of change of current density $\dot{\vec J}$ in the wire in the comoving frame of the charge. Why? Because by Jefimenko's equation, that is how you compute the electric field due to something. And you need the original spacetime configuration of the charges and their motions to get these in any frame and length contraction is then there relating the different densities of the conduction charges and the non conduction charges in the different frames.
Another way is to just say there is an electromagnetic field and when you are trying to make the field due to each little piece of (possibly moving) charge you place a bit of electromagnetic field at each point in spacetime and then do not need to worry about which frame (of any) you might later compute the charge or the field since people should agree. So it would be like taking the Liénard–Wiechert fields for $\vec E=(\alpha_1,\alpha_2,\alpha_3)$ and for $\vec B=(\beta_1,\beta_2,\beta_3)$ and then just making $F=\alpha_1 \gamma^0\gamma^1+
\alpha_2 \gamma^0\gamma^2+
\alpha_3 \gamma^0\gamma^3+
\beta_1 \gamma^2\gamma^3+
\beta_2 \gamma^3\gamma^1+
\beta_3 \gamma^1\gamma^2,$ and letting anyone break it into parts however they like.
And since you might read this the entirely wrong way. I will say the following. The magnetic field in a frame exerts forces on moving charges in that frame. Take the instantaneously comoving frame of the charge. In that new frame compute the Liénard–Wiechert fields for $\vec E=(\alpha_1,\alpha_2,\alpha_3)$ alone (no magnetic fields) and you'll need relativity such as length contractions to compute the electric field in the new frame using the data in the original frame. And that's all people were ever trying to say. Just use the electric field from the Liénard–Wiechert fields in the comoving frame and you get the actual physical force felt in that frame so can vet it in any other frame. Electric fields and forces in all frames gives you everything. For people that love covariant methods instead of invariant ways, the electromagnetic field is just a way to easily generate an electric field for an arbitrary frame.
The rest is me just trying to not let anything wrong slip by. This doesn't address self forces. It isn't about vacuum solutions to Maxwell. And the whole issue of boundary conditions and reactions is a serious issue.
You could use equations like $u(uF-Fu)/2$ that never require you to pick some direction in space and say you have a frame. They can do it by just computing the spacetime rotation of the worldline's tangent continuously parameterized by proper time in a frame invariant (not just covariant) way, and then there is just a frame invariant $F.$ Or else, you could do covariant physics where you write your equations in an arbitrary frame and then we find that F is just a way to have enough information to get the $\vec E$ in every frame.
It's not like we can say covariant is wrong and invariant is right since they make the exact same predictions.