From the constraint $v=a\dot{\phi}$ of a rolling disk over a plane, where $a$ is the radius of the disk we can derive these two equations:
we have two differential equations of constraint:
$dx=asin\theta d\phi$
$dy=-acos\theta d\phi$
Can you rigorously explain to me why can't I integrate these functions? Is it their non-integrability that makes these constraints non-holonomic?
HINT: Rewrite the first constraint as $$ f \left[ dx +(- a \sin \theta) d\phi + (0) d\theta \right] = 0. $$ where $f(x, \theta, \phi)$ is some unknown integrating function. We want to know whether this can be written as $$ dg = \frac{\partial g}{\partial x} dx + \frac{\partial g}{\partial \phi} d\phi+ \frac{\partial g}{\partial \theta} d\theta $$ Assuming that $g$ is a "nicely-behaved" function of the coordinates, its mixed partial derivatives are independent of the order of differentiation. Using this fact, can you show that this implies $f = 0$?
To answer your second question: there are different definitions of "non-holonomic" constraints used by different authors. If you're following Goldstein, any constraint that cannot be written in the form $f(x_i) = 0$ is non-holonomic; but there are also non-holonomic constraints that can only be written as equalities among the higher derivatives, or as inequalities.
In classical mechanics, holonomic constraints are relations between the position variables (and possibly time) that can be expressed in the following form: $g(y,t) = 0$
Nonholonomic constraints is when we can't express it in the form given above, and have to express it as $g(y,y',t) = 0$, where y' must be a variable to the constraint.
But, be careful, even though some constraints giving in the form $g(y,y',t) = 0$, it does not mean it can't be converted to a holonomic constraints of form $g(y,t)-$constant$ = 0$. These constraints that can be converted are actually holonomic constraints.
Thus the constraints that cannot be reduced to holonomic constraints by integration are nonholonomic constraint.
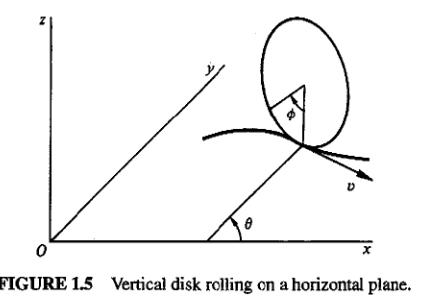
Now let $g(x,\theta,\phi,t) = dx-a\sin\theta d\phi = 0$ be our constraint.
If the differential constraint was exact the it should be in the form of
\begin{equation}\frac{dg}{dt} = \frac{\partial g}{\partial x}\frac{dx}{dt} + \frac{\partial g}{\partial \theta}\frac{d\theta}{dt} + \frac{\partial g}{\partial \phi}\frac{d\phi}{dt} +\frac{\partial g}{\partial t} =0\end{equation}
which is just
\begin{equation}\frac{dg}{dt} =0\end{equation}
which can be integrated into
\begin{equation}g(y,t)-constant = 0\end{equation}
which is a holonomic constraint
Showing that no integral exists
By dividing $g(x,\theta,\phi,t)$ by $dt$ and add $0\frac{d\theta}{dt}$ thus we get:
\begin{equation}\frac{dx}{dt}-a\sin\theta\frac{d\phi}{dt}+ 0\frac{d\theta}{dt} = 0\end{equation}
I order for it to be exact, we must be able to get
\begin{equation}\frac{\partial g}{\partial x} = 1,\frac{\partial g}{\partial \theta} = 0,\frac{\partial g}{\partial \phi} = -a\sin\theta\,\frac{\partial g}{\partial t} = 0\end{equation}
Suppose we want to try integrate to get g that matches the above partial derivatives
Method 1
\begin{equation}\frac{\partial g}{\partial x} = 1\end{equation} integrate with respect to $x$, \begin{equation}g = x + k_1(\theta,\phi,t)\end{equation} differentiate with respect to $\theta$, \begin{equation}\frac{\partial g}{\partial \theta} = k'_1(\theta,\phi,t) = 0 \end{equation} integrate with respect to $\theta$, then sub in for $k_1$, \begin{equation}g = x + \theta + k_2(\phi)\end{equation} differentiate with respect to $\phi$, \begin{equation}\frac{\partial g}{\partial \phi} = k'_2(\phi,t) = -a\sin\theta\end{equation} integrate with respect to $\phi$, then sub in for $k_2$, \begin{equation}g = x + \theta + a\cos\theta + k\end{equation}
But if we take $\frac{\partial g}{\partial \theta} = -a\sin\theta + 1\neq 0$ thus NOT integrable.
Method 2
Or we could simply do the exact check \begin{equation} \left(\frac{\partial g}{\partial y_i}\right)_{y_j} = \left( \frac{\partial g}{\partial y_j} \right)_{y_i}\end{equation} and immediately we can see that \begin{equation} \left(\frac{\partial g}{\partial \phi}\right)_{\theta} = -a\cos\theta \neq 0 =\left( \frac{\partial g}{\partial \theta} \right)_\phi\end{equation}
which fails the exact check and thus NOT integrable.
