0
$\begingroup$

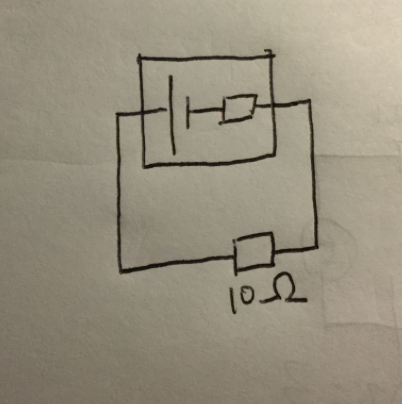
Say we had a circuit with a cell (with internal resistance)and an external resistor of $10 \Omega$ as shown:

enter image description here

The $10\Omega$ resistor then increases in temperature, leading to an increase in resistance in this resistor. My thinking was that since $10 \Omega$ resistor now has a value higher than $10\Omega$, more voltage drops across this resistor. Since your emf is constant, less voltage must drop across the internal resistor. Less voltage drop means less resistance. Where is the flaw in my logic?

Improve this question
$\endgroup$
2
  • $\begingroup$ Welcome to SE.Physics! Since you're asking about why your calculation for voltage changes, how are you calculating it? What information/measurements do you have, and what equations are you using? $\endgroup$
    – Nat
    Commented May 18, 2019 at 6:28
  • $\begingroup$ Ignore the small resister initially. In this case, the voltage drop across the $10\ \Omega$ resistor is the same as the $V$ supplied by the battery. Since $V=IR$, this implies $I=V/R$ where $R=10\ \Omega$ and $V$ is constant. If $R$ increases because of higher temperature, then $I$ decreases since $V$ is constant. Adding the small resistor in series with $R$ increases the total resistance which decrease the current further. $\endgroup$
    – Cinaed Simson
    Commented May 18, 2019 at 7:21

4 Answers 4

Reset to default
1
$\begingroup$

The flaw is in the statement 'Less voltage drop means less resistance.' A reduction in potential difference between two points can be caused either by a reduction in resistance or by a reduction in current, depending upon the circumstances.

The overall voltage drop across the internal and external resistance is shared across the two elements in proportion to the resistance they offer. If the external resistance increases, it will take a larger share of the overall voltage drop. In the extreme cases:

-where the external resistance is infinite, there will be no voltage drop across the internal resistance;

-where the external resistance tends to zero, all the voltage drop is across the internal resistance.

Improve this answer
$\endgroup$
0
$\begingroup$

Your logic is not flawed ( assuming the internal resistance is constant). However, if the internal resistance is much smaller than the load resistance, the increase in voltage across the load resistance may be difficult to measure. This is because a small change in the load resistance will have little effect on the cell’s terminal voltage. In other words the battery will approach being a constant (ideal) voltage source (terminal voltage = emf).

Hope this helps.

Improve this answer
$\endgroup$
0
$\begingroup$

Since your emf is constant, less voltage must drop across the internal resistor

By constant emf, I understand that a voltimeter reads the same value between the poles of the cell, no matter if the external resistance is $10 \Omega$ or more. So, by definition the voltage drop doesn't change, but the current decreases from its initial value when the temperature of the resistor increases.

Improve this answer
$\endgroup$
1
  • $\begingroup$ This is not what constant EMF means. For a battery with constant EMF the voltage across the poles depends on the resistance of the external load. EMF is not the same as the voltage across the battery's poles unless you have an ideal battery. Which is not the case here. $\endgroup$
    – nasu
    Commented Mar 15, 2022 at 4:36
0
$\begingroup$

Ohm's law states that if the external resistance goes up, the current goes down. The voltage is constant if you ignore the voltage source's internal resistance. If you account for the small internal resistance the voltage of the source goes a little up.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.