What causes the back of a bike to lift when the front brake is applied? (Like in an endo.)
Also, if I were to replicate this effect with a wood block with wheels that crashes against a wall (only the wheels touch the wall) would it work?
$\begingroup$
$\endgroup$
2
-
6$\begingroup$ inertia! my friend, inertia... $\endgroup$– Vineet MenonCommented Dec 13, 2012 at 16:36
-
$\begingroup$ Note that the usual case of an endo (as opposed to a 'stoppie'), the back of the bike doesn't lift directly: the friction of the rider to the seat is lower than the friction of the wheel to the road, the bike stops, the rider keeps moving forward (inertia), and the rider's body (legs) hits the handlebars pulling the bike over. $\endgroup$– freiheitCommented Jan 16, 2013 at 18:01
Add a comment
|
5 Answers
$\begingroup$
$\endgroup$
7
Applying the brakes makes the wheel stop turning in relation to the bicycle's frame but not in relation to the road. The bike's center of mass (especially with a rider pressing against the handle bars) is higher than the hub of the front wheel.
When the brakes are applied that mass has momentum toward the front of the bike that exerts a force on the front wheel to turn toward the front of the bike. Since the wheel can still turn on the road, it does, but since the wheel and bike are locked together, the bike rotates with the front wheel.
-
-
$\begingroup$ The front wheel does not need to stop. It only needs to decelerate "quickly enough". That means decelerate the front wheel quicker than the center of mass can be decelerated by a force applied to the contact point between front wheel and road. $\endgroup$– ndimCommented Dec 13, 2012 at 22:16
-
1$\begingroup$ The height of the hub relative to the center of gravity has nothing to do with it. Even if the center of gravity were lower than the hub, the object could tilt forward with enough acceleration. All that has to happen is that the force of acceleration plus gravity acts on the center of mass through a line of action that falls outside the object's base of support. $\endgroup$– KazCommented Dec 14, 2012 at 1:15
-
$\begingroup$ @Kaz, do you mean deceleration or are you assuming the bike is headed down a steep hill? $\endgroup$ Commented Dec 14, 2012 at 14:52
-
$\begingroup$ @Kaz, If the center of mass were in line with the hub of the wheel, then would the friction between the road and the tire be high enough to keep the tire from just skidding forward without the backup rising up? $\endgroup$ Commented Dec 14, 2012 at 18:20
$\begingroup$
$\endgroup$
3
There is simplified "cheat" method for thinking about this.
The hard way is to draw a free-body diagram of the bicycle, in which all the forces acting on it are made plain: the force of gravity acting through its center of mass, the force of friction from braking, and so on.
The cheat method is to visualize the bicycle in an accelerating frame of reference, and then to pretend that the acceleration is just a form of gravity. Under Newtonian physics, acceleration is not distinguishable from gravity.
For instance if you are standing on a train which accelerates, then you simply tilt forward, as if you were standing on a slope. It feels exactly as if gravity has increased and slanted.
So the reason the rear wheel lifts is because, since you are decelerating, it is as if you are cycling down a steep slope (on a planet where gravity is a little big greater than that of Earth, but that is irrelevant). If you're on a slope facing downhill, your bike is tilted forward, so that its center of gravity is shifted so that it is more above the front wheel, rather than the rear.
If the tilt is sufficiently pronounced, your center of gravity will move ahead of the front wheel, and at that point, the rear wheel will lift.
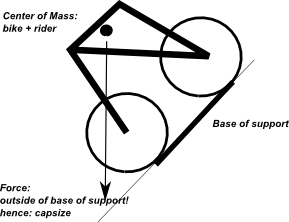
While that force still acts through the base of support, the rear wheel will not lift. However, there is also the question of how much support comes from the rear versus the front wheel. Even before the rear wheel lifts, "weight transfer" takes place to the front wheel. The bike and rider are supported more by the front than the rear wheel, because the imaginary force acting through the center of mass intersects the imaginary support base closer to the front wheel than to the rear wheel. This weight distribution toward the front limits the effectiveness of brakes on the rear wheel, since brakes can only work to the extent that the wheel can develop friction against the road. Also, it means that you can never brake so hard using the rear brake alone that the bike will tilt. As soon as it is close to the verge of tilting, the braking power is lost: an example of stabilizing negative feedback.
-
$\begingroup$ That's an awesome answer, and it makes so much sense. $\endgroup$– DLACommented Dec 15, 2012 at 1:05
-
$\begingroup$ I figure I'll have to make my model have a slope when it collides for it to work properly then. $\endgroup$– DLACommented Dec 15, 2012 at 1:06
-
$\begingroup$ Note that there are some limitations to this slope analogy. As you approach 90 degree slope, the component of the gravitational pull which is in the direction perpendicular to the sloped ground approaches zero. But under deceleration on level ground, the gravitational component never approaches zero! The force is always equal to the weight of the bike and rider. $\endgroup$– KazCommented Jan 10, 2013 at 23:01
$\begingroup$
$\endgroup$
9
If we disregard wind resistance, there are three points where external forces apply to the combined bike/rider body: The front and rear wheel contact points with the road, and the combined center of mass. If these forces generate a torque that is not zero, the rear wheel will lift off while braking (or the front wheel lift off during hard acceleration).
If you want to replicate the system with a block of wood with wheels bumping into a wall, use very small wheels. If the front wheel suddenly stops due to braking on a bike, the bike will rotate around the contact point of the front wheel. On the other side, if you run the front wheel into a wall, the front wheel will stop and the center of the rotation will be the front wheel hub. (Assuming sufficiently stiff wheels.)
Some time ago, I have hacked together an interactive web page showing the forces to a braking/accelerating trike/bike on different slopes. Playing with that page might help you understand (requires SVG+CSS+Javascript).
I have originally built the page for a tadpole tricycle. For looking at a standard bicycle, select "upright bicycle" on the page and then play a little with the + and - buttons for the acceleration/deceleration. If you have understood what is going on, try the + and - buttons for the slope as well.
-
$\begingroup$ That's a great simulation. However, I'm having some trouble understanding what causes it to flip at -0.55g and not at -0.54g. What is the difference between these two points? You also mention that the anglar momentum has to have a non-zero value. How can I calculate this angular momentum? I'm sorry for asking, I just feel I'm about to understand all of this! :) $\endgroup$– DLACommented Dec 15, 2012 at 11:52
-
$\begingroup$ At -0.54g, the vector sum of the forces on the front wheel contact point points towards the front of the center of mass. This results in clockwise torque, pushing down the rear wheel. At -0.55g, said vector sum points towards the rear of the center of mass, so the resulting torque is lifting the rear wheel. $\endgroup$– ndimCommented Dec 15, 2012 at 16:47
-
$\begingroup$ Ah, yes! That makes a lot of sense! But hang on, how can you know what these values are? Is it hypothetical, or is there a way to calculate the reaction force of the ground on the two wheels? $\endgroup$– DLACommented Dec 15, 2012 at 18:33
-
$\begingroup$ Also, I'm planning to conduct an experiment to illustrate how varying center of mass affects the extent that the wheel lifts. Do you think it would work to have a scale model of a bike (same ratio of dimensions, and same location of center of mass) on four wheels have its front wheels strike a barrier? I conducted a proof of concept with a toy car with a pencil in front, while in the real thing it would have wheels sticking out that would stop as they hit the barrier: cl.ly/Ldpg Will this yield similar results to if it was conducted with locking front wheels? $\endgroup$– DLACommented Dec 15, 2012 at 18:42
-
$\begingroup$ Thanks for your patience. Just wanted to add that the independent variable will be the position of the center of mass (towards front or back) and the dependent variable will be the angle it lifts to. Do you have any comments or criticisms? I'd prefer to deal with them sooner rather than later! Thanks again! $\endgroup$– DLACommented Dec 15, 2012 at 18:46
$\begingroup$
$\endgroup$
5
The center of mass of the bike+rider wants to keep moving forward (Newton's first law)
Since the center of mass is higher on the bike - when it goes forward the bike pivots around the point where the front wheel sticks to the road.
Imagine attaching a string to the middle of the wooden block and pulling it forward, while having the front wheel stuck to the ground.
answered Dec 13, 2012 at 16:22
-
$\begingroup$ Excellent answer. Short, correct, easy to understand. $\endgroup$– BeskaCommented Dec 13, 2012 at 18:48
-
$\begingroup$ The center of mass could be lower than the front axle and the bike would still pivot around the contact point between front wheel and road. $\endgroup$– ndimCommented Dec 13, 2012 at 22:16
-
$\begingroup$ @ndim - yes, I had originally talked about brakes and the axle as the pivot then changed it. It's now a bit wrong - will fix. $\endgroup$ Commented Dec 13, 2012 at 22:49
-
$\begingroup$ Of course, the front wheel friction needs to be large enough to actually deliver the required horizontal decelerating forces. $\endgroup$– ndimCommented Dec 13, 2012 at 22:56
-
$\begingroup$ @ndmi - or you need to hit a rock! $\endgroup$ Commented Dec 13, 2012 at 23:06
$\begingroup$
$\endgroup$
3
My naive argument would be based on energy conservation. I won't write any equations. This is for you to do.
The bike + rider has both rotational and translational kinetic energy in addition to the potential energy of the system. When you apply the brakes, the translational KE is converted to both rotational KE and PE which manifests as work done against gravity. You lift up, pivoting about a point, which accounts for a change in rotational motion due to an external torque (friction).
A very interesting real world experiment which I do every day (I bike to work) is to stand up if I wish to brake efficiently (in my case it is only marginally efficient, but I do feel the difference). The translational KE is converted to PE and hence the brakes don't have to work as hard to stop my motion. The heavier the person, the more effective the technique.
So, if you are a fat bastard, this may be a useful way to stop quickly.
answered Dec 13, 2012 at 17:32
-
$\begingroup$ I must be misunderstanding you, because the way I see it, the PE when you stand up is not coming from the bike's KE, but from work done by the ground reaction from you standing up, so the only reason that may make you stop quicker is that, while you are in the process of standing up, ground reaction is increased, and so you can have a higher friction force. But this has very little to do with KE being converted to PE. Could you elaborate on your standing by braking theory? $\endgroup$– JaimeCommented Dec 13, 2012 at 18:17
-
$\begingroup$ Suppose you stand up adiabatically, where does the PE come from? Granted that one cannot really do this adiabatically as friction with the road etc will slow you down. $\endgroup$ Commented Dec 13, 2012 at 22:10
-
1$\begingroup$ The PE comes from the work done by your legs in pushing you up, in the same way you can get up from a squat without affecting your translational energy. $\endgroup$– JaimeCommented Dec 13, 2012 at 22:58