Mass-Spring Damper system - moving surface

Question

I need help with a physics problem, I don't know much about dampers, how can this be solved?

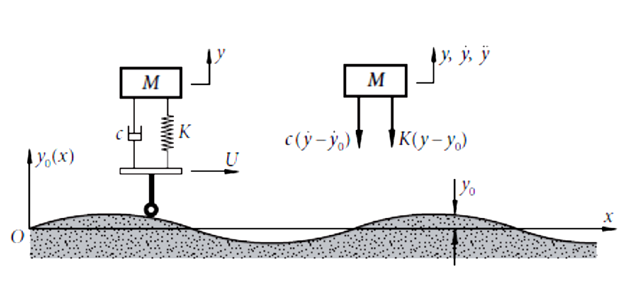
we have $y_0(x)=\mu\sin(\Omega x)$

We arrive at this equation for motion (where we define $b$ and $w_0$ ourselves, and $z=y(t)-y_0(t)$)

$$\displaystyle\frac{d^2z}{dt^2} + b\displaystyle\frac{dz}{dt} +w_0^2z = \mu\Omega^2U^2\sin(\Omega Ut)$$

Can someone show me the steps in between for determining this equation of motion

EDIT: What I have is (with $y(ii)$ meaning second derivative of $y$, and $y(i)$ first):

$$m(y(ii)-y_0(ii))=-K(y-y_0)-C(y(i)-y_0(i))$$

which simplifies as

$$(y(ii)-y0(ii))+\frac{c}{m}(y(i)-y_0(i))+\frac{k}{m}(y-y_0)=0$$

which $\implies$ $z(ii)+bz(i)+w_0^2z=0$ (which is wrong)

Maybe I could be shown a proper free body diagram by someone if my idea of the forces are wrong?

Answer 1

What you have worked out so far is the left hand side of the answer. You have written that the sum of the accelerations is zero, but that is not correct because the system is being driven by the roller moving across the sinusoidal surface. Parameterize in terms of time by writing $x=Ut$, then rewrite your $y_0(x)$ equation as $y_0(t)$. Now that you have the vertical position of the roller as a function of time, you can find its acceleration by taking the derivative twice with respect to time. The result should look comforting. This new acceleration term goes with the force that drives the system.