Dot convention inductors in series: what is going on

Question

So I'm really confused with mutual inductors and dot convention. If your answer is going to be a link to any website I can assure I read them all and that only left me more confused. So here are my questions:

-> Should we write the absolute values of the mutual inductance? If so, why? Here is the example provided by my book:

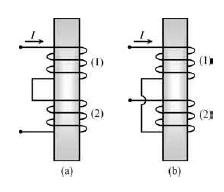
They say that for the left side we have concordant coupling (mutual inductance positive) and therefore the series inductance is going to be

$L = L_{11} + L_{22} + 2 |L_M|$

Why using the absolute value though? Isn't the coupling concordant and mutual inductance positive)? What if it was negative, do you still have concordant coupling? I can see that if we apply the right-hand screw rule the fluxes have the same direction.

And for the right side discordant coupling (mutual inductance negative) and therefore the series inductance is going to be

$L = L_{11} + L_{22} - 2 |L_M|$

Again: Why using the absolute value though? Isn't the coupling discordant (mutual inductance negative)? What if it was positive, do you still have discordant coupling? I can see that if we apply the right-hand screw rule the fluxes have opposite directions.

Next example my book provides is this one

By applying the same thinking pattern of the previous one we have discordant coupling (mutual inductance negative) and therefore the series inductance is going to be

$L = L_{11} + L_{22} - 2 |L_M|$

HOWEVER, my book now provides this

$L = L_{11} + L_{22} + 2 L_M$

I know that because $L_M$ is indeed negative this will lead us to the same result however why removing the absolute value now? Or are they assuming concordant coupling even though the mutual inductance is negative? Or is there a step I'm missing? This one didn't bothered me so much because I ended up with the same answer.

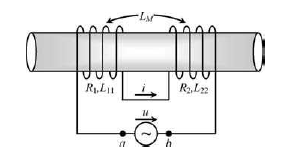
However, the final case is where I drop the ball:

So here I had previously calculated the mutual inductance and found out it was indeed negative. The series connection also suggests to me that we have we have discordant coupling (by dot convention) and therefore the series inductance is going to be

$L = L_{11} + L_{22} - 2 |L_M|$

But now my book gives the answer

$L = L_{11} + L_{22} - 2 L_M$

And since $L_M$ is negative this will originate different values.

I feel so confused right now, I have no idea how am I supposed to solve problems right this because I keep getting different answers. Can someone help me organize my line of thought. I think I understand concept of mutual inductance. What I don't understand is this thing with absolute values and series connection and I don't really know what to do.

Answer 1

I would just follow these simple rules:

1. Always consider mutual inductance, $M$, positive, in which case, $2|M|=2M$.
2. Add $2M$ (or $2|M|$) to the total series inductance, if the fluxes in the two inductors have the same direction, i.e., amplify each other, and subtract $2M$ otherwise.