How to derive the moment of inertia of a thin hoop about its central diameter?

Question

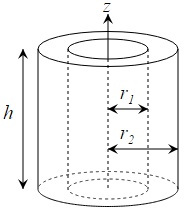
For lack of a better image, I am searching for the moment of inertia of this

where$\ r_1 = r_2$ (negligible thickness), and where the object would be rotating around its central diameter, which is perpendicular to the z-axis. Basically, either$\ I_x$ or$\ I_y$.

I could easily use the perpendicular axis theorem to find that$\ I_z = I_x + I_y = 2I_x = 2I_y$ and solve for my desired moment (x- or y-axis), which would give me $\frac{1}{2}MR^2$. Easy.

However, I have perused multiple sources and even attempted on my own to arrive at such an expression, and all of them (including mine) have included a second term including the width of the hoop, or in the case of my illustration, the height of the cylinder:$$I_x=I_y=\frac{1}{2}MR^2 + \frac{1}{12}MW^2$$

To make matters more confusing, my attempts at deriving left me with an identical expression to the one above, except$\ W$ is to the third power. I tried splitting the hoop into many rings and using the parallel axis theorem to account for the fact that every ring except the central one is a distance$\ z$ from the axis of rotation. It's likely that my issues are due to either poor math or a misconception of how I should even set this up based on$\ I=\int r^2dm$, but I would like to hear how others would go about solving this.

Answer 1

The inertia $I$ is actually a tensor whose components are

$$ I_{ij} = \int{\rm d}^3{\bf x}~\rho({\bf x}) [{\bf x}\cdot{\bf x}\delta_{ij} - x_ix_j] \tag{1} $$

So, for example the component $I_{11}$ can be calculated as

$$ I_{11} = \int{\rm d}^3{\bf x}~\rho({\bf x}) [x^2 + y^2 + z^2 -x^2 ] = \int{\rm d}^3{\bf x}~\rho({\bf x}) [y^2 + z^2] \tag{2} $$

To calculate this we need the density, which for this problem is just

$$ \rho({\bf x}) = \rho(r,\phi,z) = \frac{M}{2\pi R h}\delta(r-R) \tag{3} $$

Replacing (3) in (2) you get

\begin{eqnarray} I_x &\stackrel{\rm def.}{=}& I_{11} = \int {\rm d}r {\rm d}\phi {\rm d}z ~r \left[ \frac{M}{2\pi R h}\delta(r-R) \right] (y^2 + z^2), ~~y=r\sin\phi \\ &=& \frac{M}{2\pi R h}\left\{ \int {\rm d}r {\rm d}\phi {\rm d}z ~r \delta(r-R)(r^2\sin^2\phi) + \int {\rm d}r {\rm d}\phi {\rm d}z ~r \delta(r-R)(z^2) \right\} \\ &=& \frac{M}{2\pi R h}\left\{ R^3h \int_0^{2\pi}{\rm d}\phi~\sin^2\phi + 2\pi R \int_{-h/2}^{h/2}{\rm d}z~z^2 \right\} \\ &=&\frac{M}{2\pi R h}\left\{ \pi R^3h + 2\pi R \frac{h^3}{12}\right\} \\ &=& \frac{M}{2}\left(R^2 + \frac{h^2}{6}\right) \tag{4} \end{eqnarray}

with a similar expression for $I_y$

Answer 2

You cannot use perpendicular axis theorem b/c the hoop height along z axis is not zero (h instead). When h is zero, it can be applied. And the derived result is reduced to MR^2/2. The derivation is elegant with Dirac delta function for "thin" hoop or no thickness.

To explain it better in intuition, choosing the Cartesian coordinates as in the diagram where x,y coordinates go thru the center of the cylinder, I is dragonized and can be realized in this format (as 3x3 matrix) with z axis in the diagram.

diag(Ix,Iy,Iz)=Integral( diag(y^2+z^2, x^2+z^2, R^2=x^2+y^2) rho(x,y,z) dv )

since Iz=MR^2, and X,Y axis are symmetric, therefore each of x^2, y^2 integration contributes to MR^2/2. One also realize that z^2 integration part is the uniform bar, which is well known as Mh^2/12. Therefore the result of I tensor is:

diag( MR^2/2+Mh^2/12, MR^2/2+Mh^2/12, MR^2)