As jobe pointed out, there is a thorough treatment of your question here. You should read it. However, I think I can supplement with a more qualitative discussion and an example.
Amendment: This could use some community critiquing, so I wouldn't take it as perfectly presented just yet.
Answer:
Allow you to give my answers first, then the explanation:
- You can apply Faraday's law in it's differential form to a fixed point in space even for time-dependent boundaries. It is always valid for a fixed point in space.
- If you really want to apply the differential form to a moving point, the easiest way is to transform the E and B-fields to a moving frame.
Explanation:
The question was how to properly treat the general case of Faraday's Law:
$$\begin{align}
\oint_C\vec E \cdot d\vec l & = - {d \over d t} \iint_S \vec B \cdot d \vec A \\
\iint_S (\vec \nabla \times \vec E)\cdot d\vec A & = - {d \over d t} \iint_S \vec B \cdot d \vec A \\
\end{align}$$
Where $S$ is a smooth surface with one boundary curve $C(t)$ that may have explicit time dependence. Only when $C$ does not depend on $t$ can you bring the total derivative inside the double integral, downgrade it to a partial derivative, and then peel away the integral:
$$ \begin{align}
\iint_S (\vec \nabla \times \vec E)\cdot d\vec A & = - \iint_S {\partial \vec B \over \partial t} \cdot d \vec A \\
\vec \nabla \times \vec E &= - {\partial \vec B \over \partial t } \\
\end{align} $$
How do we know it is safe to peel off the integral? That is equivalent to declaring $\vec \nabla \times \vec E$ must equal $- {\partial \vec B \over \partial t}$ at every point $P$ on $S$. To see why this must be true imagine shrinking the surface $S$ down around the point $P$. At some point the smoothness of $\vec E$ and $\partial \vec B/\partial t$ must kick in and make these constant over the integral, thereby removing its need:
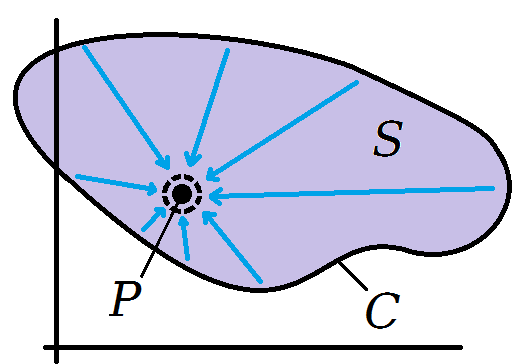
And this brings me to my first point I would like to make:
$\oint_C\vec E \cdot d\vec l = - {\partial \over \partial t} \iint_S \vec B \cdot d \vec A$ is over a surface, while $\vec \nabla \times \vec E = - {\partial \vec B \over \partial t }$ is at a point.
Allow me to now show how Faraday's law in differential form applies to loops of changing area with the classic sliding bar example:
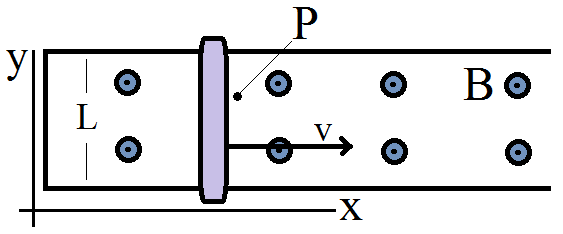
The answer is of course that voltage $\mathcal{E} = BLv$ generates clockwise current around the loop, an answer readily acquired from the integral form of Faraday's law. But what does the differential form tell us? Consider a fixed point $P$ in space about to be overtaken by the sliding bar:

Since the bar is a (perfect) moving conductor, it (perfectly) suppresses the magnetic field in its interior. This is how $P$ witnesses a changing magnetic field, even when $P$ is stationary. Let's write out the differential form with $\vec B = +B \hat z$:
$$\begin{align}
(\vec \nabla \times \vec E)_z &= -{\partial B_z \over \partial t}\\
{dE_y \over dx} - {dE_x \over dy} &= -{\partial B \over \partial t} \\
{\Delta E_y \over \Delta x} &\approx -{ \Delta B \over \Delta t} \\
E_y &\approx {\Delta x \over \Delta t} B \\
E_y &\approx v B \\
\mathcal{E} &\approx v B L \\
\end{align}$$
This is the same result as found with Faraday's law in integral form. The approximation can be made exact with a bit more rigor, but it gets the point across of how having a stationary point $P$ is no limitation in principle.
What if you really want $P$ to move:
Sure, $P$ can move. One method is to apply the Leibniz integral rule for two dimensions, which guides you through differentiation of integral limits:
$${d \over dt} \iint_{S(t)} \vec F(\vec r, t) \cdot d\vec A = \iint_{S(t)} ({\partial \vec F(\vec r,t) \over \partial t} + [\vec \nabla \cdot \vec F(\vec r,t)\vec v ])\cdot d\vec A - \oint_{C(t)} [\vec v \times \vec F(\vec r,t)] \cdot d \vec l$$
Where $\vec v$ is the velocity at each point on $C(t)$ and $\vec F$ is your vector field. I am going to quote the result of applying this to Faraday's law, since that was done expertly in the previously linked answer:
$$\vec \nabla \times (\vec E + \vec v \times \vec B) = -{\partial \vec B \over \partial t}$$
This is correct, but I find it misleading. The second method I would like to show is much simpler: convert $\vec E$ and $\vec B$ to the moving frame:
$$\begin{matrix}
\text{E and B in a moving inertial frame} \\
\begin{align}
\vec E' &= \vec E + \vec v \times \vec B \\
\vec B' &= \vec B - {1 \over c^2} \vec v \times \vec E \\
\end{align}
\end{matrix}$$
These are the (non-relativistic) conversions of electric and magnetic fields to those in an inertial frame moving at velocity $\vec v$. They make lots of quasistatic problems trivial, and Faraday's law is no different. Applying it to the sliding bar example from above:
$$\begin{array}{c|c}
\text{The rest frame} & \text{The moving frame}\\
\hline
\vec E = 0 & \vec E' = \vec v \times \vec B \\
\vec B = +B\hat z & \vec B' = \vec B \\
\end{array}$$
$\vec E = 0$ in the rest frame because there is no charge density $\rho$ in this problem and we require $\vec E' = 0$ when $\vec v = 0$. Thus from the moving bar's perspective, it sees a constant $\vec B$ field and $\vec E$ field everywhere; no Faraday's law required. Since $E'_y = vB$, we can recover the voltage $\mathcal{E} = vBL$ as previously found. Note from this calculation we are actually measuring $\vec E'$ in the moving frame.
Lastly, the result from applying the Leibniz integral rule:
$$\begin{align}
\vec \nabla \times ( \vec E + \vec v \times \vec B) &= - {\partial \vec B \over \partial t} \\
\vec \nabla' \times ( \vec E' ) &= - {\partial \vec B' \over \partial t} \\
\end{align}$$
is the same as applying the field transformations for E and B.



