Inductance - Sign convention problem

Question

My problem is with a circuit containing a resistor of suitable resistance $R$ and a solenoid. Initially, a battery is connected which generated a current in the circuit and at $t=0$, the battery is disconnected from the circuit.

Now I need to find the current as a function of the time $i(t)$, for which I've used Kirchhoff's Voltage Law.

I know that the voltage drop across the resistor is $V=IR$, and the back EMF induced in the solenoid is $V=-L\frac{di}{dt}$ where $L$ is the self-inductance of the coil.

Now, I don't know how to determine the sign of voltage across the solenoid while traversing the loop. I mean, whether it's positive or negative..?

Answer 1

Actually, for electric circuits, the voltage across an inductor is

$v_L = L \dfrac{di_L}{dt}$

By the passive sign convention, the inductor current enters the terminal that is the more positive when $v_L>0$.

Here's what you do. Pick a direction for the inductor current by drawing an arrow pointing into one of the terminals. Label that arrow $i_L$. Now, place a plus sign at the terminal the current enters and a minus sign at the other terminal. This is the polarity of the inductor voltage $v_L$.

By the formula above, if the inductor current is increasing with time, $v_L>0$. If the inductor current is decreasing with time, $v_L < 0$.

You can't pick the "wrong" direction or polarity. The signs will take care of themselves.

Is you problem similar to this one?

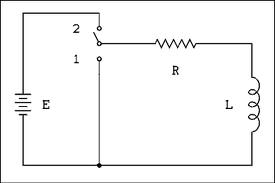
When the switch has been in position 2 for a long time, the series electric current is constant and clockwise through the resistor, inductor, and source. Since there is only one current, the series current, let's just label that $i$ and have the arrow pointing into the left end of the resistor.

According to the passive sign convention, the polarity of our voltage variables, $v_R, v_L$ have the positive sign at the left end of the resistor and the top end of the inductor.

Since the current is constant, $v_L = 0$, thus $i=E/R$ and $v_R = E$ for $t<0$.

If at $t=0$ the switch is (instantaneously) changed to position 1, The current through the inductor must be same at $t=0+$ as at $t=0-$, i.e., the current through the inductor is continuous.

With the same current as before, the voltage across the resistor $v_R(0+) = E$. But now, this means that $v_L(0+) = -E$ by KVL (sum of voltage drops around a closed loop is zero). This implies that the current through the inductor is decreasing (negative time rate of change) which is what you should expect!

We have the initial condition, $i(0+) = E/R$, and the form of $i(t)$ is the well known decaying exponential:

$i(t) = \dfrac{E}{R}e^{-\frac{t}{\tau}}, \tau = \dfrac{L}{R}, t>0$

Answer 2

If you want to go more in depth. The negative sign in the equation, $v(t) = -L\frac{\mathrm {d}i}{\mathrm{d}t}$ comes from the assumption of active convention, not passive convention. In a voltage source the electromagnetic field comes from the positive end of the voltage source. Therefore, it re-enters the voltage source from the negative end and comes back into the circuit from the positive end.

Then the entry point is negative and the exit point is positive so the energy or the work done by the electromagnetic field depends on the final voltage minus the initial voltage. Since the initial voltage is negative and a negative number multiplied by a negative number is positive, the final voltage of the voltage source is positive. In this case, the negative sign does apply because current creates an induced magnetic field in the inductor.

For instance, if the current is increasing then the magnetic field wants to increase in the direction of the current, but the inductor doesn't like that (look up right hand rule for more explanation). Therefore, it creates an induced magnetic field in the opposite direction. Because the negative derivative of the change in magnetic flux (the area of the magnetic field between the loops of the inductor) with respect to time is equal to voltage, the voltage is negative in the inductor. This derivative is actually the same as $-L\frac{\mathrm{d}i}{\mathrm{d} t}$ except the derivative of magnetic flux is multiplied by the number of loops in the inductor.

If you assume the passive convention, then you don't need to worry about the negative sign because the voltage source would be negative and the inductor voltage would be positive so the current across a resistor, for instance, in a Resistor/Inductor Circuit would gradually increase since the inductor "holds" the voltage back from the resistor. In other words negative voltage from the source plus positive voltage from the inductor (using Kirchoff's Voltage Law) creates a smaller voltage in the resistor.

I hope this satisfies your curiosity.