0
$\begingroup$

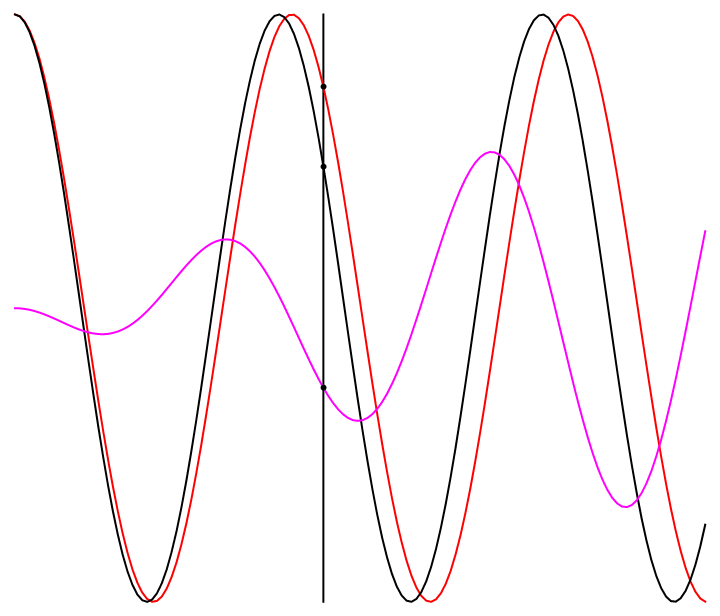
Does anybody get the answer the book gives for this?

Decades ago, I worked through most of the problems in the first part of the original exercise manual for the Feynman Lectures on Physics. It was exceedingly rare that the answer in the book was wrong. I realize that this is a hand-corrected OCR transcription, so it's possible that errors crept in.

Nonetheless past experience tells me that I may be overlooking something subtle (or obvious).

This is Exercise 17.5 in Exercises for the Feynman Lectures on Physics.

Two particles $A$ and $B$ execute harmonic motion of the same amplitude ($10cm$) on the same straight line. For particle $A$, $\omega_{A}=20\text{rad}\text{s}^{-1};$ for $B$, $\omega_{B}=21\text{rad}\text{s}^{-1}.$ If at $t=0$, they both pass through $x=0$ in the positive $x$-direction (hence are then "in phase"),

(a) How far apart, $\Delta x,$ will they be at $t=0.350\text{s}?$

(b) What is the velocity $V$ of $B$ at $t=0.350\text{s}?$

Part (a):

Writing the expressions for position

$x_{A}\left[t\right]=\alpha\cos\left[\omega_{A}t\right]=10\cos\left[20\times0.350\right],$

$x_{B}\left[t\right]=\alpha\cos\left[\omega_{B}t\right]=10\cos\left[21\times0.350\right].$

Calculate the magnitude of their differences

$\Delta x=\left|x_{B}\left[t\right]-x_{A}\left[t\right]\right|=10\left|\cos\left[7.35\right]-\cos\left[7.00\right]\right|$

$\Delta x=2.71\text{cm}.$

The answer in the book is $\Delta x=2.18\text{cm}.$

Part (b):

$V_{A}\left[t\right]=-\alpha\omega_{A}\sin\left[\omega_{A}t\right],$

$V_{B}\left[t\right]=-\alpha\omega_{B}\sin\left[\omega_{B}t\right].$

$V=V_{B}\left[t\right]-V_{A}\left[t\right]=-\alpha\omega_{B}\sin\left[\omega_{B}t\right]-\left(-\alpha\omega_{A}\sin\left[\omega_{A}t\right]\right)$

$=\alpha\left(\omega_{A}\sin\left[\omega_{A}t\right]-\omega_{B}\sin\left[\omega_{B}t\right]\right)$

$V=-52.49\text{cm}\text{s}^{-1}.$

The answer in the book is $V=-49.4\text{cm}\text{s}^{-1}.$

The first attached diagram shows a plot of position versus time. The horizontal line represents $t=0.35$. The magenta curve represents the difference of the positions.

The second graphic depicts the velocities.

enter image description here enter image description here

Improve this question
$\endgroup$
9
  • $\begingroup$ "Homework-like questions should ask about a specific physics concept and show some effort to work through the problem. " I'm 50% off this site already. $\endgroup$
    – Steven Thomas Hatton
    Commented Jan 15, 2018 at 5:09
  • $\begingroup$ Please explain how my post did not demonstrate an effort to solve the problem, or how it failed to ask about a specific physical concept. Had I expended the same time and effort solving the problem, as I did posting the question, I would not have needed to post the question. $\endgroup$
    – Steven Thomas Hatton
    Commented Jan 15, 2018 at 5:18
  • $\begingroup$ i DO NOT NEED YOUR HEPL! $\endgroup$
    – Steven Thomas Hatton
    Commented Jan 15, 2018 at 5:18
  • 1
    $\begingroup$ A procedural note: questions cannot be deleted if they have answers that are accepted or have upvotes. (Generally, if you ask questions here you are soliciting others to spend their time answering them; removing the question also deletes its answers and it is unfair to the people who have worked to write those posts.) $\endgroup$
    – Emilio Pisanty
    Commented Jan 15, 2018 at 13:26
  • 1
    $\begingroup$ In this specific case there is no need to delete the post; it is simply closed and we can all move on. Simply take home the message that worked-example questions just aren't a good fit for this site, and this is a clear example of the kinds of questions that we don't take. If you want to ask about a given method then do that, with a possible (incidental!) reference to the problem at hand. This question, instead, is simply asking for a validation for the solution of a set-piece, and we don't do those types of questions. $\endgroup$
    – Emilio Pisanty
    Commented Jan 15, 2018 at 13:28

1 Answer 1

Reset to default
1
$\begingroup$

I think the answer lies in your solution for the distance as function as time. We are told that at time t=0 both are at the origin, this means that the motion should be described by a sine function and not a cosine function. I hope this helps.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.