How does one describe how a basis vector changes through space using the Christoffel symbols?

Question

I have been watching this video, which explains in fair detail what each of the terms in Einstein’s field equation represents. The portion I linked explains the tensor object ${\Gamma^\alpha}_{\beta\gamma}$, which I believe is also called a Christoffel symbol.

To summarize, if spacetime is curved or if a coordinate system is curved, then the basis vector in a particular dimension is not necessarily constant throughout space. The change in the basis vector along a dimension can be described as a linear combination of all the coordinate system’s basis vectors.

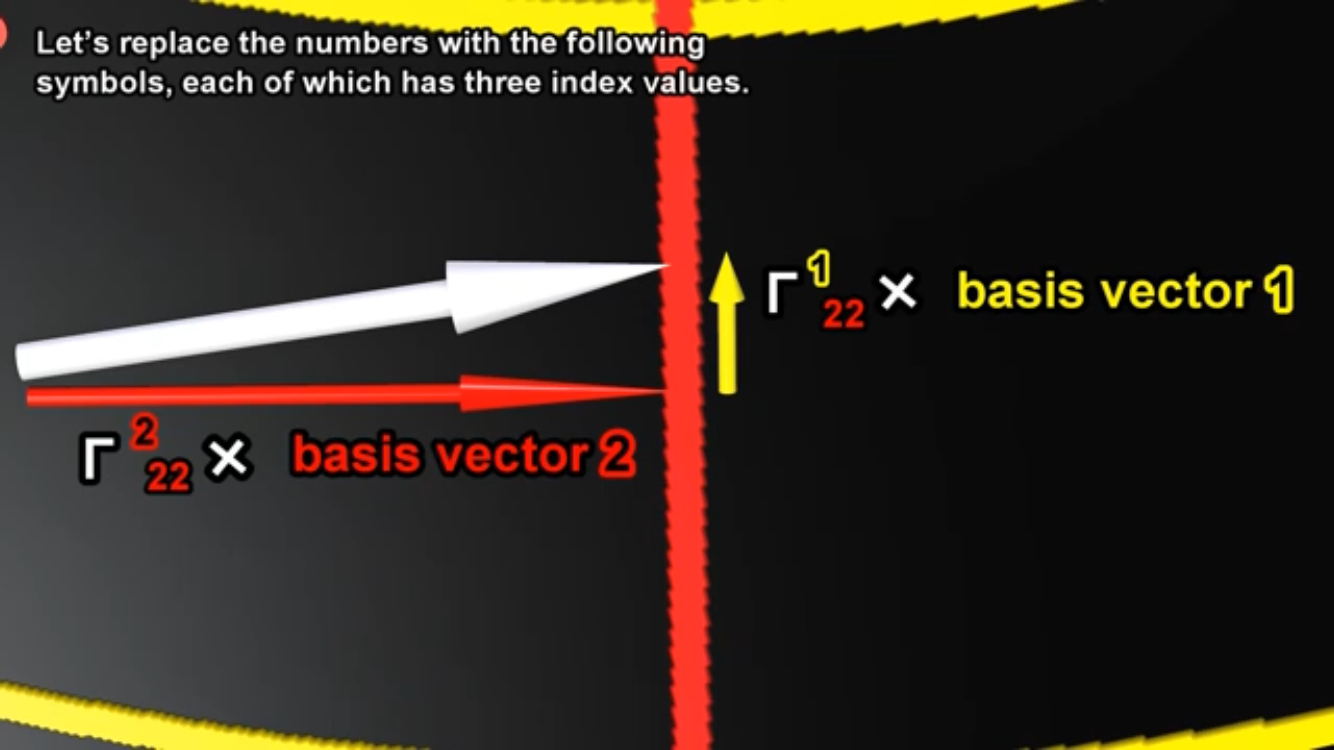
The video indicates that for the tensor object ${\Gamma^\alpha}_{\beta\gamma}$,

• $\alpha$ indicates which basis vector it is multiplying;
• $\beta$ indicates which basis vector is ‘moving’ or being examined; and
• $\gamma$ indicates the direction in which the basis vector (of the $\beta$-dimension) is moving.

I would like to understand how the difference in a basis (covariant) vector $\tilde e_\mu$ between two points in space, $\vec a = \begin{pmatrix} x_a & y_a & z_a \end{pmatrix}^\mathsf{T}$ and $\vec w = \begin{pmatrix} x_w & y_w & z_w \end{pmatrix}^\mathsf{T}$, is mathematically described. (Note that $\tilde e_\mu$ is not the component of $e$ in the $\mu$-dimension but rather the basis vector $\tilde e$ of the $\mu$-dimension.)

I figured that one could define $$\tilde e_\mu(\vec a) + \Delta\tilde e_\mu = \tilde e_\mu(\vec w)$$

These are my particular thoughts:

• For three-dimensional space, one must sum over $\alpha\in\{1,2,3\}$.
• If one is investigating the change in $\tilde e_\mu$, then one should set $\beta=\mu$.
• Since $\vec w - \vec a$ could span any number of dimensions, then I suppose that one ought to sum over $\gamma\in\{1,2,3\}$.

This leads me to my best guess that $$\Delta\tilde e_\mu = \sum_{\gamma=1}^{3} \sum_{\alpha=1}^{3} {\Gamma^\alpha}_{\mu\gamma} \, \tilde e_\alpha$$

Naturally, one would also have to take into account that ${\Gamma^\alpha}_{\beta\gamma}$ could vary across space, so this should probably look more like $$\Delta\tilde e_\mu = \sum_{\gamma=1}^{3} \sum_{\alpha=1}^{3} {\Gamma^\alpha}_{\mu\gamma}(\vec a,\vec w) \, \tilde e_\alpha$$

I have the presentiment that one does not define actually ${\Gamma^\alpha}_{\beta\gamma}$ as a function of two vectors but rather resolves the issue of ${\Gamma^\alpha}_{\beta\gamma}$ varying across space by integrating along the path from $\vec a$ to $\vec w$.

How close am I to correct? How is this actually done in practice?

Answer 1

So, assume that you have a set of basis vectors, call them $\mathbf{e}_{\mu}$. One actually defines the connection coefficients as the components of the directional derivative of the basis vectors:

$\nabla_{e_v} e_u \equiv \Gamma^{\alpha}_{\mu v} e_{e_\alpha}$

So, how you read this is: the connection coefficients $\Gamma^{\alpha}_{\mu v}$ is the $\alpha$-component of the change of $e_{\mu}$ by a change in the $e_{v}$ direction, so I would say your formulation is correct. One then uses this definition along with properties of the Koszul connection to define the covariant derivative in a coordinate-independent manner.

(Also, just a comment: the general definition of a basis vector: $e_{\mu} = \frac{\partial}{\partial x^{\mu}}$ is valid in general (flat/curved) spacetimes)