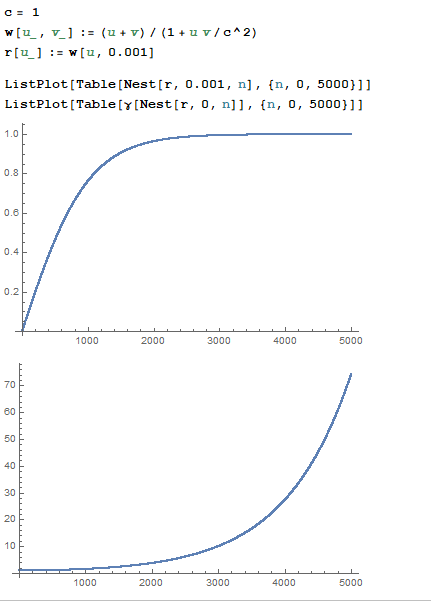
LINK : My answer as user82794 (former diracpaul) here : Inertia on relativistic mass when particle is near speed of light.
A Non-Relativistic Interpretation of Relativistic Results
(warning : all stuff here is old-fashioned, but gives you the answers)
When self-studying special relativity, an exercise was created in order to understand non-relativistically the relativistic result that a force could not accelerate a particle to speed greater than $\:c\:$. The exercise, the Figure and the solution are already in LaTeX and are copy-paste here. May be is useful.
The solution to the following exercise yields a non-relativistic interpretation of the relativistic result that energy $\:E\:$ has inertia, that is resistance to be accelerated, as material objects. The inertia of material objects is expressed by their inertial mass $\:m\:$ while the inertia of energy $\:E\:$ is expressed by its mass equivalent$\: m_{E}=E/c^{2}\:$. Also, based on this interpretation, an explanation of the impossibility of a force to accelerate an object to velocity greater than that of light ($c$) is given.
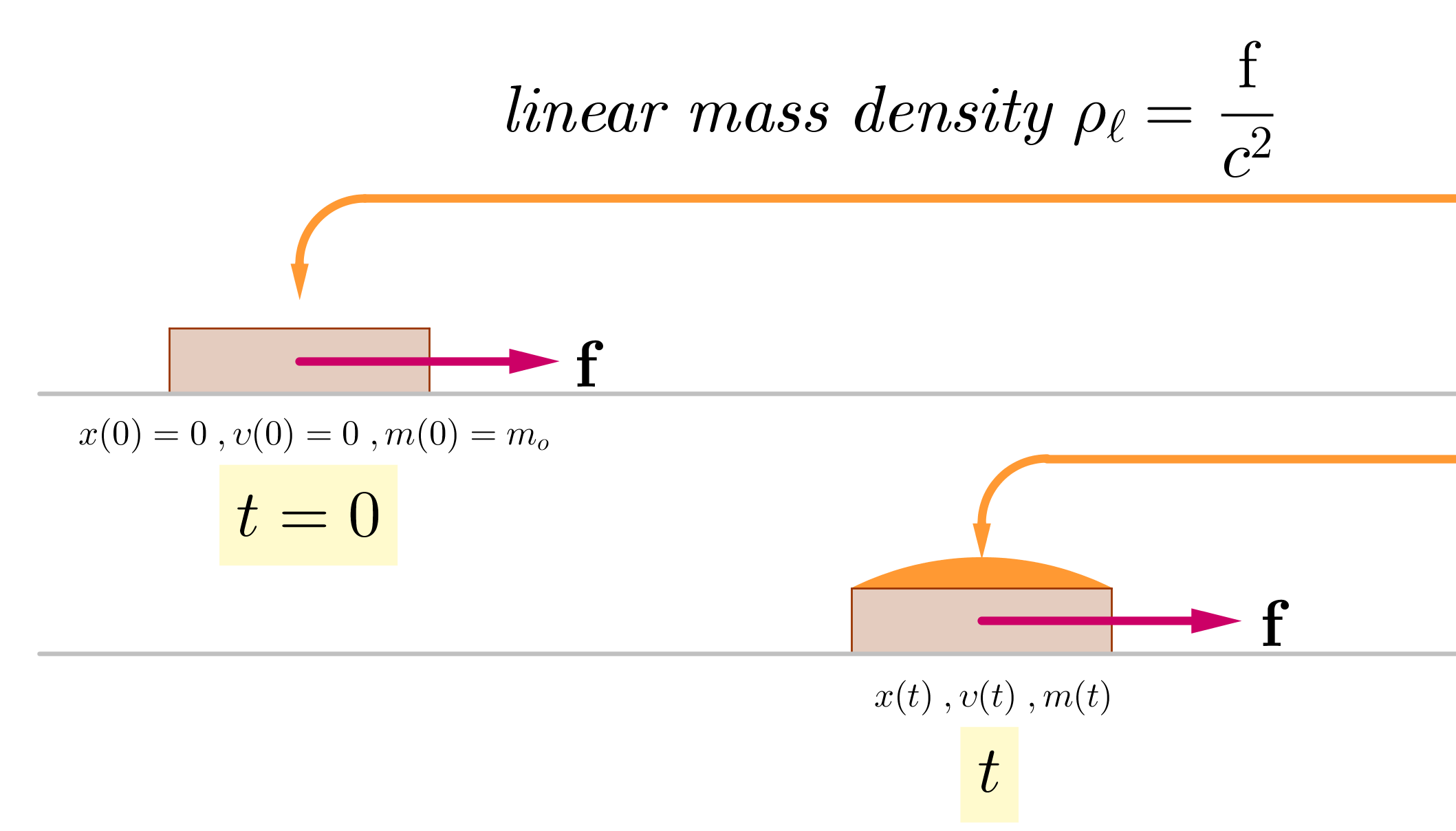
A body of mass $\:m_{o}\:$ initially at rest is moving on a surface without friction under the influence of a constant force $\:\mathbf{f}\:$, as in Figure above. As it is moving, it is carrying away material from a straight line above it. The straight line has a constant linear mass density $\:\rho_{\ell}=f/c^{2}\:$, where $\:\rm{f}\:$ the magnitude of the constant force $\:\mathbf{f}\:$ and $\:c\:$ a quantity with dimensions of speed.
The exercise concerns the determination of the following :
(a) the position $\:x(t)\:$, the velocity $\:\upsilon(t)\:$ and the mass $\:m(t)\:$ as functions of time.
(b) any explicit relation, if there exists, between above quantities.
Answers :
(for a proof see in the link above)
Position $\:x(\upsilon)\:$ as function of speed $\:\upsilon$ :
\begin{equation}
x\left(\upsilon\right)=\dfrac{m_{o}c^{2}}{f}\left( \dfrac{1}{\sqrt{1-\dfrac{\upsilon^{2}}{c^{2}}}}-1\right)
\tag{01}
\end{equation}
Speed(1)$\:\upsilon(x)$ as function of position $\:x$ :
\begin{equation}
\upsilon\left(x\right) = c \sqrt{1-\dfrac{1}{\left(1+\dfrac{f}{m_{o}c^{2}}x\right)^{2}}}
\tag{02}
\end{equation}
Position(2)$\:x(t)\:$ as function of time $\:t$ :
\begin{equation}
x\left(t\right)=c \left[\sqrt{t^2+\left(\dfrac{m_{o}c}{f}\right)^{2}}-\left(\dfrac{m_{o}c}{f}\right)\right]
\tag{03}
\end{equation}
Speed $\:\upsilon(t)$ as function of time $\:t$ :
\begin{equation}
\upsilon\left(t\right)=c \sqrt{1-\dfrac{1}{\left(\dfrac{f}{m_{o}c}t\right)^2+1}}
\tag{04}
\end{equation}
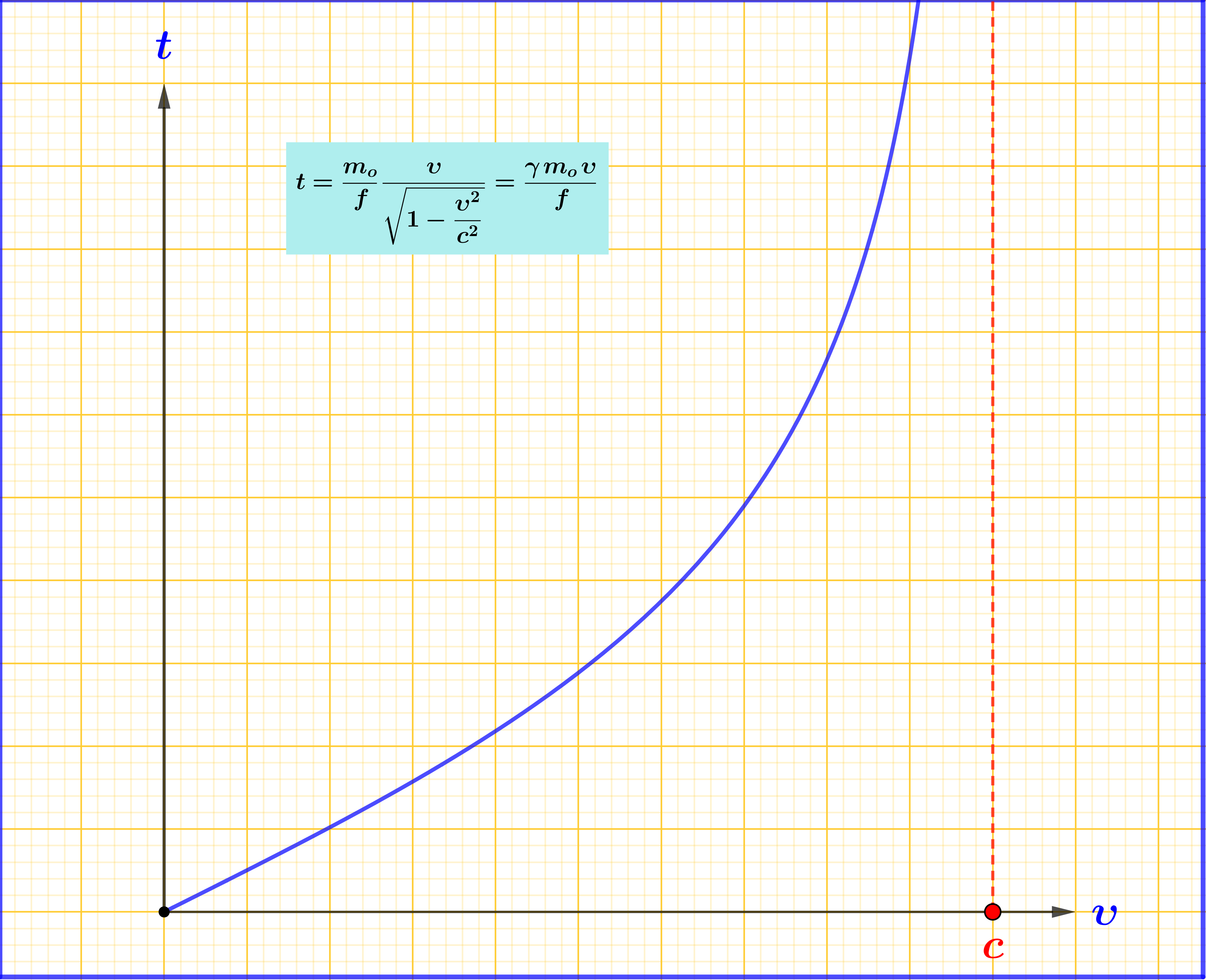
From (04) the time needed to accelerate the body from rest to speed $\:\upsilon$ is :
\begin{equation}
\boxed{\:\:t=\dfrac{m_{o}}{f}\dfrac{\upsilon}{\sqrt{1-\dfrac{\upsilon^{2}}{c^{2}}}}=\dfrac{\gamma\,m_{o}\,\upsilon}{f} \:\: \vphantom{\dfrac{\frac12}{\sqrt{1-\dfrac{\upsilon^{2}}{c^{2}}}}}}
\tag{05}
\end{equation}
Equation (05) is expressed as :
\begin{equation}
\dfrac{f\,t}{m_{o}\,c}=\dfrac{\upsilon}{c\sqrt{1-\dfrac{\upsilon^{2}}{c^{2}}}}=\dfrac{\gamma\,\upsilon}{c}=\sqrt{\gamma^{2}-1}
\tag{06}
\end{equation}
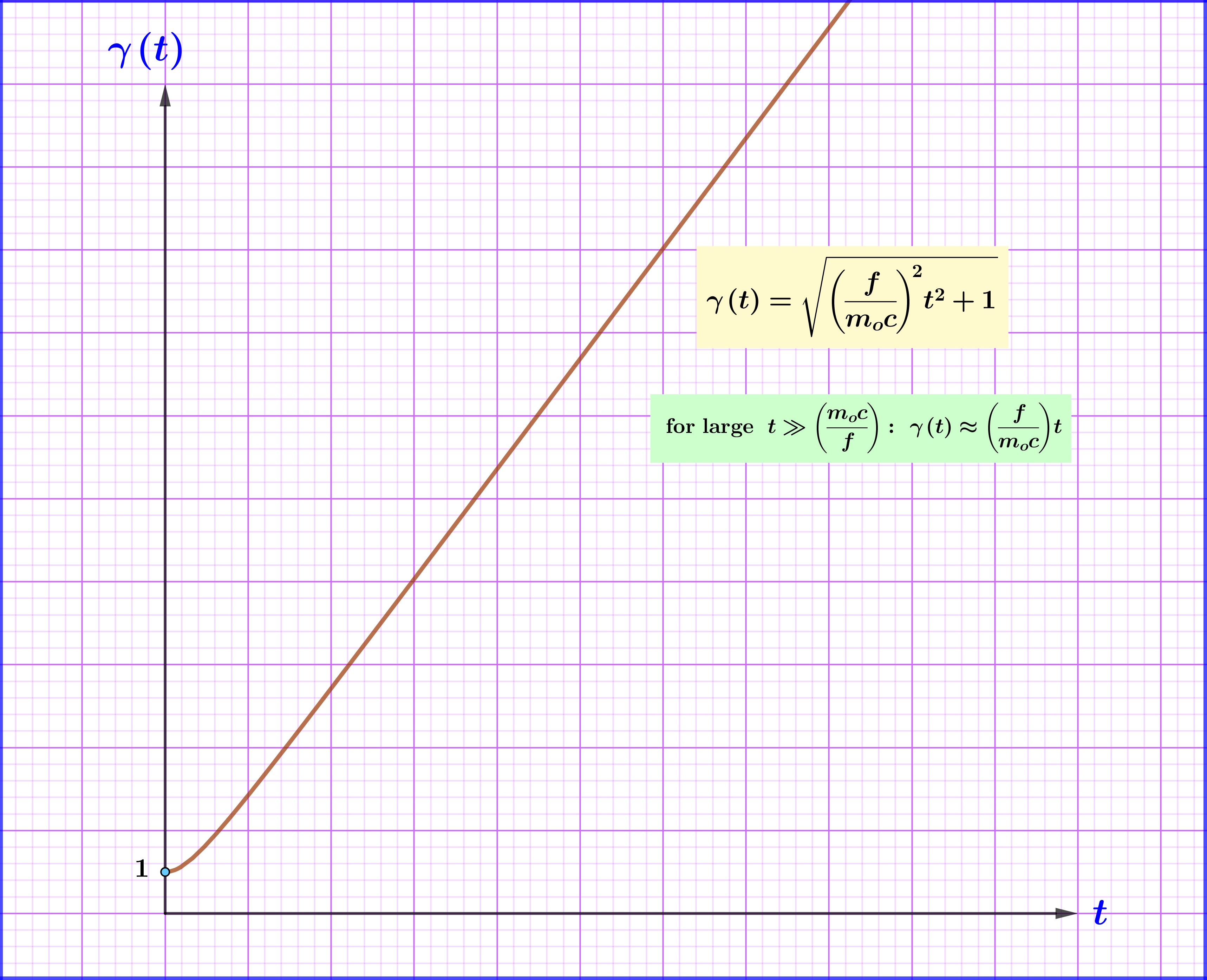
From (06), or directly from (04)
\begin{equation}
\boxed{\:\:\gamma\left(t\right)=\sqrt{\left(\dfrac{f}{m_{o}c}\right)^{\!2}\!t^{2}+1}\:\: \vphantom{\dfrac{\dfrac12}{\dfrac12}}}
\tag{07}
\end{equation}
For the proper time $\:\tau\left(t\right)\:$ as function of the time $\:t\:$ we have
\begin{equation}
\mathrm d \tau=\dfrac{1}{\gamma\left(t\right)}\,\mathrm d t =\dfrac{1}{\sqrt{\left(\dfrac{f}{m_{o}c}\right)^{\!2}\!t^{2}+1}}\,\mathrm d t
\tag{09}
\end{equation}
and under the condition $\:\tau\left(0\right)=0\:$
\begin{equation}
\tau\left(t\right) =\int\limits_{0}^{t}\mathrm d \tau=\int\limits_{0}^{t}\dfrac{1}{\gamma\left(t\right)}\,\mathrm d t =\int\limits_{0}^{t}\dfrac{1}{\sqrt{\left(\dfrac{f}{m_{o}c}\right)^{\!2}\!t^{2}+1}}\,\mathrm d t =\dfrac{m_{0}c}{f}\sinh^{-1}\left(\dfrac{f}{m_{o}c}\,t\right)
\tag{10}
\end{equation}
that is
\begin{equation}
\boxed{\:\:\tau\left(t\right) =\dfrac{m_{0}c}{f}\sinh^{-1}\left(\dfrac{f}{m_{o}c}\,t\right)\:\: \vphantom{\dfrac{\dfrac12}{\dfrac12}}}
\tag{11}
\end{equation}
and inversely
\begin{equation}
\boxed{\:\:t\left(\tau\right) =\dfrac{m_{0}c}{f}\sinh\left(\dfrac{f}{m_{o}c}\,\tau\right)\:\: \vphantom{\dfrac{\dfrac12}{\dfrac12}}}
\tag{12}
\end{equation}
Inserting this expression of $\:t\:$ in (04) we have the following function $\:\upsilon \left(\tau\right)\:$, that is the speed as function of the proper time $\:\tau$
\begin{equation}
\boxed{\:\:\upsilon \left(\tau\right) =c\tanh\left(\dfrac{f}{m_{o}c}\,\tau\right)\:\: \vphantom{\dfrac{\dfrac12}{\dfrac12}}}
\tag{13}
\end{equation}
Mass(3) $\:m(t)$ as function of time $\:t\:$ and mass $\:m(\upsilon)\:$ as function of $\:\upsilon$ :
\begin{equation}
m\left(t\right)=\sqrt{m_{o}^{2}+\left(\dfrac{f t}{c}\right)^{2}}=\dfrac{m_{o}}{\sqrt{1-\dfrac{\upsilon^{2}}{c^{2}}}}=m(\upsilon)
\tag{14}
\end{equation}
From above equations we have :
\begin{equation}
\lim_{t\rightarrow +\infty}x\left(t\right) = +\infty
\tag{15}
\end{equation}
\begin{equation}
\lim_{t\rightarrow +\infty}\upsilon\left(t\right) = c \;,\quad \upsilon\left(t\right) < c
\tag{16}
\end{equation}
\begin{equation}
\lim_{t\rightarrow +\infty}m\left(t\right) = +\infty
\tag{17}
\end{equation}
The force could not accelerate the particle to speed greater than $\:c\:$ because its work is feeding continuously back as mass (inertia) to this particle.
(1)
Equation (02) is identical to
\begin{equation}
v=c\sqrt{1-\left(\dfrac{m_0 c^2}{F\cdot x + m_0 c^2}\right)^{2}}
\end{equation}
given without proof by @jaromrax here : Is there a formula that gives the position of an object depending on the time, but which doesn't allow the object to surpass the speed of light?.
(2)
Equation (03) is identical to
\begin{equation}
x(t) = \frac{c^2}{\alpha}\left[\sqrt{1 + \frac{\alpha^2 t^2}{c^2}}-1 \right]\;,\; t \ge 0
\end{equation}
given without proof by @Alfred Centauri in the same link here : Is there a formula that gives the position of an object depending on the time, but which doesn't allow the object to surpass the speed of light?. Simply replace
\begin{equation}
\alpha = \dfrac{f}{m_{0}}
\end{equation}
(3)
In Modern Physics there exists only the mass at rest $\:m_{o}\:$, while the $^{\prime\prime}$motional mass$^{\prime\prime}$ $\:m\:$ is not used any more. In above answer think of $\:m(t)$ and $\:m(\upsilon)\:$ as auxiliary functions that make elaboration easier.