1
$\begingroup$

I am unable to clearly understand the meaning of fitting the data. Like in this paper of determination of mass and width of Z boson and number of neutrinos (from ALEPH detector)

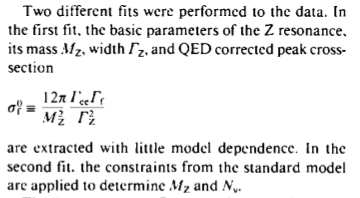
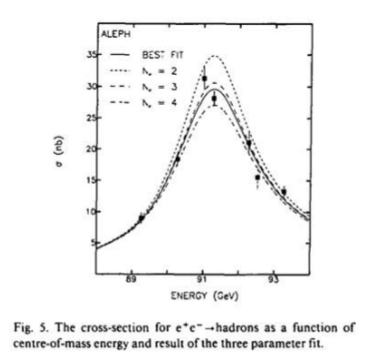
enter image description here

Does it mean that that we draw the lineshape using theory and taking into account xyz parameters/constraints?

As here one of them says taking little model dependence. So what does it mean?

Also, what does it mean when I say two parameter fit or three parameter fit in this case?

enter image description here

Improve this question
$\endgroup$

1 Answer 1

Reset to default
4
$\begingroup$

When one has a resonance, particularly one never seen before , one starts by using the data left and right of the resonance as a background extrapolated under the resonance so as to get the experimental significance of the signal. An example is the Higgs discovery:

higs

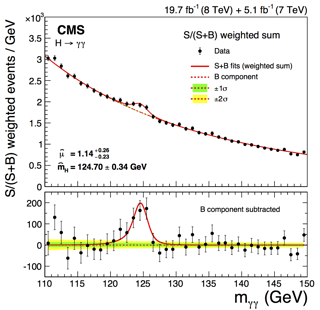
Combined diphoton mass spectrum illustrating the significance of the observed excess, where events are weighted by the expected signal-to-background ratio. The corresponding background-subtracted distribution is shown in the lower panel.

This is a plot that establishes the existence of a resonance, but cannot be compared to theory because of the measurement errors , the width in this example should be of the order of MeV,not GeV, the experimental errors have to be unfolded.

The use of Monnte Carlo events is necessary to fit the data.

In the Monte Carlo the events are generated according to the theory one wants to fit. Events are generated according to the errors in the various detectors and one can unfold the true shape of the resonance.

It also uses the kinematics of the theory under consideration, according to various parameters of the standard model to be tested .

That is the minimal way of fitting the Z (in your question), "little model dependence".

The second method is using two parameter fit with variations of the standard model (3 neutrino species or 4) as a constraint, reducing the number of free parameters, because of wanting to find the dependence on the number of neutrinos, the two free parameters are the mass of the Z and the number of neutrinos. This means at least two different Monte Carlo data sets in the region of the Z.

edit after comment:

This is how the number of neutrinos was determined by trying to fit with open parameters the crossection and the number of neutrinos, using the previously fitted parameters of the standard model except these two.

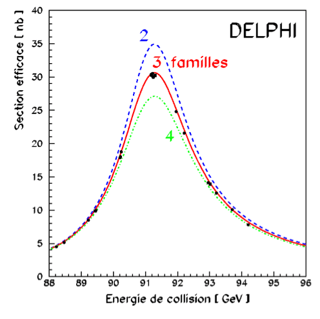
numnu

The black dots are the data, the hashed lines the best fits to the data for each hypothesis.

Improve this answer
$\endgroup$
6
  • $\begingroup$ Sir, could you explain the last paragraph again... that is about two parameter and three parameter fit..(like which are those two or three parameters here...I guess two of them are $m_Z$ and $N_\nu$ as you mentioned. So what is the third parameter?) $\endgroup$
    – kbg
    Commented Nov 6, 2017 at 20:32
  • 1
    $\begingroup$ When one is doing a fit in a complex problem some parameters are left free to be determined by the fit. Normally for fitting the Z or the H or ... one leaves as free the first (called little model dependence, i.e. it is mostly kinematics). To determine whether there are two or three or four neutrinos, the values of all parameters were fixed by the standard model except for the crossection and then three different models were applied, with two neutronos three or four . $\endgroup$
    – anna v
    Commented Nov 7, 2017 at 4:38
  • $\begingroup$ Sir, I have added a plot in the question. Does, it mean that except $m_z,N_\nu$ and peak cross section, all other factors (including decay width of z to leptons and decay width of z to $\nu\bar{\nu}$ was taken from the standard model? $\endgroup$
    – kbg
    Commented Nov 9, 2017 at 8:09
  • $\begingroup$ I have included that plot in my answer in an edit after my last comment. Yes, they use the standard model to predict the differences if the theory had two, three, or four neutrinos, with free parameters only the numberof neutrinos and the total crossection of the Z; and the best fit is the three. $\endgroup$
    – anna v
    Commented Nov 9, 2017 at 14:36
  • 1
    $\begingroup$ Have a look at this cds.cern.ch/record/2217139/files/9789814733519_0008.pdf , elaborate fits use all channels, it is just that the hadronic channels are dominant and that the number of neutrinos affect the peak crossection. $\endgroup$
    – anna v
    Commented Nov 9, 2017 at 15:46

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.