0
$\begingroup$

So, this is a question from a book I've been solving,

A car is moving with a uniform speed of 40km/h towards a vertical wall where it'll take a 90 degree turn. When the car is 20km away from the turn, a fly flies towards the car with a uniform speed of 100km/h, after touching the car it flies back to the wall. It continues to do so till the car reaches the wall. Find 1)the total distance travelled by the fly. 2)Number of trips it makes.

I was able to solve the first part,

time taken by the car to reach the wall = $\frac{20km}{40{km\over h}}=\frac{1}{2}h$

dist. travelled by the fly in that time $= 100\frac{km}{h}*\frac{1}{2}h=50km$

Now for the 2nd part; 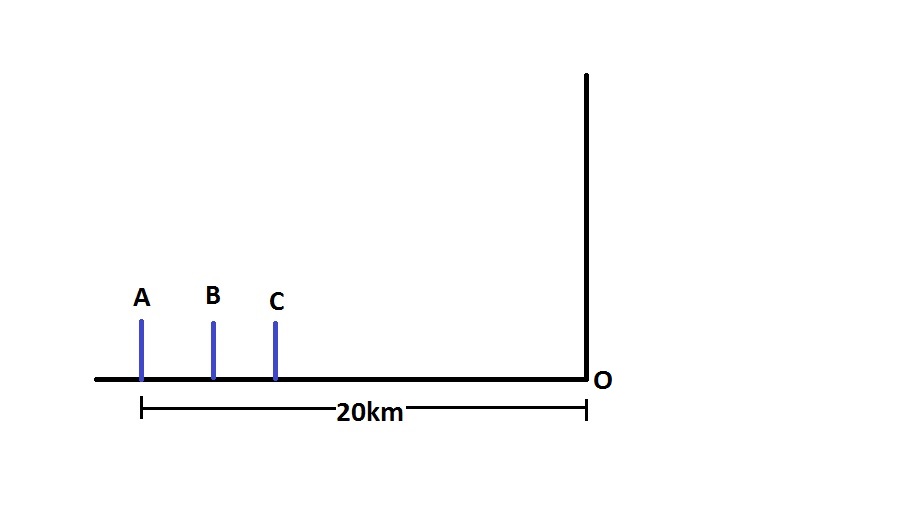enter image description here The car is at A when the fly starts moving towards it. They meet at B after time $t$.

$\Rightarrow$ AB$=40t$ and OB$=100t$

$\Rightarrow$OA=AB+OB

$\Rightarrow$$20=40t+100t$

$\Rightarrow$$t={1\over7}h$

$\Rightarrow$OB$={100\over7} km$

Now time taken by the fly to reach back the wall will be $t'={{100\over7}km\over100{km\over h}}={1\over7}h$. Now the car is at C.

$\Rightarrow$OC=OB-BC=$\frac{100-40}{7}km=\frac{60}{7}km$

Now, distance of car from the wall at the beginning of the :

1st trip=$20km$

2nd trip=$\frac{60}{7}km=\frac{3}{7}*20km$

3rd trip=$(\frac{3}{7})^2*20km$

nth trip=$(\frac{3}{7})^{(n-1)}*20km$

At nth trip, the car will be zero distance away from the wall. i.e. $(\frac{3}{7})^{(n-1)}*20km=0$

Now the book says that the fly will make an infinite no. of trips before the car makes the turn.

My question is that how is that possible?! The last equation has no solution. since a non-zero number raised to any power will never give zero.

Also, the distance the car travells is finite so how can the fly make an infinite number of trips?

Improve this question
$\endgroup$
3
  • $\begingroup$ You end up with $x^a = 0$, think about $\lim_{x \to \infty} x^a$ where x is less than 1 $\endgroup$
    – user95137
    Commented Oct 3, 2017 at 0:38
  • $\begingroup$ You should come to the realisation that one of your statements near the end of your question is wrong $\endgroup$
    – user95137
    Commented Oct 3, 2017 at 0:38
  • $\begingroup$ A number less than one when raised to infinite power gives zero. $\endgroup$
    – Rajendra Pd
    Commented Oct 3, 2017 at 0:54

1 Answer 1

Reset to default
0
$\begingroup$

First I'll try to explain it without any equations. Imagine when the car is a few metres away, the fly can rapidly fly back and forth and touch the car many number of times. If you can visualise that, then fine. Now coming to your equation, the answer given is correct according to your equation. Any number less than 1 raised to the power infinity is zero. I'll try to explain it to you. 3/7 = 0.428, (3/7)^2 = 0.183, (3/7)^3 = 0.078. As you can see, for any fraction less than 1 the value approaches zero as the power keeps increasing so when n (given) is infinity the value is zero

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.