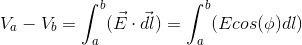Electric potential from integration sign changes

Question

I'm having problems with the sign of the electric potential in this situation.

If given that

If I choose a path from point A to point B (in the figure below), the angle between the electric field and the path of integration is $0$. Continuing with this integration gives $E(b-a) = -Ea$. (This is because the bottom plate is y = 0)

However, if I choose a path from point B to point A, the angle between the electric field and the path of integration becomes $\pi$. This also gives the same as the integration before, $-Ea$.

I understand that I am introducing one negative from flipping the path of integration and another negative from the cosine, which is why they cancel. But I know they should be opposite in sign if I am reversing the path, but I do not know what I am doing wrong.

Help is really appreciated! Thank you!

Answer 1

The potential difference between two points is minus the work done by the electric field on unit positive charge in going from one point to the other.

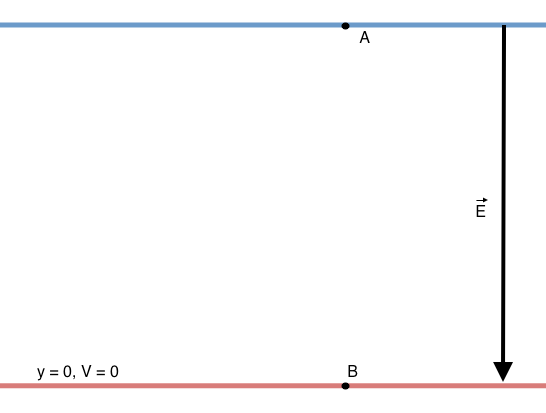
Let the distance between $A$ and $B$ be $L$ as shown in the diagram.

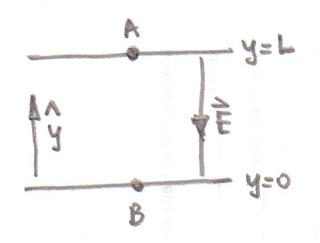
$\vec E = -E\, \hat y$ and $d\vec l = dy \, \hat y$ where $-E$ and $dy$ are components of the electric field and displacement in the $\hat y$ direction.

Minus the work done by the electric field after undergoing a displacement $d\vec l$ is

$-(\vec E \cdot d\vec l) =-(-E\,\hat y \cdot dy\, \hat y) = + E\,dy$

Note that no choice has been made about the direction of the total displacement.

Going from $B$ to $A$

$\displaystyle V_{\rm A} - V_{\rm B} = - \int ^A _B \vec E \cdot d\vec l = \int ^L _0 E\,dy = +EL$

Going from $A$ to $B$

$\displaystyle V_{\rm B} - V_{\rm A} = - \int ^B _A \vec E \cdot d\vec l = \int ^0 _L E\,dy = -EL$

Now perhaps the integrations are masking what is happening so because the electric field is constant I can write that minus the work done by the electric field $\vec E$ in undergoing a displacement of $\Delta \vec l$ from $B$ to $A$ is $- \vec E \cdot \Delta \vec l$.

$\vec E = - E \, \hat y$ as shown in the diagram and $\Delta \vec l = +L\,\hat y$ when going from $B$ to $A$.

and so minus the work done by the electric field is $-(- E \, \hat y \cdot (+L\,\hat y)) = +EL$

Going the other way from $A$ to $B$ the electric field stays the same but now the displacement $\Delta \vec l = -L \, \hat y$ and so minus the work done by the electric field is $-EL$.

So when you integrate the limits of the integration give you the direction of travel and in effect decide whether the incremental steps are positive (in the $\hat y$ direction) or negative (in the $-\hat y$ direction).

With your introduction of $\cos \phi$ you were in effect choosing the direction of travel for the test charge and then in one of you integrals interposing a different direction of travel by your choice of limits of integration.