I have done enough research on this to know that the equations for converting source output energy to absorbed dose in humans are too complex for me to use.
For the purposes of estimation, is rad linearly proportional to energy of the radiation?
In other words, if I know that 0 Joules from a source elicits 0 rad in a human and 450 Joules elicit 600 rad, could I assume that 75 Joules elicits 600 rad?
It depends on how the total energy emitted is being increased.
If the amount of energy is increased by exposing the target for a longer period of time, then the dose absorbed is linearly proportional. Twice the time means twice the dose.
If the amount of energy is increased by increasing the intensity of the source, perhaps by adding more radioactive substance, then the dose is linearly proportional. Twice the mass of cobalt-60 results in twice the dose rate.
If the energy is increased by increasing the energy of the radiation, then the resulting dose gets complicated. Increasing the energy of X-rays can result in a larger dose if all of the photons are absorbed. However, higher energy X-rays penetrate more deeply into the target, which means they lose less energy than lower energy photons.
The graph above shows the attenuation length (what distance reduced the intensity by $1/e$) as a function of the original photon energy. This length in the graph is scaled by the density of water for historical reasons. As the energy of the photon goes up, it takes more and more distance to stop it. Notice that this is not a simple function.
In medical X-rays, radiologists will take advantage of this effect to create X-ray beams that deposit most of their dose beneath the skin of the patient. This effect is called depth dose.
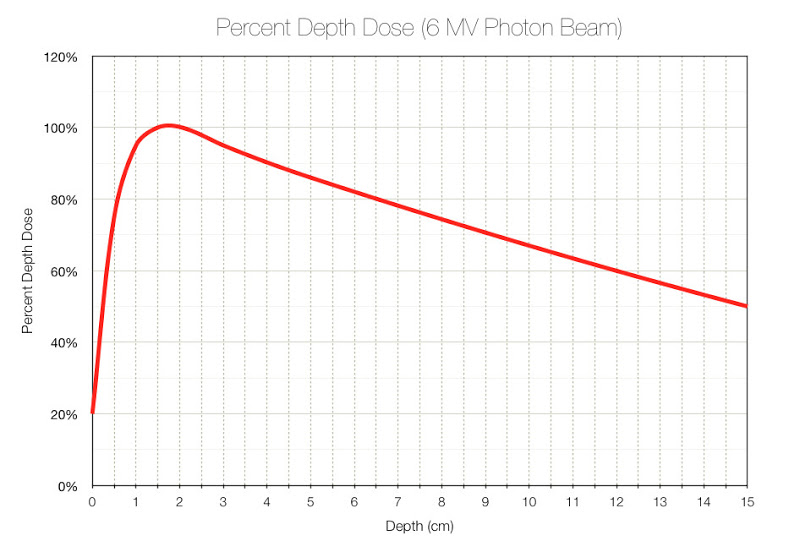
As can be seen from the above graph, the largest dose is deposited 1.5 cm beneath the skin of the patient. If you can imagine a radiation target smaller than this, increasing X-ray energy does not necessarily increase the dose, since higher energy X-rays can pass straight through the target, with very little energy being deposited in the target.
